I am a total noobi to solar power and even electricity so I apologize if I am asking a silly question.
Imagine I have a 10 watt light bulb which I want to leave on for 24 hours a day. Will I just need a solar panel which can generate 10 watts? Or will I maybe need a 20 watt solar panel, so 10 watts is used to power the 10 watt light bulb and the other 10 watts gets stored into a battery to power the light bulb during the night for 12 hours?
Assume the power input to the bulb is 10 Watts.
Assume for now 100% efficiency from battery output to bulb input.
Efficiency of energy storage by the battery of energy supplied to it will vary with battery chemistry and how well the charger is designed. Best case using a Lithium battery of some sort, over 90% efficiency may be achievable. Lower or much lower efficiency is often achieved in practice.
Efficiency of energy provided at the battery terminals compared with energy out of the PV panel will depend on the interface design and will also vary with battery state of charge.
Power output from the panel at any moment (Wp) and compared to the maximum power the panel can make under ideal conditions (Wmpp) will vary with insolation level (sunshine level), panel conditions, atmospheric conditions and more.
SO overall, a say 100 Watt panel will produce 100 Watts in full sunshine when new and will produce the equivalent of 2 or 3 hours of equivalent sunshine in most continental US locations in winter and 5 to 6 hours of equivalent full sunshine.
ie you get 200 to 700 Watt-hours per day depending on season.
With the very best interface equipment (MPPT, intelligent battery sizing to minimise resistive losses, ... you may get 95% + of this energy at the battery terminals and, as above, 90%+ of this actually stored into the battery.
So PV Watts rating x 0.95 x 0.9 x hours_equivalent_per_day = Watt-hours available. Say 85%. Using 80% would be safer and still very optimistic in many cases.
At the start I assumed 100% battery out to bulb in power.
Regardless of load type (which is usually LED in this context), if you want constant brightness as battery varies or constant "bulb" input there will be some conversion losses. 90% from battery to bulb or LED would usually be excellent.
So overall PV "nameplate rating" watt-hours to 'bulb' input watt-hours is at best about 75%. Usually less.
When the sun is providing energy, some gains can be had by running the bulb from the panel without battery storage. This gain is useful but still a small part of the total energy needed via the battery. I'll ignore it in the following and it can be factored in later if needed.
From the above:
Watt hours available = (Panel Watts rated) x 75% x Sunshine hours.
Watt hours wanted = Load_Watts x 24.
Rearranging the above -
Panel Watts needed = Load Watts x 24 / (0.75 x Sunshine hours )
= Load_Watts x 32 / Sunshine_Hours
So eg 10 Watt load in winter with 2 hours/day sunshine hours /day (= equivalent full sunshine).
Panel Watts needed = 10 x 32 / 2 = 160 Watts !!!
10 Watt load in Summer with 6 sunshine hours/day.
Panel watts needed = 10 x 32 / 6 = 53 Watts.
In practice higher Watts will be needed.
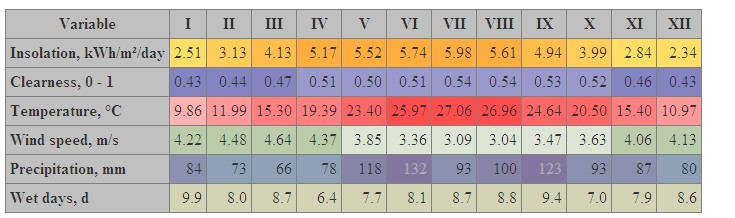
Averge sunshine hours per day can be found at the wonderful Gaisma site here - this example is for Houston
Top line is insoltaion in kWh/m^2/day = sunshine hours/day = hours of equivalent full sunshine. I = January, II = February etc.
2.34 hours/day in January.
5.98 hours/day in July
These are means for many years and any year and any day in the montyh may vary widely from this. That's weather for you :-)
More later ...
... in my comment ;o) Thnx. Interesting read.
\$\endgroup\$
You'll be unable to power a light bulb for 24 hours on solar panels alone. You need something to bank the energy generated by the panel so that it can be used when there is no sunlight. A battery pack is the most reasonable solution here.
The energy generated from the solar panel is dependent upon many factors such as your geographic location, orientation, angle, sun light hours, etc. Without more information it would be hard to size the solar panel, thought I can tell you a 10 watt solar panel will not suffice if you wish to run the 10 watt bulb indefinitely. The panel rating will need to be 3x (30W) or 4x (40W) the draw to be self sufficient.
As for the battery, you'll need to choose one with correct capacity and voltage. Your voltage will be determined by your lamp. For example, if you have a 12V lamp, you'll choose a 12V battery. Next, you'll need to calculate the capacity. Batteries are rated in A * hours. So, a 1Ah battery can supply 1 amp for an hour. According to your information, it sounds like night time in your location is approximately 12 hours. So, at minimum, the battery will have to survive without charge for 12 hours. First we determine the current as dictated by your chosen bulb (again, I am using 12V from my example but you'd substitute your voltage here):
P=V*I --> 10W/12V = .83A
Now that you know your current, you'd size you battery capacity by:
I*h --> .83A*12 hours = 10Ah
So, you'll need a minumum of a 12V, 10Ah battery to survive the night until your charging cycle can start again.
Not just any battery type will do either. You'll need a battery capable of accepting a charge. Examples of this would be lead-acid, NiMH, Li, etc. Controlling the charge is also another concern.
