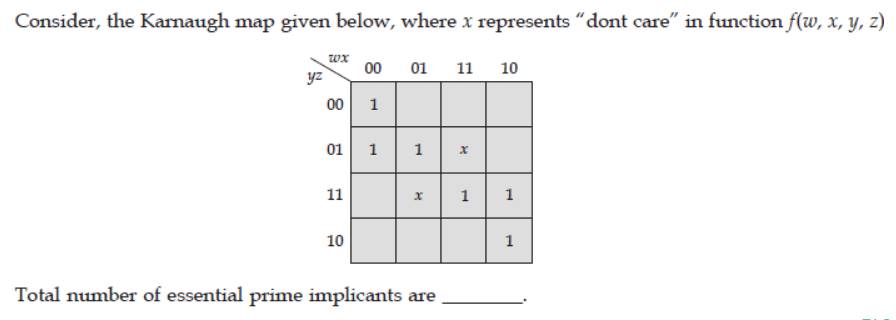
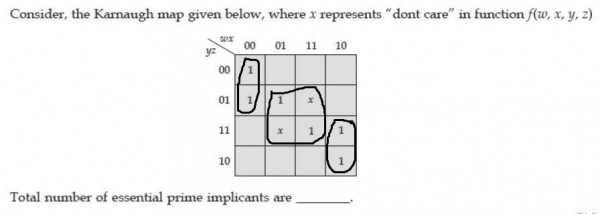
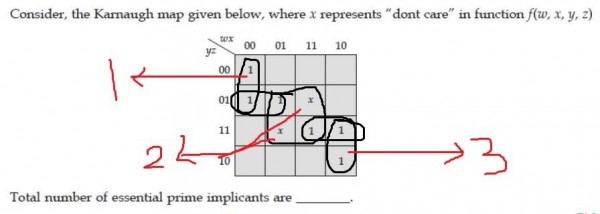
According to me, 3 is the number of Essential Prime Implicants.
If a don't care is used in getting minimal solution, then the group with that don't care can also be considered as EPI(provided it is grouped only once). Here, don't care must be included to form a quad. That quad is necessary to form Minimal Expression.
This is how minimal expression is obtained.
Thus we have 5 Prime Implicants and 3 Essential Prime Implicants.
So, Am I correct?
I answered a very similar question here: Essential Prime Implicant
A prime implicant is only essential if no other prime implicants can 'cover' its outputs... the only prime implicants that fit this definition are the ones in the corners. The ones in the middle can be covered both by the square and by the two horizontal rectangles. So the answer is two.
Only the 1s in xz are used for finding if a prime implicant is essential. Because we don't care if the other two are covered or not.
f(w,x,y,z) = w'x'y' + wx'y + xz is just as valid as f(w,x,y,z) = w'x'y' + wx'y + w'y'z + wyz
Since we can replace xz with w'y'z + wyz and get a different formula that does not simplify to xz, but all the results that aren't don't care still match, xz is not essential to this k map.
The other two are correct though, since only one implicant covers w'x'y'z' and only one covers wxyz, those are essential.
