Phase Voltage Confusion

Question

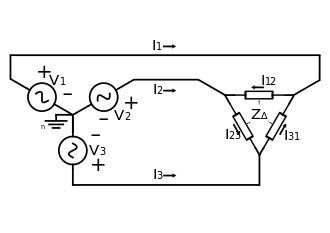
Here's a question that I was asked with the answer keys.

From the problem I deduced the net load impedance for one of the elements in the delta configuration will turn out to be 172.767|_3.66194 Ohms. Assuming that the voltage and current will be in rms we can use the equation. $$ \frac{V_l}{Z_p} = I_p $$

So given the phase voltage of 200V we can use this equation to solve for Ip

$$ \frac{(\sqrt{3}\angle{30^\circ})V_p}{Z_p} = \frac{\sqrt{3}*200\angle{30^\circ}V_{rms}}{172.767\angle{3.66194^\circ}} = 2.00507 \angle{26.3381^\circ}$$

This clearly doesn't match with the first answer but matches with the second. But why? I realized that the sqrt(3) factor is the corporate but removing it would change our equation to... $$ \frac{V_p}{Z_p} = I_p $$ Why is the phase voltage equal to the line voltage? I thought phase voltage was defined to be

$$ V_l = \sqrt{3}V_p $$

Which contradicts the equation they used above.

My other question is, is the phase voltage defined to be something else if we don't connect a 3 phase voltage source to it?

Answer 1

As you pointed out, the value you computed is one of the line currents, as you took the line voltage and divided by the load impedance.

The phase current will actually consist of the difference between two line currents. I believe this diagram can make it clear to you. Note that \$I_1 = I_{12} - I_{31}\$

Why is the phase voltage equal to the line voltage?

My other question is, is the phase voltage defined to be something else if we don't connect a 3 phase voltage source to it?

I agree this can be confusing.

A phase voltage is a voltage measured across any one component.

The diagram I presented you has a phase voltage for the source and a phase voltage for the load. The phase voltage for the load is actually the line voltage. In a \$\Delta\$ connection, the phase voltage is the same as the line voltage. That is not true for a Wye connection. At the diagram, the voltage across the top impedance \$Z\Delta\$ equals \$I_{12}Z\Delta\$, which is clearly the line voltage.

The phase voltage definition doesn't change, but the voltage you are gonna consider does. You can't just assume the phase voltage is always the voltage between phase to ground, as this is not true for a \$\Delta\$ connection. However, this does not depend on having a 3-phase source connected or not, it simply depends on the given connection.