Finding input impedance of the circuit

Question

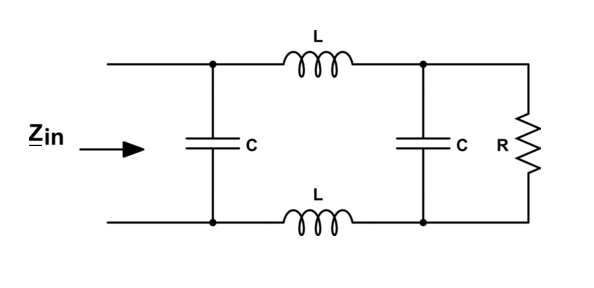
I'm having difficulties finding complex impedance of the following circuit:

What I did first is find the impedance of \$C\$ and \$R\$ in parallel, which is: $$\underline{Z}_{CR}=\frac{R}{1+j\omega CR}$$ Then, I added two inductor impedances: $$\underline{Z}_{CRL}=2j\omega L+ \frac{R}{1+j\omega CR}$$ and finally calculated the parallel of that with capacitor impedance \$\frac{1}{j\omega C}\$ which yields: $$\underline{Z}_{in}=\frac{\omega CR+2 \omega L(1+(\omega CR)^2)-j(1+2\omega CR^2)}{\omega CR + j(2\omega ^2 LC(1+(\omega CR)^2) -2(\omega CR)^2 -1)}$$ However, I'm not getting the correct result: $$\underline{Z}_{in_{correct}}=\frac{R(1-2LC \omega ^2) + j2L\omega}{1-2LC\omega ^2 +j2RC\omega (1-LC\omega ^2)}$$

Answer 1

\$\small R//C\$: $$\small \frac{R/sC}{R+1/sC}=\frac{R}{1+sCR}$$

Add series L's: $$\small \frac{R}{1+sCR}+2sL=\frac{R+2sL+2s^2RLC}{1+sCR} $$

Parallel C: $$\large \frac{\frac{R+2sL+2s^2RLC}{sC(1+SCR)}}{\frac{1}{sC}+\frac{R+2sL+2s^2RLC}{1+sCR}}$$

Clear denominators:

$$\small \frac{{R+2sL+2s^2RLC}}{1+sRC+sRC+2s^2LC+2s^3RLC^2} $$

Simplify: $$\small \frac{{R+2sL+2s^2RLC}}{1+2sRC+2s^2LC+2s^3RLC^2} $$

\$\small s \rightarrow j\omega\$:

$$\small \frac{{R(1-2\omega^2LC)+j2\omega L}}{(1-2\omega^2LC)+j2\omega RC(1-\omega^2LC)} $$

Answer 2

I think Chu missed a "2" factor in there somewhere. Here's my approach:

$$\begin{align*} Z_\text{IN}\left(s\right)&=\left(2sL + \frac{R}{1+sRC}\right)\,\biggl|\biggr|\:\frac{1}{sC}=\frac{\left(2sL + \frac{R}{1+sRC}\right)\cdot\frac{1}{sC}}{\left(2sL + \frac{R}{1+sRC}\right)+\frac{1}{sC}}\\\\ &=\frac{\left(2sL + \frac{R}{1+sRC}\right)\cdot\frac{1}{sC}}{\left(2sL + \frac{R}{1+sRC}\right)+\frac{1}{sC}}\cdot\left[\frac{sC}{sC}\right]=\frac{2sL + \frac{R}{1+sRC}}{\left(2sL + \frac{R}{1+sRC}\right)sC+1}\\\\ &=\frac{2sL + \frac{R}{1+sRC}}{\left(2sL + \frac{R}{1+sRC}\right)sC+1}\cdot \frac{1+sRC}{1+sRC}=\frac{R+s2L+s^22RLC}{1+sRC+sC\left(s^22RLC+2sL + R\right)}\\\\ &=\frac{R+2L\,s+2RLC\,s^2}{2RLC^2\,s^3+2LC\,s^2+2RC\,s+1}\\\\ \therefore\\\\ Z_\text{IN}\left(j\omega\right)&=\frac{R\left(1-2LC\,\omega^2\right)+j\,2L\,\omega}{\left(1-2LC\,\omega^2\right)+j\,2RC\,\omega\left(1-LC\,\omega^2\right)} \end{align*}$$