What actually is the differential gain of an operational amplifier and why does its value change when we consider the common-mode gain?

Question

Okay, this may be a stupid question, but at the beginning of my Applied Electronics course they taught us that the differential gain \$A_d\$ of an operational amplifier is ideally infinite; however, in real op amps, we get a limited bandwidth. Which is fine, because it's still very high. My problem raises when I was introduced to common-mode gain and differential-mode gains. In particular, I read that an amplifier subject to common-mode input signals has as a (non-ideal) output a linear combination of its differential mode input voltage mode and its common mode input voltage, i.e., $$A_dv_d + A_{cm}v_{cm}.$$ And for now, everything's fine. But let's consider the practical example in the picture below:

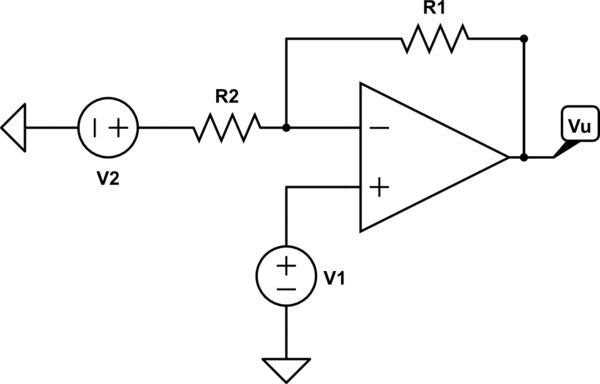
^{simulate this circuit – Schematic created using CircuitLab}

According to what I read, in the schematic above $$V_u=(1+\frac{R_1}{R_2})(v_+-v_-)=\frac{R_1+R_2}{R_2}V_1-\frac{R_1}{R_2}V_2.$$ And since \$\frac{V_1+\frac{R_1}{R_1+R_2}V_2}{2}=v_{cm}\$ and \$v_d=v_+-v_-\$, then \$V_1=v_{cm}+\frac{v_d}{2}\$ and \$V_2=v_{cm}-\frac{v_d}{2}\$. Moreover: $$V_u=\frac{R_1+R_2}{R_2}(v_{cm}+\frac{v_d}{2})-\frac{R_1}{R_2}(v_{cm}-\frac{v_d}{2})=v_{cm}(\frac{R_1+R_2}{R_2}-\frac{R_1}{R_2})+v_d(\frac{R_1+R_2}{2R_2}+\frac{R_1}{2R_2})=\Big(\frac{1}{2}+\frac{R_1}{R_2}\Big)v_d+v_{cm}=A_dv_d+A_{cm}v_{cm}.$$ How are we even just able to say that \$A_d=\frac{1}{2}+\frac{R_1}{R_2}\$? It's limited, fine, but I would've imagined bigger numbers. I guess I can live with a common gain of 1, but since they called both differential gains \$A_d\$, are they seriously the same?

Already from the beginning, I couldn't even understand why would we use $$V_u=(1+\frac{R_1}{R_2})v_d,$$since \$V_u=A_dv_d\$, with \$A_d\$ a big value and, instead, considering an ideal op-amp, as \$A_d\$ approaches infinity, \$V_u=\frac{1}{\beta}v_+=(1+\frac{R_1}{R_2})v_+.\$ But shouldn't \$(1+\frac{R_1}{R_2})\$ be the gain from the non inverting terminal to the output (i.e., closed loop gain), not of the op-amp, or am I wrong? What am I missing? Thank you in advance!

Edit: I am starting to assume that the answer is due to the principle of superposition of effect, i.e., shutting \$V_2\$ off and compute \$Vu\$ due to \$V_1\$, and then shut \$V_1\$ off and compute the overall gain due to \$V_2\$, and adding then the two results together. This could make sense, I suppose, but I can't still figure out why the result is equivalent to \$v_d\frac{R_1+R_2}{R_2}\$, since \$v_d\$ is supposed to be very small and it should therefore be amplified by a high gain \$A_d\$.

Edit 2: fixed typo that made the result wrong, but the question still holds.

Edit 3: fixed the value of \$\beta\$ in the formulas for this schematic. Unfortunately, I chose an unconventional position for \$R_1\$ and \$R_2\$ in the schematic.

Answer 1

$$\bbox[4px,border:1px solid red]{V_u=(1+\frac{R_2}{R_1})(v_+-v_-)}$$ The above assumption where you start from is actually wrong.

\$(v_+-v_-)\$ is infinitesimaly small or zero ideally.

The correct relation is: $$V_u = a_d.v_d + a_c.v_c$$ $$ie.,V_u = a_d.(v_+-v_-) + a_c.\frac{(v_+ +v_-)}{2}$$ $$\bbox[8px,border:1px solid black]{V_u = G_{OL}.(v_+-v_-)} $$ Where \$a_d\$ or \$G_{OL}\$ is the open-loop differential gain or simply open-loop gain, and it is very big or infinite for ideal op amp. And open-loop common-mode gain \$a_c = 0\$.

Coming to closed-loop gains in your circuit:

$$V_u=(\frac{R_1+R_2}{R_1})V_1-(\frac{R_2}{R_1})V_2 \tag1$$

The circuit has a negative feedback and you can calculate closed-loop differential and common-mode gains using the relations: $$V_1 = V_c+\frac{V_d}{2}$$ $$V_2 = V_c - \frac{V_d}{2}$$ where \$V_d\$ and \$V_c\$ are differential and common-mode components of \$V_1\$ and\$V_2\$:

$$V_d =V_1-V_2 $$ $$V_c = (V_1+V_2)/2 $$

Equation (1) can be simplified as: $$V_u=(\frac{R_1+R_2}{R_1}).(V_c+\frac{V_d}{2})-(\frac{R_2}{R_1}).(V_c - \frac{V_d}{2})$$ $$\implies V_u = (\frac{1}{2}+\frac{R_2}{R_1}).V_d+1.V_c \tag2$$ Compare (2) with: $$V_u = A_d.V_d+A_c.V_c$$ $$\bbox[8px,border:1px solid black]{\therefore A_d = (\frac{1}{2}+\frac{R_2}{R_1}), A_c = 1 }$$ where \$A_d\$ and \$A_c\$ are closed-loop differential and common-mode gains respectively.