I don't understand the solution to this problem. How can I write a ramp function in the form of a step function? 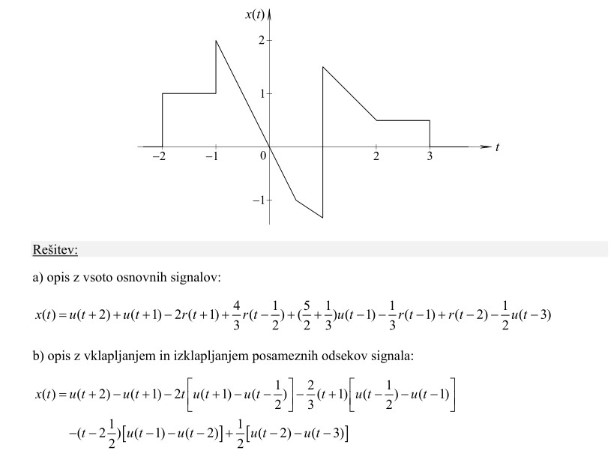
-
\$\begingroup\$ sorry, I don't understand the solution either – it's not written in a language I can read. Anyway, I think the formulas are quite clear. Could you please try to explain what confuses about these formulas? \$r\$ seems to be a ramp function. \$\endgroup\$– Marcus MüllerCommented Jan 8, 2020 at 15:10
3 Answers
How can I write a ramp function in the form of a step function?
You are not writing a ramp function in the form of a step function. The ramp function is t or to be more generic (t-a), where a is the value where the ramp function crosses the x-axis.
The step function u(t), when multiplied by the ramp function, determines the start of the combined function. For example, u(t-4)*(t-2) defines a function that is zero before t=4 due to u(t-4), and that crosses the x-axis again when t=2 due to (t-2).
So, in general, you could define r(t-b) = u(t-b)*(t-b) as your ramp function which is zero for t < b and goes up with slope of 1 for t > b.
The rectangular portions are made with step functions alone. The ramp portions include terms which are functions of t, giving them the slope.
You are piecing step and ramp functions together. You can choose when they start but they go on forever after they do and overlap so subsequent functions that start in the future must add to cancel out past functions that aren't needed anymore (of course you can also add to augment them, like changing from one step to a higher step or changing the slope of a ramp). Might be easiest in your mind to just completely cancel out all past functions before starting a new one each time and then simplify the terms to get rid of redundancies from all the needless cancelling.
r(t) is probably defined as a line with a slope of 1 crossing through (0,0) except that r(t) = 0 when t<0 (ie. starts when t=0). So multiply by a constant it to change the slope and add a constant to t to shift when it starts.
You can decide where on the Y-axis a ramp function starts by starting a step function at the same time.
