0
\$\begingroup\$

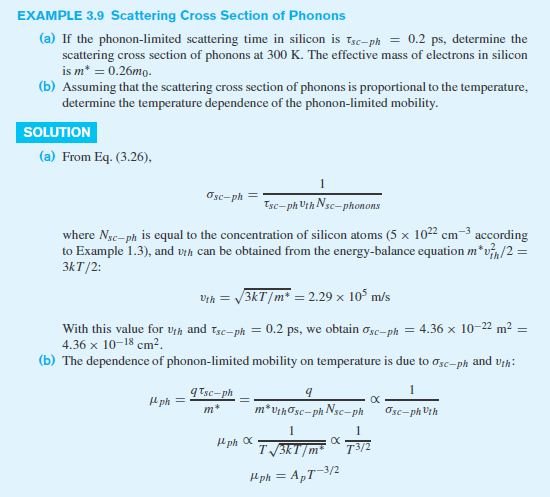
I'm working through Example 3.9 from Sima Dimitrijev's Principles of Semiconductor Devices textbook, and I'm not sure how to know which units to use for k in solving for \$v_{th}\$ in part a. The result there is obtainable using the Boltzmann constant k with units in J/K. So far in this textbook we've been using k with units in eV/K. I need insight into knowing which version of k to use, as both versions lead to a final answer with the same units.

Example 3.9 from Sima Dimitrijev's Principles of Semiconductor Devices

When I divide eV/J in Wolfram|Alpha I get a unitless number \$1.602 \times 10^{-19}\$, which I recognize as the value of electron charge with units in Coulombs. I feel like this is key to understanding my question but I'm not quite there. Any help is greatly appreciated!

\$\endgroup\$
12
  • \$\begingroup\$ See the Wiki page on the electron volt. \$\endgroup\$
    – jonk
    Commented Mar 7, 2021 at 16:34
  • \$\begingroup\$ If you're handling your units right, both ways will get you to the same answer. ...I hate how they don't teach how to properly handle units in schools. \$\endgroup\$
    – Hearth
    Commented Mar 7, 2021 at 16:34
  • 2
    \$\begingroup\$ @JakeNixon J and eV have the same dimension, not the same units. They are two different units. \$\endgroup\$
    – Hearth
    Commented Mar 7, 2021 at 16:40
  • 1
    \$\begingroup\$ @JakeNixon um, while they describe the same thing, an energy, they're not the same unit, so this is just a mistake by you, it seems? \$1\,\text{eV}\ne 1\,\text{J}\$! \$\endgroup\$
    – Marcus Müller
    Commented Mar 7, 2021 at 16:40
  • 1
    \$\begingroup\$ None "part" of the equation tells you that, you just need to write down what you're doing, and use the unit you get by using "your" choice of representation. I mean, this is really basic handling of units in calculations! \$\endgroup\$
    – Marcus Müller
    Commented Mar 7, 2021 at 16:51

1 Answer 1

Reset to default
2
\$\begingroup\$

As I said in the comments, I hate that they don't teach you how to properly handle units in schools...

You know how 1 kJ is 1000 J, or 1 foot is 12 inches? in the same way, 1 J is 6.242·10¹⁸ eV, and 1 eV is 1.602·10⁻¹⁹ (= 1/6.242·10¹⁸) J. The fact that you can convert one to another means they measure the same dimension, but the fact that they aren't equal, that that conversion factor isn't 1, says that they're different units.

\$\endgroup\$
5
  • \$\begingroup\$ This makes sense and helps me with terminology and understanding. I will admit that this was unintuitive as far as particle physics goes, but your analogy with feet and inches helped me very much. \$\endgroup\$
    – Jake Nixon
    Commented Mar 7, 2021 at 16:59
  • 1
    \$\begingroup\$ @JakeNixon I'm just really surprised this surprised you! I can't remember a time as student when I didn't use units to check whether my calculations made sense – which you can't do if this wasn't clear to you, so things must've been much harder on you (or you're just much smarter)! Either way, you should really get used to keeping the units around in all your calculations, and work a lot on paper; this becomes natural pretty quickly, I promise :) \$\endgroup\$
    – Marcus Müller
    Commented Mar 7, 2021 at 17:09
  • \$\begingroup\$ @MarcusMüller The reason that this was tricky to me is because sqrt(eV/kg) and sqrt(J/kg) can both be measured in meters per second at the end of the day. The issue lies in the fact that sqrt(1J/1kg) 1 m/s, whereas sqrt(1eV/1kg) is 4.003*10^-10 m/s. I was only looking at the final dimension. I've discussed with my classmates and this wasn't intuitive to anyone at this level. Perhaps it's something to blame on American education. \$\endgroup\$
    – Jake Nixon
    Commented Mar 7, 2021 at 17:27
  • \$\begingroup\$ yes, they can be measured in different units, but they are different units; if you treat the numbers and units separately, you'll notice that pretty quickly :) \$\endgroup\$
    – Marcus Müller
    Commented Mar 7, 2021 at 17:33
  • \$\begingroup\$ @JakeNixon As I said a few times, American education is really bad in this regard. Even into university I had teachers who didn't properly handle units and it got on my nerves every time. \$\endgroup\$
    – Hearth
    Commented Mar 7, 2021 at 18:03

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.