DC Power Supply - Constant Current and Voltage

Question

Can a DC power supply provide a constant voltage and constant current regardless of the load connected. For example 15V and 2A @ y ohms and 2y ohms.

Answer 1

Can a DC power supply provide a constant voltage and constant current regardless of the load connected.

No.

You can have constant current or constant voltage.

The condition for 15 V and 2 A is given by \$ R = \frac V I = \frac {15} 2 = 7.5 \ \Omega \$.

At twice that resistance (15 Ω) the current would fall to 1 A for a 15 V constant voltage supply.

At 15 Ω the voltage would rise to 30 V for a 2 A constant current supply.

---

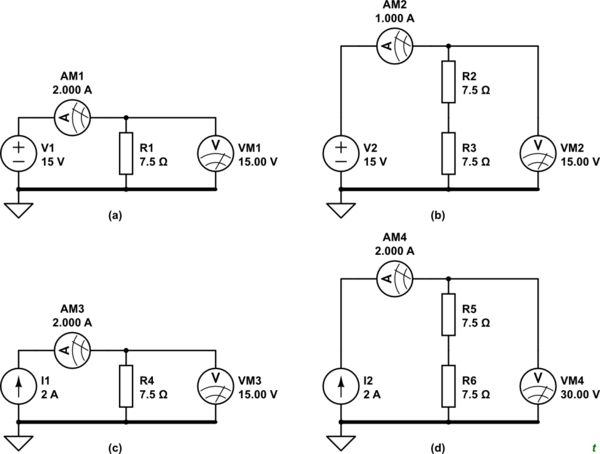
^{simulate this circuit – Schematic created using CircuitLab}

Figure 1. The various scenarios.

Answer 2

Short answer, no.

The problem with this question is that there is no such thing as constant voltage OR constant current. These exist only in the ideal world of circuits, in the real world such things dont exist. They are ok approximations, but that is all they are, and those approximations break down and become useless past a certain point. Those limitations even exist when talking about ideal circuits to an extent as well, lets dive in and see what I mean.

As we know from simple ohms law we have:

$$V = I \cdot R$$

So we know that if V and I are constant values then R must be a constant value was well.

$$R = \frac{V}{I}$$

So for there to be a C.V and C.C supply then the power supply would need to somehow control not just the voltage and current but also the resistance to ensure the equation remains satisfied. Consider the following circuit.

^{simulate this circuit – Schematic created using CircuitLab}

You might think the above circuit would do what your asking for by creatively adjusting Ri. Afterall if you want 10V constant voltage and 1A constant current you might think you can just set your voltage source V to 10V, then as long as RL is less than 10 ohms you can adjust Ri such that RL and Ri add up to 10 ohms, which ensures you would have a constant current of 1A right? Wrong, because the power supplies voltage would be measured at the point CC, not CV. So while CV might be able to stay at a constant voltage in that scenario the fact that Ri would be greater than 0 would mean that there would be some voltage dropped across it and CC therefore would be less than 10V.For example if RL were 5 ohms and Ri 5 ohms we would see only 5V at the output even though the internal voltage source is 10V. In fact all you really made in doing so is a really inefficient constant current source.

The reason i introduce it like this is because its not far off from how real world CC and CV power sources operate. The difference is that in a real powersource Ri is not a variable resistor, or even intentional. All power sources have a small unintended but impossible to avoid internal resistance, which is then hooked up to a variable voltage source. When operating in CC mode its still really a voltage source, not a current source, but its just smart and adjusts the voltage down or up until the expected current is reached. The catch, however, and why it isnt a true CC or CV is in that unintended Ri value, which forces it to only operate as a CC or CV within a certain range of values.

Here is a quick look at a closer model of how a real world adjustable power supply looks.

^{simulate this circuit}

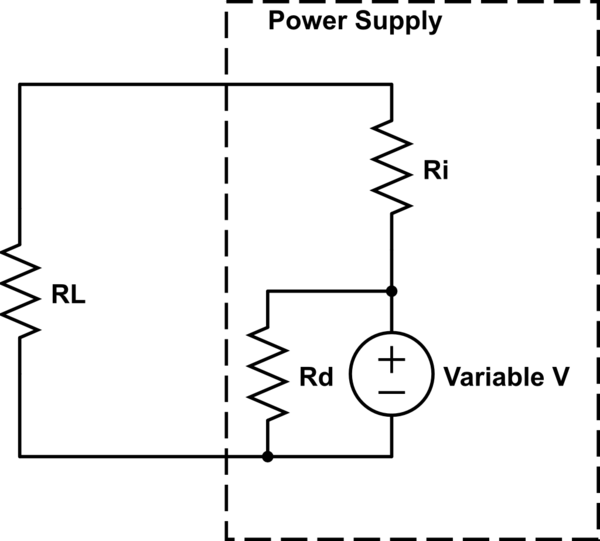
Notice we also added Rd, this represents any internal discharge in our voltage source, after all insulators are not perfect. While Ri is usually a very small value and always greater than 0 in the real world, likewise Rd is a very large value but finite in the real world.

In this case the variable voltage source, V, inside will either just be set to the desired voltage on constant voltage, in which case Ri is usually so small, and Rd so large, as to not be a concern, or, it is set in constant current mode in which case the voltage is automatically adjusted until the desired current is reached for any given load, changing as the load changes. But consider why this isnt a true CC or CV...

First, in CV mode Ri is going to present a small voltage drop, which means its true voltage is always somewhat less than its set voltage. This is only negligible when RL is considerably larger than Ri though, once RL is of similar or smaller value to Ri then the actual voltage will be much less than the set voltage. If RL and Ri are the same value, in fact, then the output voltage will be half the set voltage. If you short circuit the output with a 0 resistance wire then the output voltage will drop to 0 (all the voltage would be across Ri), not much for constant voltage huh? You could of course increase the voltage source to compensate for Ri, but since Ri is the dominant resistance now in the circuit that only goes so far. Afterall if RL is 0 ohms then no matter how high you can get V it wont cut it.

For this reason when we talk about ideal circuit models it would make no sense to short out a constant voltage voltage source at all.

The same problems crop up when talking about using it in CC mode. That is, as RL gets higher and higher the voltage would have to rise to maintain the same current. Too high and the voltage source cant keep up anymore. Rd in CC mode plays a similar limiting factor as Ri did in CV mode. That is once RL approaches a similar or larger value as Rd most of your current is going to go through Rd and be impossible for your voltage source to overcome and still deliver constant current.

As above where a CV source makes no sense when presented with a short a CC source makes no sense when handling an open circuit (which is why Ri and Rd exist in the real world, they make sense in the real world).

So in short, not only is it impossible to get CC and CV at the same time, its impossible to ever build a CV or a CC power supply at all. The best you can do is have a variable voltage supply that adjusts to approximate one or the other, and that approximation will only hold any meaningful value within a window of values.

PS: A battery (the simples CV approximation we have) is no different. the Ri I mentioned is why if you try to pull too much current from a battery its normally somewhat constant voltage will begin to drop. Even a battery isnt truly a CV device and has these limits.

Answer 3

A constant current source will have a compliance voltage (the greatest voltage difference it can provide -- usually rated at its maximum current if it is a variable current source.)

A constant voltage source will have a compliance current (the maximum current it can supply -- usually rated at its maximum voltage setting if it is a variable voltage source.)

The reason compliance is used is because the supply itself cannot determine both current and voltage, at once. If you were to set a constant current supply to \$1\:\text{A}\$ and placed a load of \$1\:\text{M}\Omega\$ at its terminals, then it would need to comply with \$1\:\text{MV}\$ in order to supply that current setting. And such current sources are... well... rather rare. Odds are that it can't comply with more than, say, \$36\:\text{V}\$, at best. So even with your desired current setting, the current source would simply fail to comply with the necessary voltage to achieve it.

So, No. You can't set both a fixed voltage and also a fixed current and then attach any load you want to it. That would only work with exactly one load resistance. And the odds of that are slim to none.

Things just don't work that way.