RL Transient Analysis confusion

Question

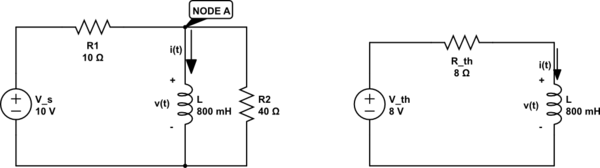
^{simulate this circuit – Schematic created using CircuitLab}

When I am using Thevenin's circuit and deriving inductor currents equations i am getting different Time constant \$ \tau\$ from what I am getting when I use Node Current analysis.

Here is my work for Thevenin's Circuit:-

\$from\ KVL :\$

\$V_{th} = i*R_{th} + L*\frac{di(t)}{dt}\$

\$\frac{V_{th}}{L}\ = i*\frac{R_{th}}{L}\ + \frac{di(t)}{dt}\$

Now after solving the homogeneous equation I get

\$i(t)= A*e^{-\frac{t}{\tau}\ \hspace{35pt} Where\: A\: is\: Constant}\$

\$ \tau = \frac{L}{R_{Th}}\ = \frac{0.8}{8}\ = 0.1s \$

This is what I am getting from Thevenin's equivalent circuit now Look at Original Circuit Equation

\$from\ KCL\: at\ node\: A :\$

\$ \frac{V_s-v}{R1}\ + \frac{0-v}{R2}\ - i = 0 \$

\$ \frac{V_s}{10}\ - v*(\ \frac{1}{10}\ +\frac{1}{40}\ ) - i =0 \$

\$ 8*v+i = \frac{V_s}{10}\ \$

\$ now\qquad v = L*\frac{di(t)}{dt}\ \$

\$ \frac{di(t)}{dt}\ + \frac{i}{8L}\ = \frac{V_s}{80L}\ \$

Now solving homogeneous equation

\$ \frac{di(t)}{dt}\ = - \frac{i}{8L}\ \$

\$i(t)= A*e^{-\frac{t}{\tau}\ \hspace{35pt} Where\: A\: is\: Constant}\$

\$ \tau = L*8 = 0.8*8 = 6.4s \$

Now here i am getting different \$ \tau \$ from what i get in Thevenin's circuit...also source is just constant. Please tell me where I'm making mistake.

Answer 1

Your first approach is correct. Second one, you took the KCL at node A (correct) but then two lines from there its V/8 but you wrote it V*8

PS: \$-V(\frac{1}{10} + \frac{1}{40}) = -\frac{V}{8} \$ ; not -V8!!!