What is wrong with this MOSFET differential pair analysis?

Question

I am confused regarding the analysis of the circuit on the left and its small-signal equivalent circuit on the right:

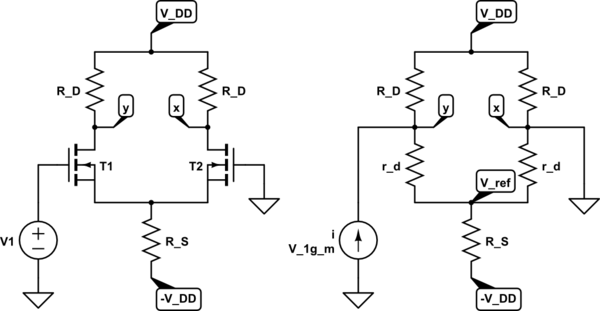
^{simulate this circuit – Schematic created using CircuitLab}

Here is my analysis:

Let \$i_1\$ and \$i_2\$ be the current through the parts above and below node \$y\$ respectively. Given that node \$x\$ is grounded, and assuming the reference \$V_{\text{ref}}\$ is at ground potential, we have:

$$i_1 = g_m \cdot \frac{r_d}{2R_D + r_d} \cdot V_1$$ $$i_2 = g_m \cdot \frac{2R_D}{2R_D + r_d} \cdot V_1$$ $$V_y = i_2r_d = g_m \cdot \frac{2R_Dr_d}{2R_D + r_d} \cdot V_1$$

However, \$V_y\$ is given as:

$$V_y = g_m \cdot \frac{R_Dr_d}{R_D + r_d} \cdot V_1$$

Where did I go wrong?

For clarity, \$i_1\$ is the current through the left \$R_D\$ and \$i_2\$ is the current through the left \$r_d\$.

Answer 1

Just because the gate of T2 is grounded doesn't mean that the drain is (AC) ground. Basically, as T1's current changes (from \$V_1\$), since T1 and T2 are coupled via their sources, T2's current also changes.

If \$R_s >> r_d\$, then the total current is constant, so the signal at \$x\$ is the inverse of the signal at \$y\$ (\$x == -y\$).

Your small signal circuit is not correct either. \$V_1g_m\$ is not from ground, but is across \$r_d\$.