Can someone help me derive the input and output resistances and the open circuit gain for a common base amplifier, using the pi model and T models? Please assume Va to be early voltage and no external resistor.
Circuit: 
Please note: I'm a newbie, and will really appreciate it if someone can either help me with the derivation or share resources for the same.
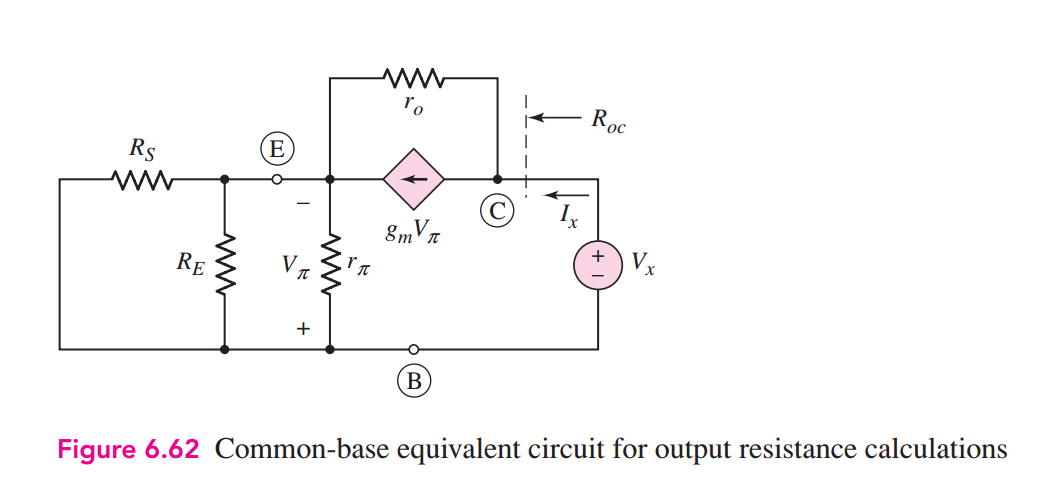
Well, we are trying to analyze the following circuit:
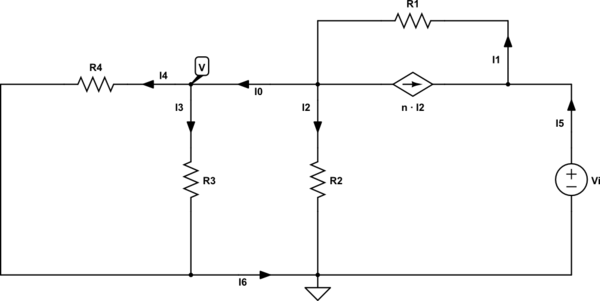
simulate this circuit – Schematic created using CircuitLab
When we use and apply KCL, we can write the following set of equations:
$$ \begin{cases} \begin{alignat*}{1} \text{I}_1&=\text{I}_5+\text{n}\cdot\text{I}_2\\ \\ \text{I}_1&=\text{I}_0+\text{I}_2+\text{n}\cdot\text{I}_2\\ \\ \text{I}_0&=\text{I}_3+\text{I}_4\\ \\ \text{I}_6&=\text{I}_3+\text{I}_4\\ \\ \text{I}_5&=\text{I}_2+\text{I}_6 \end{alignat*} \end{cases}\tag1 $$
When we use and apply Ohm's law, we can write the following set of equations:
$$ \begin{cases} \begin{alignat*}{1} \text{I}_1&=\frac{\text{V}_\text{i}-\text{V}}{\text{R}_1}\\ \\ \text{I}_2&=\frac{\text{V}}{\text{R}_2}\\ \\ \text{I}_3&=\frac{\text{V}}{\text{R}_3}\\ \\ \text{I}_4&=\frac{\text{V}}{\text{R}_4} \end{alignat*} \end{cases}\tag2 $$
Substitute \$(2)\$ into \$(1)\$, in order to get:
$$ \begin{cases} \begin{alignat*}{1} \frac{\text{V}_\text{i}-\text{V}}{\text{R}_1}&=\text{I}_5+\text{n}\cdot\frac{\text{V}}{\text{R}_2}\\ \\ \frac{\text{V}_\text{i}-\text{V}}{\text{R}_1}&=\text{I}_0+\frac{\text{V}}{\text{R}_2}+\text{n}\cdot\frac{\text{V}}{\text{R}_2}\\ \\ \text{I}_0&=\frac{\text{V}}{\text{R}_3}+\frac{\text{V}}{\text{R}_4}\\ \\ \text{I}_6&=\frac{\text{V}}{\text{R}_3}+\frac{\text{V}}{\text{R}_4}\\ \\ \text{I}_5&=\frac{\text{V}}{\text{R}_2}+\text{I}_6 \end{alignat*} \end{cases}\tag3 $$
Now, we can solve for the input resistance \$\displaystyle\text{R}_\text{i}:=\frac{\text{V}_\text{i}}{\text{I}_5}\$ as follows:
$$\text{R}_\text{i}:=\frac{\text{V}_\text{i}}{\text{I}_5}=\frac{\text{R}_1\text{R}_4\left(\text{R}_2+\text{R}_3\left(1+\text{n}\right)\right)+\text{R}_2\text{R}_3\left(\text{R}_1+\text{R}_4\right)}{\text{R}_2\left(\text{R}_3+\text{R}_4\right)+\text{R}_3\text{R}_4}\tag4$$
I used the following mathematica-code to solve this question:
In[1]:=Clear["Global`*"];
FullSimplify[
Solve[{I1 == I5 + n*I2, I1 == I0 + I2 + n*I2, I0 == I3 + I4,
I6 == I3 + I4, I5 == I2 + I6, I1 == (Vi - V)/R1, I2 == V/R2,
I3 == V/R3, I4 == V/R4}, {I0, I1, I2, I3, I4, I5, I6, V}]]
Out[1]={{I0 -> (R2 (R3 + R4) Vi)/(
R1 R2 R3 + R2 R3 R4 + R1 (R2 + R3 + n R3) R4),
I1 -> (((1 + n) R3 R4 + R2 (R3 + R4)) Vi)/(
R1 R2 R3 + R2 R3 R4 + R1 (R2 + R3 + n R3) R4),
I2 -> (R3 R4 Vi)/(R1 R2 R3 + R2 R3 R4 + R1 (R2 + R3 + n R3) R4),
I3 -> (R2 R4 Vi)/(R1 R2 R3 + R2 R3 R4 + R1 (R2 + R3 + n R3) R4),
I4 -> (R2 R3 Vi)/(R1 R2 R3 + R2 R3 R4 + R1 (R2 + R3 + n R3) R4),
I5 -> ((R3 R4 + R2 (R3 + R4)) Vi)/(
R1 R2 R3 + R2 R3 R4 + R1 (R2 + R3 + n R3) R4),
I6 -> (R2 (R3 + R4) Vi)/(
R1 R2 R3 + R2 R3 R4 + R1 (R2 + R3 + n R3) R4),
V -> (R2 R3 R4 Vi)/(R1 R2 R3 + R2 R3 R4 + R1 (R2 + R3 + n R3) R4)
