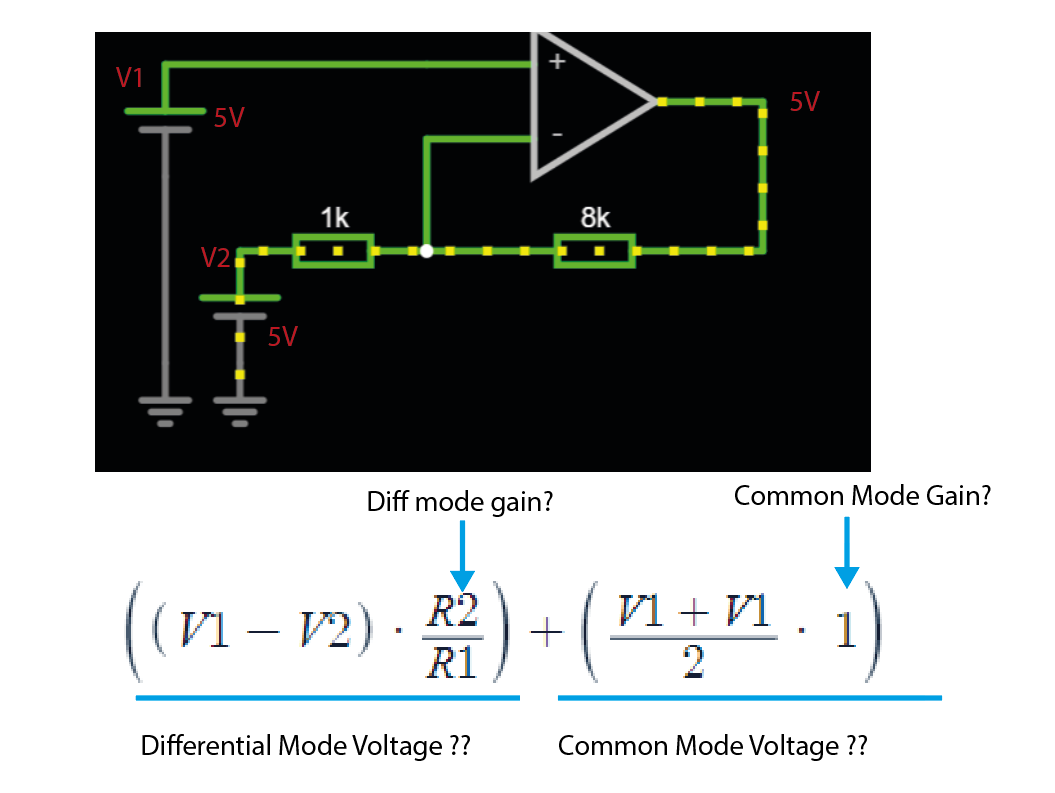
Non-inverting op-amp configuration multiplying common mode voltage by 1?
And can this equation be considered correct? While learning about op-amps, we made the assumption that common mode gain was considered zero. Does this only apply to difference amplifier configuration? If this equation is correct, is common mode noise included in the output in a non-inverting op-amp configuration?
The op-amp is doing exactly what it's supposed to do. You can apply superposition. Ground V1 and you have an inverting gain of 8 times 5 V or -40 V. Ground V2 and you have a non-inverting gain of 9 times 5 V or 45 V. Sum the two and you have your 5 V output. You don't have a differential amplifier configuration, you have an inverting and non-inverting summer.
Your equation is not correct, although it happens to yield the correct answer in this case.
The correct answer is Vout = V1 + (V1-V2)(R2/R1)
You can use superposition but setting the common mode voltage to zero does not simplify the analysis. You'd do better to just separate the two input voltages.
Common mode gain of the op-amp itself is (ideally) zero, but when you put it in a circuit you are measuring the voltages at different points. If you put a R2/R1 divider on the non-inverting input you will have a differential amplifier with (ideally) a common mode gain of zero.
Your transfer function is: Vo = (V1-V2)([R2/R1]+1)+V2, or specifically, 5V = (5V-5V)*([8]+1)+5V. The gain is 9 for V1 and the gain is 8 for V2. Ideally, the opamp has no common-mode gain; it cancels (rejects) signals common to both its inputs, hence what the CMRR (common-mode rejection ratio) specification tells you about the performance of real opamps. Like John D said, you don't have a difference amplifier configuration in your circuit if that's what you are trying to do.
