My circuit needs a s-curve type of pot, that is a W pot but i cant find it in a store.
How can i make a W pot Either using a log or a linear pot?
w pot graph
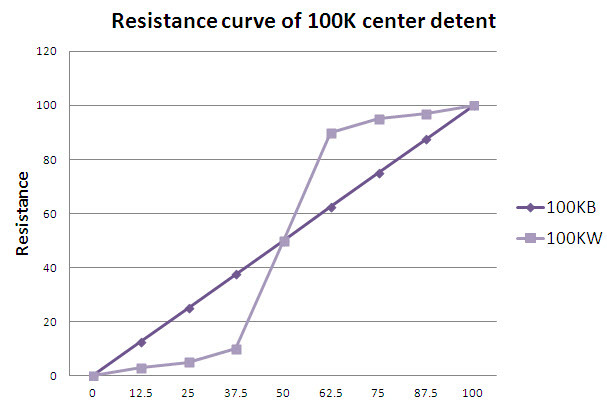
W pot=G pot Does anyone have a G pot graph?
\$\begingroup\$
\$\endgroup\$
3
-
\$\begingroup\$ What about using a transistor-based resistor? With 3 Op-amps, each one for each curve, complementing a s-curve. \$\endgroup\$– DorCommented Jul 14, 2013 at 21:12
-
1\$\begingroup\$ If this is for guitar, it is a waste of effort. You just need a scale around the pot to remember the knob positions. Put some equally spaced ticks around it and for the technically minded player you can put the actual calculated values next to these like kHz or dB or whatever. \$\endgroup\$– KazCommented Jul 15, 2013 at 4:32
-
1\$\begingroup\$ Tick mark label values are easy to calculate if a pot is linear. Just make sure the first and last tick line up accurately with the extreme rotations of the knob. Then linearly interpolate the resistance in between and plug into the circuit's formulas. \$\endgroup\$– KazCommented Jul 15, 2013 at 4:34
Add a comment
|
3 Answers
\$\begingroup\$
\$\endgroup\$
3
What are you going to do with the result? Things are mostly controlled digitally nowadays, which is why there is little point to non-linear pots anymore. Set up the pot to drive the A/D input of a microcontroller, then perform whatever non-linearities you want on the resulting linear reading.
Even in the unusual case where you really do want a non-linear analog voltage from the user setting, you can still use a cheap micro (under $.50) to read the pot, perform the non-linear function, produce PWM from than, then a R-C filter to make the average voltage level. The linear pot plus micro is often cheaper than the fancy low-volume non-linear pot.
answered Jul 14, 2013 at 17:07
-
\$\begingroup\$ The op is using various pots in a guitar effects foot pedal. \$\endgroup\$– Andy akaCommented Jul 14, 2013 at 19:05
-
1\$\begingroup\$ @Andy: If the point is to manipulate audio (which he hasn't actually said), then this is exactly the case I was talking about. Put a linear pot on the DSP and the rest is just math or a lookup table to get whatever non-linear function you want. This is no different from whatever other controls this device has. They are all just numbers the user can set by rotating knobs. The meaning of the numbers is up to the firmware. \$\endgroup\$ Commented Jul 14, 2013 at 19:08
-
\$\begingroup\$ Found out W pot is also known as graphic pot or G pot \$\endgroup\$ Commented Jul 19, 2013 at 5:54
\$\begingroup\$
\$\endgroup\$
It is possible using negative impedance convertor (http://en.wikipedia.org/wiki/Negative_impedance_converter).
Connect its ground point to midpoint of a pot, and output to a wiper. If Rnic = -Rpot/2, then curve is nearly perfect.
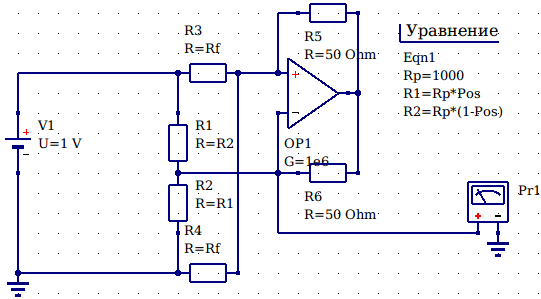
(Rf is -2*Rnic in this schema)
\$\begingroup\$
\$\endgroup\$
1
An s-curve pot is not simply achieved by adding resistors unfortunately. An inverse s-curve pot is easy to do. See this article right at the end for an example - it mistakingly calls it an "s-curve" but it means an "inverse-s curve".
Just so you are sure, please check if you need an s-curve or an inverse-s curve
This is the curve you get when you have a 100k pot with fixed resistors (100k and 50k) from both ends meeting at the wiper:
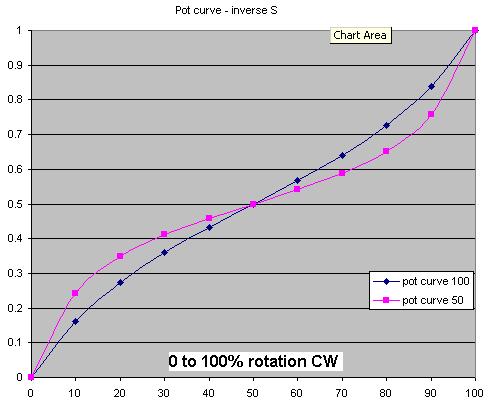
The vertical axis is \$ V_{out} / V_{in} \$ where \$ V_{in} \$ is applied across the pot and \$ V_{out} \$ is wiper to common connection of input
No matter what value of fixed resistors you choose the curve will be inverse S. Using a log pot won't help either - it'll still be an inverse S but biased towards the top of the graph.
answered Jul 14, 2013 at 16:31
-
\$\begingroup\$ i want a s-curve, or maybe close to a center dent pot. which becomes linear in middle. \$\endgroup\$ Commented Jul 14, 2013 at 16:52