I am trying to calculate the frequency response of the following circuit:
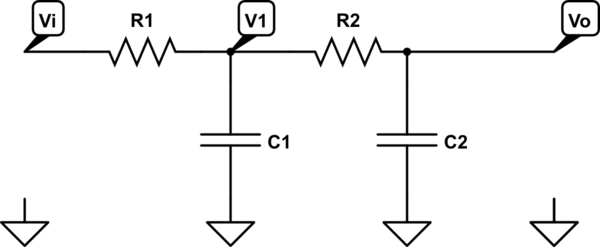
simulate this circuit – Schematic created using CircuitLab The two extra GND points are used to show that Vi and Vo are measured in relation to the ground.
Here are 2 methods for this:
Method 1
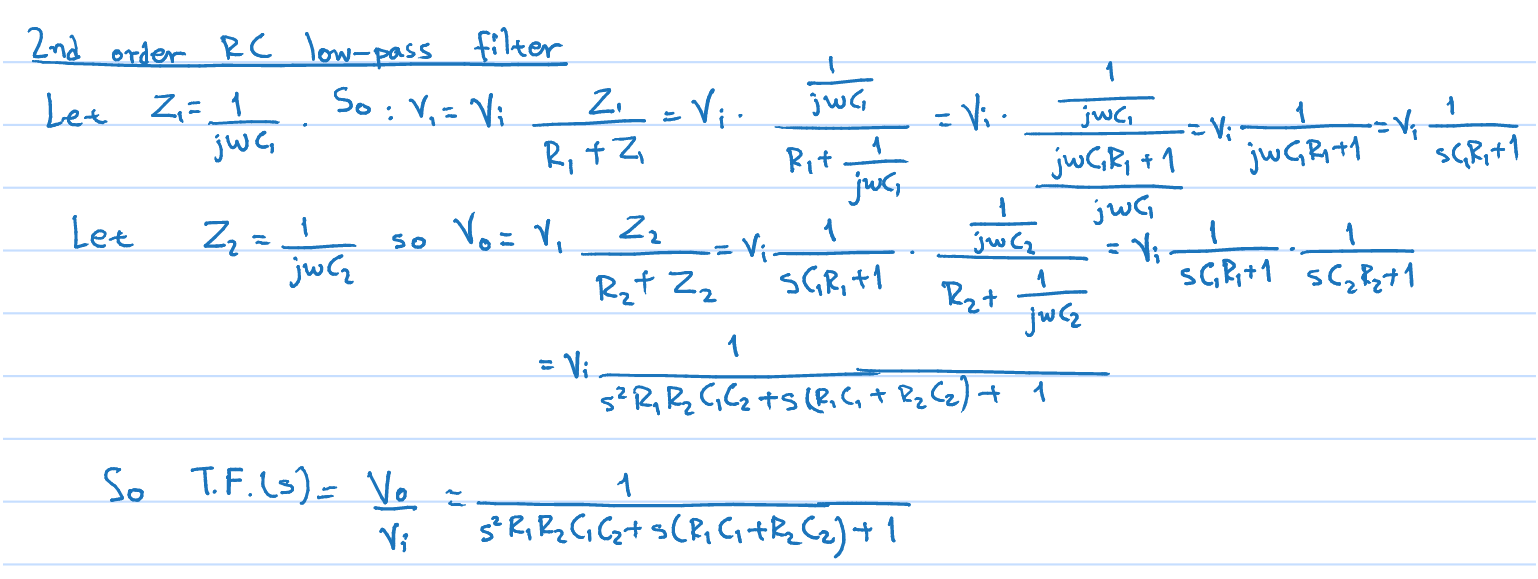
Method 2

The first method is the one I thought. As you can see, I used the formula of voltage divider two times. But the frequency response I found is different from the one in the 2nd method. At this point, let me clarify that the 2nd method supposes R1=R2=R and C1=C2=C but either way the frequency response is different. Basically, the 2nd method finds the same function as in here: http://sim.okawa-denshi.jp/en/CRCRkeisan.htm I suppose one of the methods is wrong, but why?
Sorry for not typing the equations but I thought this would be a waste of time. If something is not clear please ask me to clarify it.
