Circuit with switch - express the current for different times

Question

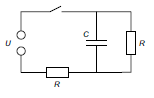
Consider the circuit below consisting out of a capacitor C and two identical resistors R. For \$t<0\$ the switch is open and the capacitor is uncharged. At \$t=0\$ switch is shut and the circuit is connected to the voltage source with constant voltage U.

a) What's the total current in the circuit immediately after the switch is shut? What's the charge of the capacitor and the total current after a very long time?

b) Determine for \$t>0\$ the total current in the circuit and the charge of the capacitor as a function of time by setting up a suitable differential equation and solving it.

I'm practicing for my exam in physics and I have always been weak concerning even the easiest circuits and I found this one in my textbook.

Here are my thoughts so far:

a) I suppose that in this type of circuit the current and the voltage are in phase, meaning that immediately after the switch is shut the current should be \$I=0\$?

But I don't seem to find an approach getting expressions for the current and the charge after a very long time. Can't I just assume that the capacitor will be fully charged, meaning \$Q=C\cdot U\$? About the current: Since at every part of the circuit there should be a different current, meaning at $R$ the current should be \$I_R=\frac{U}{R}\$ and I was thinking that since \$Q=C\cdot U\$ and the time derivative of \$Q\$ is \$I\$ and since \$U\$ is constant, does that mean that the current is \$0\$? Seems kind of unlikely.

b) I'm kind of lost here. I'm supposed to set up a differential equation which then would give me a function with which I can find \$Q\$ and \$I\$ at certain points?

I would appreciate any help. I seriously need to get better with circuits.

Answer 1

Copying the answer I gave when you posted the same question on Physics.SE:

I suppose that in this type of circuit the current and the voltage are in phase,

This question is asking for a transient solution, not an ac steady-state solution. So we can't really talk about the phase of the voltage or the current.

meaning that immediately after the switch is shut the current should be I=0?

This conclusion is incorrect.

Can't I just assume that the capacitor will be fully charged

No. It's explicitly stated in the problem, "For t<0 the switch is open and the capacitor is uncharged". The charge on a capacitor can not change instantaneously (when current is finite), so the charge on the capacitor is also 0 in the instant after the switch is closed.

A corollary that will help you solve this is that the voltage waveform across a capacitor is continuous.

I don't seem to find an approach getting expressions for the current and the charge after a very long time

The usual way to find the steady state dc response is to analyze the circuit in the dc regime, treating all capacitors as open circuits and all inductors as short circuits.

since U is constant, does that mean that the current is 0? Seems kind of unlikely.

No it's not unlikely. If the capacitor continued to accumulate charge after a long time, it's voltage would be increasing without bound, which would cause problems.

I'm supposed to set up a differential equation which then would give me a function with which I can find Q and I at certain points?

Start with

$$I_c = C\frac{\mathrm{d}V}{\mathrm{d}t}$$

and write \$I\$ in terms of other quantities you know (like the source voltage, the capacitor voltage, and the resistor values).

Answer 2

1) at t = 0, the cap is a short, therefore I = U / R

2) At very long time, because it is a DC source, the cap isn't see by the DC source. Then I = U / 2R

3) the easiest way to find the proper differential equation is to find the Thevenin equivalent seen by the capacitor. You will end up with the 'classical' RC circuit.

Regards MathieuL.