I want to implement a low pass filter in front of instrumentation amplifier as shown below:
In this this tutorial, it says "The overall differential-mode filter bandwidth should be at least 100 times the input signal bandwidth." But it doesn't explain why. What would be the effect if I have the cutoff frequency just above the signal bandwidth?
What would happen if you want to measure a 1 MHz signal but the bandwidth of your measurement amplifier is also 1 MHz ? At that frequency your input signal will be attenuated by 3 dB. This is because the definition of bandwidth is the frequency point where the attenuation is 3 dB. So your measured signal would be smaller than the actual signal at Vin.
Now what would happen if you want to measure a 1 MHz signal but the bandwidth of your measurement amplifier is much more, it is now 100 MHz. There would hardly be any attenuation of the signal.
The 100 times more bandwidth will result in a considerable margin regarding attenuation caused by a limited bandwith. If you do not require large accuracy then 10 times more bandwidth could suffice as well.
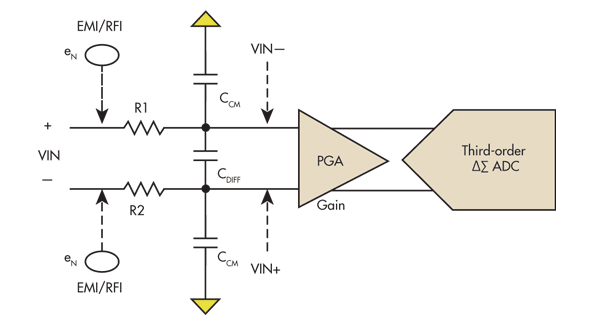
The article says: -
The overall DM filter bandwidth should be at least 100 times the input signal bandwidth.
And this is just plain rubbish in all practical designs. Sorry ADI but you are feeding people misleading information. If your base band extends to signals that are 1kHz a 1st order filter at 100 kHz will have an attenuation of 0.54\$\mu\$ dB at 1kHz. What is this in real numbers - 0.999999937.
At ten times the signal frequency, the little bit of attenuation is now 0.043 dB or 0.995 (real numbers) and this can be regarded as significant in some quarters. However, if you are going to be digitizing signals you might choose for an anti-alias filter to be 2nd order and this can actually restore the "droop" at 1kHz.
So, thank you to the questioner for pointing this out.
