1
\$\begingroup\$

I am quite new to electrical engineering.

I understand how to use Kirchhoff's circuit laws to get to my desired equations. What I don't understand though, is how to treat operational amps within those circuit laws. I can't find a helpful source which describes the process behind it. For example I would like to know how to get such equations for the following circuit. Where would the loops (Kirchhoffs second law) be in this circuit?

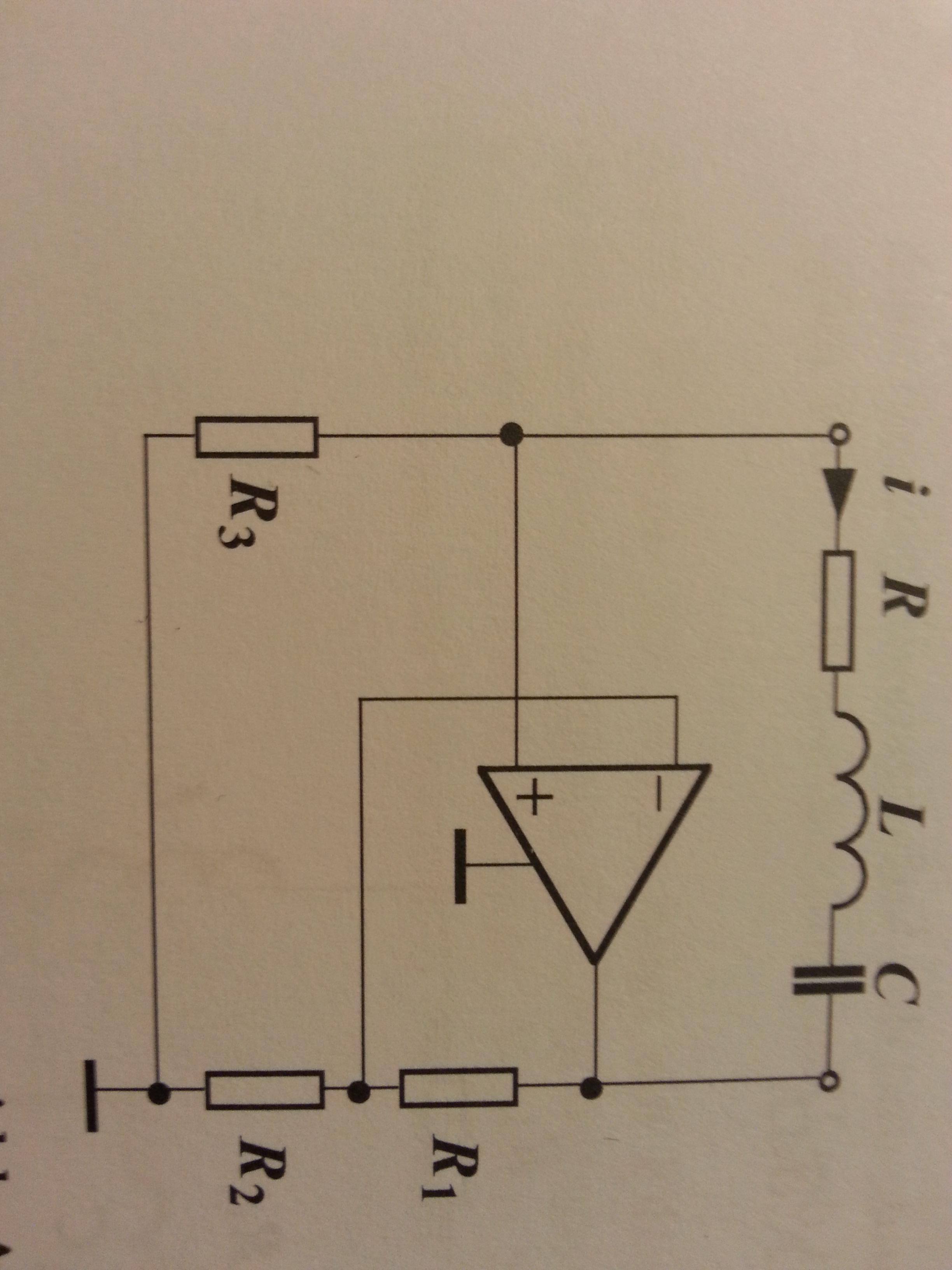
Example

\$\endgroup\$
4
  • \$\begingroup\$ Replace the op amp with a suitable model, like a voltage-controlled voltage source for example, and proceed from there. If you need to model frequency response add suitable RC pole, etc. \$\endgroup\$
    – John D
    Commented Dec 6, 2015 at 18:57
  • \$\begingroup\$ Understand the virtual earth \$\endgroup\$
    – Autistic
    Commented Dec 6, 2015 at 19:29
  • 2
    \$\begingroup\$ If you're modeling the op amp as an ideal op amp, then you can't apply KCL at the op amp output terminal, because you can't know how much current is flowing there -- all you can know is the voltage at the output node. With a negative feedback configuration, the op amp drives its output voltage to whatever is required to make its input terminals' voltages equal. (Or pretty nearly equal). So its output is modeled as an "ideal voltage source", powered by magic unlimited current, so KCL is left with an unknowable unknown. \$\endgroup\$
    – MarkU
    Commented Dec 7, 2015 at 1:06
  • \$\begingroup\$ There numerous free materials for this, e.g. op-amps for everyone or OCW/youtube lectures etc. In addition to what was said, you need to [preferably] solve his circuit in the s-domain because of the L and C. Come back when you have tried something. Also qsapecng can solve this; you can use it to check your work if this is homework or just get an answer otherwise. \$\endgroup\$
    – got trolled too much this week
    Commented Dec 8, 2015 at 3:41

0

Reset to default