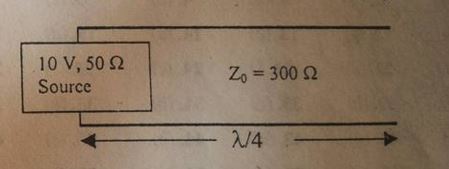Output Voltage of Quarter Wave transmission line

Question

Consider a 300 Ω lossless line operating at 1GHz which is connected to a source of 10V and impedance of 50 Ω.

What is the magnitude of voltage at the open circuit end?

My attempt:

At the input side the voltage (\$V_o\$) is 0V and the current at the sending end is given by:

$$I_o = \frac{10}{50} = 0.2A$$

and

$$V_o = 0V$$

Now the positive going voltage amplitude (\$V_+\$) is given by:

$$V_+ = 0.5 (V_o + I_o \cdot Z_o)$$

The negative going voltage amplitude (\$V_-\$) is given by:

$$V_- = 0.5 (V_o - I_o \cdot Z_o)$$

where \$Z_o\$ is the characteristic impedance.

Thus, \$V_+ = 30V\$ and \$V_- = -30V\$.

Now the voltage at any point on the line is given by:

$$V(z) = V_+^{-bz} + V_-^{+bz}$$

where \$bz\$ is the electrical length of the line.

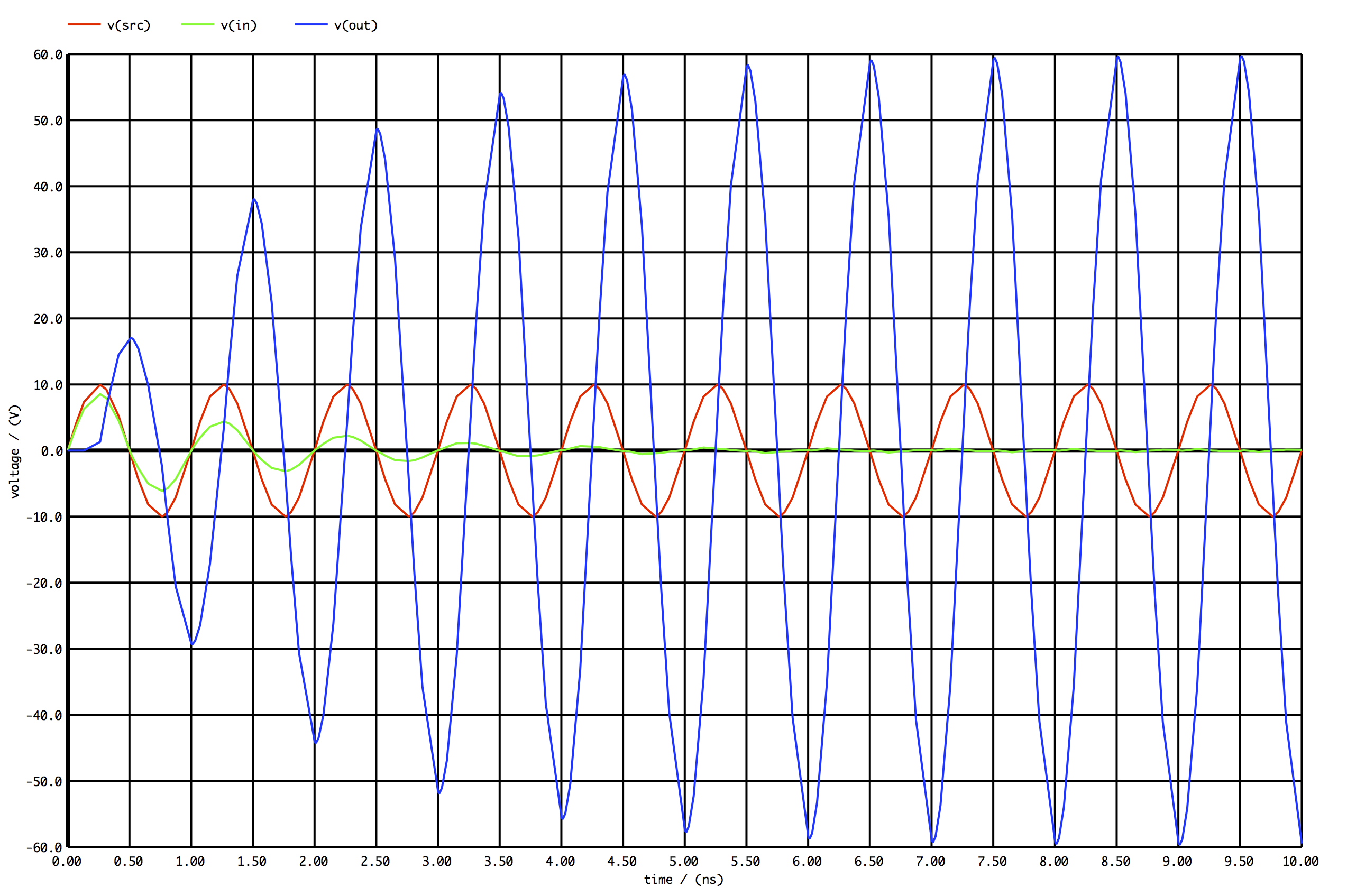
Thus at Load end \$V(\lambda/4) = j60 \cdot sin(bz) = j60\$

Is it possible to get a voltage of 60V at the open end while you are applying only a source of 10V? Does reflection at open end has anything to do with this?

Answer 1

Yes, 60v is what you would see at the end of the quarter-wavelength transmission line. This is due to the multiple reflections back and forth from the open at the end of the transmission line and the impedance mismatch at the input (300-ohm to 50-ohm). This effectively is creating a standing wave giving you 300/50 = 6 multiplication in voltage at the end of the quarter-wavelength and 0V at the input after a few cycles.

You can see the response below

Answer 2

γ = a + jβ a = 0 γ = jβ

Propagating wave

v = VI e-rz + VR erz

No change in the voltage at the open end it will same,

vr = 10 volt.