0
\$\begingroup\$

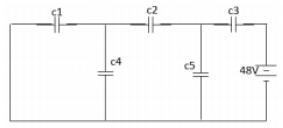
enter image description here

Each capacitor is \$24\text{nF}\$ and I need to find the voltage on \$C_\text2\$.

\$C_1+C_4=2*24=48nF\$

\$\frac{1}{C_\text{(1+4)||2}}=\frac{1}{C_\text{1+4}}+\frac{1}{C_2}=\frac{1}{16}\$

\$C_\text{(1+4)||2}=16nF\$

\$C_\text{(1+4)||2}+C_5=16+24nF=40nF\$

\$\frac{1}{C_{tot}}=\frac{1}{40}+\frac{1}{24}=\frac{1}{15}\$

So \$C_\text{tot}=15\text{nF}\$ \$Q_\text{tot}=C*V=15nF*48=720\text{nC}\$.

How should I continue to find the voltage on \$C_2\$?

Built the qeustion using CircuitLab how

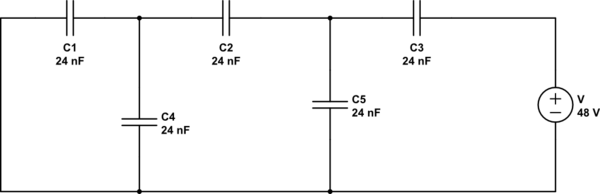
schematic

simulate this circuit – Schematic created using CircuitLab

\$\endgroup\$
2
  • 2
    \$\begingroup\$ Strong smell of homework. Show your maths. \$\endgroup\$
    – Transistor
    Commented Jan 25, 2016 at 16:59
  • \$\begingroup\$ @transistor I have nothing to hide it is homework, Sorry I have no posted my math, I am new here, so I will do it next time \$\endgroup\$
    – gbox
    Commented Jan 25, 2016 at 17:15

2 Answers 2

Reset to default
1
\$\begingroup\$

You've made an excellent start.

You have calculated the value of C1+4//2+5 = 40nF, and you know C3. This will allow you to calculate the potential at their common node, as whatever current flows through that node from C3 flows into the C1+4//2+5 effective capacitor.

Rinse and repeat for C1+4, and the value of C2.

This will give you the voltage at each end of C2, the difference is what you require.

Be aware that the learning intention of this exercise was not to calculate the voltage on C2 per se. It was a) to get you to recognise a ladder of impedances, b) to do as you did which was to cascade the impedances up from the bottom, until you find the effective impedance at the first node (40nF) c) from which you can get the voltage at that first node (18v) d) from which you can calculate back down to get the voltage a node at a time.

This is a ladder. The simplest non-trivial network of components.

\$\endgroup\$
8
  • \$\begingroup\$ I know that C3 has 720nF, so because all capacitors are the same I need to divided it twice for C5 and C2 and then again for C4 and C1? \$\endgroup\$
    – gbox
    Commented Jan 25, 2016 at 17:23
  • 1
    \$\begingroup\$ No, the whole network of capacitors is storing a charge of 720nC, which is distributed between them unequally. No, consider the potential divider formed by C3, and the composite of C1,2,4,5. That composite has capacitance 40nF, as you calcuated. When you raise the C3 terminal voltage to 48v, what voltage ends up on C3, and what voltage on the composite of C1,2,4,5? Hint, the same current flows through C3 as flows into the 40nF of C1,2,4,5, so they will have the same charge, Q=CV. You know the Cs, so you can calculate the Vs, knowing the Q is the same for both. \$\endgroup\$
    – Neil_UK
    Commented Jan 25, 2016 at 17:31
  • \$\begingroup\$ I get \$V_2=7.2V\$ \$\endgroup\$
    – gbox
    Commented Jan 25, 2016 at 18:41
  • 1
    \$\begingroup\$ Nope. Given that C3 is 24nF and C1,2,4,5 is 40nF, and they store the same charge, the ratio of capacitances is 24/40, so the ratio of voltages is 40/24. So the voltage at the common node is 24/64 * vin = 18v. Work through your sums until you can replicate that result. Then start with 18v on the common node, and repeat the voltage/charge calcuation for the divider ladder of C2 in series with C1//C4. \$\endgroup\$
    – Neil_UK
    Commented Jan 25, 2016 at 19:30
  • \$\begingroup\$ Can I build a smaller circuit with a voltage source of 18v? \$\endgroup\$
    – gbox
    Commented Jan 25, 2016 at 19:34
1
\$\begingroup\$

As you have done begin by calculating the equivalent capacitance of C1, C2, C4, and C5.

Ceq = 40nF

You know that the total voltage across C3 and Ceq must be 48V. This is because C3 and Ceq will have the same charging profile, and thusly the same amount of charge on both capacitors.

Qeq = Q3

and given that:

Q = Vcap * C

C3 * V3 = Ceq * Veq

V3 = 1.67 * Veq

Remembering:

48 + Veq + V3 = 0

we get:

48 + Veq + 1.67 Veq = 0

thus V3 = 30.1V and Veq = 17.9V.

From here, rinse and repeat for the remaining capacitors.

\$\endgroup\$
10
  • \$\begingroup\$ where did the 0.6 came from? \$\endgroup\$
    – gbox
    Commented Jan 25, 2016 at 17:59
  • 1
    \$\begingroup\$ @gbox I added the extra step to show how to calculate 0.6 and found I made a math error. . . how emberassing -_- . . . regardless the answer should show you a better thought process. Ceq/C3 = 40/24 = 1.66666666667 \$\endgroup\$
    – R. Johnson
    Commented Jan 25, 2016 at 18:10
  • 1
    \$\begingroup\$ using the equations you wrote: I get \$24*V_3=40(48-V_3)\$ and \$V_3=30\$ \$\endgroup\$
    – gbox
    Commented Jan 25, 2016 at 18:12
  • 1
    \$\begingroup\$ You are correct, I'm not claiming to be the best proof reader in the world. I double checked everything, should be correct now. \$\endgroup\$
    – R. Johnson
    Commented Jan 25, 2016 at 18:27
  • \$\begingroup\$ Sorry it is the first time I am answering this kind of qeustion. so next I look at \$C_5\$ and \$C_{1,4,2}\$ where \$C_5=24nF\$ and \$C_{1,4,2}=16nf\$ and \$Q_5=Q_{1,4,2}\$ \$\endgroup\$
    – gbox
    Commented Jan 25, 2016 at 18:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.