Finding impedance Z and \$ \cos (\alpha) \$ in RLC circuit

Question

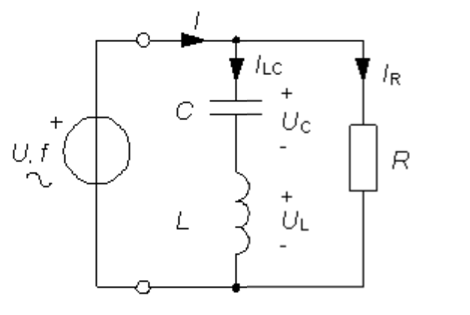
I would like to have some help with this problem:

U = 93 [V]

f = 600 [Hz]

L = 0,24 [H]

C = 0,16 [μF]

R = 950 [Ω]

This is the RCL circuit which I need to find the impedance \$Z\$ in and \$\cos(\alpha)\$

The first two questions that I had was to find the inductive reactance, XL, and the capacitive reactance, XC. I figured them out and I got XL = 905 ohm and XC = 1658 ohm.

I thought that I would use this formula:

$$\mathrm{impedance} \, Z = \sqrt{R^2+(X_L-X_C)^2}$$

But I can't get it right. I would also like to know if \$\cos(\alpha)\$ is solved through \$\cos(\alpha) = \frac{R}{Z}\$

Answer 1

If we consider this to be a circuit to have two parallel legs then the first is the series LC combination which has impedance \$ Z_1 = j \cdot (X_L - X_c) \$ , The second is \$ Z_2 = R \$

Now the simplest formula for two parallel paths is \$ Z = \frac{Z_1 \cdot Z_2}{Z_1 + Z_2} \$

Putting these together we have \$ Z = \frac{R \cdot j\cdot (X_L - X_C)}{R + j(X_L - X_C)}\$

Which is a little messy because we have \$ j = \sqrt{-1} \$ on both top and bottom of the fraction. Note in pure math \$ i \$ is used instead of \$ j \$ but in electronics \$ i \$ usually referrers to a current.

The best way to get rid of the imaginary part on the bottom is to multiply top and bottom by 1 in the form of the complex conjugate of the denominator.

So we have

\$ Z = \frac{R \cdot j\cdot (X_L - X_C)}{R + j(X_L - X_C)} \cdot \frac{R - j(X_L - X_C)}{R - j(X_L - X_C)}\$

I'll leave the rest as an exercise from here but assuming \$ \alpha \$ is the phase angle then \$ \alpha = atan\left( \frac{\text{imaginary_part}}{\text{real_part}}\right) \$ and

\$ |Z| = \sqrt{\text{real_part}^2 + \text{imaginary_part}^2}\$