Finding the Transfer Function and Simplifying it to Standard Form

Question

Hello, forgive my formatting- I am new and am trying to learn the ropes. When I tried to find H(s)=Vo/Vi, I derived:

[R2 || 1/(sC2)] / [(R1 || 1/(sC1) + (R2 || 1/(sC2)].

However, when trying to simplify the function into the form,

H(s) = K(1 + sTz)/(1 + sTp)

I am stumped. This might be as trivial as simple algebra, but perhaps I don't understand transfer functions that well. The closest I have gotten is:

1 / [((1/R2) + sC2) / ((1/R1) + sC1) + 1]

That was obtained from just dividing the top and bottom by the same expression 1 or 2 times.

I would like a hint or a push in the right direction. Thank you for your time.

Answer 1

It's actually nothing more than simple algebra. For this reason I usually use some kind of software to avoid mistakes.

In this case the result looks like this

You find the constant K by setting s to zero, which should be obvious from doing the same to the standard form.

To factor this expression out, you would have to divide the numerator by R2 and the denominator by R1 + R2.

Answer 2

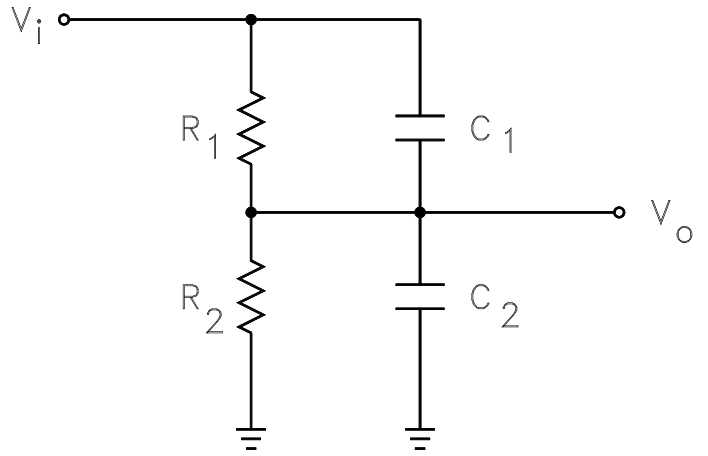
This is the typical example where the Fast Analytical Circuits Techniques (FACTs) will help you get the answer without writing a line of algebra. Look at your circuit for \$s=0\$: remove all caps. The dc transfer function is a simple resistive divider: \$H_0=\frac{R2}{R1+R2}\$. What is the time constant of this circuit? Reduce the excitation source (\$V_{in}\$) to 0 V and "look" at the resistance driving the capacitors. As you can see, both capacitors come in // forming a single cap. equal to \$C_1+C_2\$. This is what we designate as a degenerate case (1 single independent state variable despite the 2 energy-storage elements). The time constant is \$\tau=(R_1||R_2)(C_1+C_2)\$. So the pole is simply the inverse of \$\tau\$: \$\omega_p=\frac{1}{(R_1||R_2)(C_1+C_2)}\$. Is there a zero in this circuit? Yes, if the impedance \$Z\$ made of \$C_1\$ and \$R_1\$ becomes infinite at the zero frequency, the response disappears and this is your zero. What is the pole of this network (the value of \$s\$ for which \$Z\$ becomes infinite)? The time constant is \$R_1C_1\$, then the zero of this circuit is \$\frac{1}{R_1C_1}\$. The complete transfer function in a clean and ordered form is thus:

\$H(s)=H_0\frac{1+s/\omega_z}{1+s/\omega_p}\$ with

\$H_0=\frac{R2}{R1+R2}\$, \$\omega_p=\frac{1}{(R1||R2)(C1+C2)}\$ and \$\omega_z=\frac{1}{R_1C_1}\$

This where FACTs lead you to, no algebra, just inspection for these simple passive circuits.

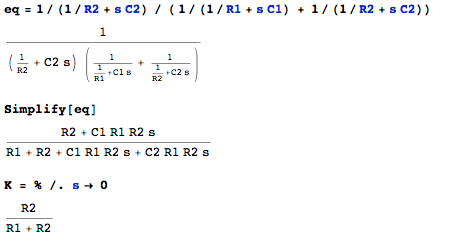
The answer given by the gentleman before is valid, but factor \$R_2\$ in the numerator \$R_2(1+sR_1C_1)\$, then \$s\$ in the denominator and then \$R_1+R_2\$. You obtain the same expression as in the above. This is a low-entropy form in which you see a dc gain (the leading term), a zero in the numerator and a pole in the denominator.

More details in the 2016 APEC presentation available here:

http://cbasso.pagesperso-orange.fr/Downloads/PPTs/Chris%20Basso%20APEC%20seminar%202016.pdf