In the given circuit, while finding Norton or Short circuit current, we short terminal A and B. So I have assumed that is(t) = short circuit current.
Is it correct to assume so?
I did it because as terminal A and B are short circuit, all the current is(t) has zero resistance path to ground. Is my analysis correct?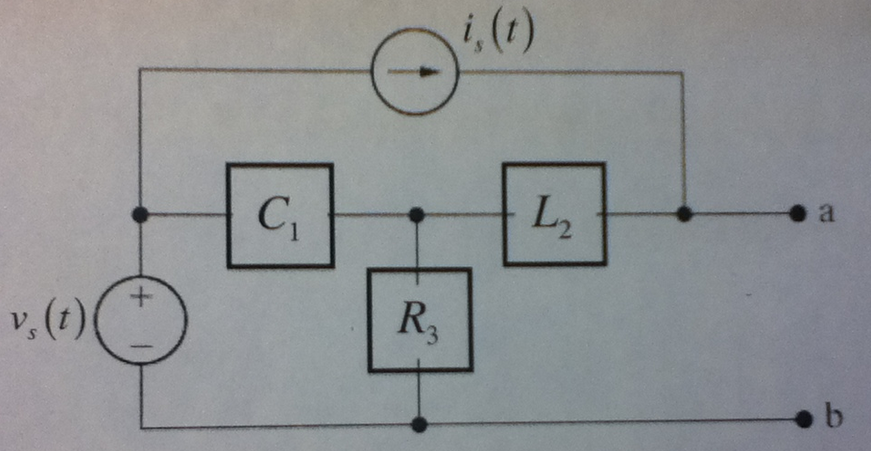
\$\begingroup\$
\$\endgroup\$
8
-
\$\begingroup\$ What about the voltage source? \$\endgroup\$– user110971Commented Nov 11, 2016 at 23:06
-
\$\begingroup\$ Yes, What about it? \$\endgroup\$– Ankit SahayCommented Nov 11, 2016 at 23:07
-
\$\begingroup\$ it also provides current. \$\endgroup\$– user110971Commented Nov 11, 2016 at 23:08
-
\$\begingroup\$ Yes, of course it does, but that results in current flowing through C1 and other elements. But the short circuit current through terminal AB will remain is(t). Is that not correct? \$\endgroup\$– Ankit SahayCommented Nov 11, 2016 at 23:10
-
\$\begingroup\$ draw the short circuit. What happens to L2? What about the current through L2? \$\endgroup\$– user110971Commented Nov 11, 2016 at 23:12
|
Show 3 more comments
1 Answer
\$\begingroup\$
\$\endgroup\$
We're using superposition to establish the short circuit curent. When ignoring \$i_1\$ we can calculate the impedance between terminals A and B:
$$ Z_{AB} = j \omega L_2 + \frac{1}{j \omega C + 1/R} $$
leading to a current of $$ i_{u1} = \frac{u_1}{Z_{AB}} = \frac{u_1}{j \omega L_2 + \frac{1}{j \omega C + 1/R}}$$
This is the first partial current. Now, if we consider \$i_1\$ and replace \$u_1\$ with a short circuit, \$i_1\$ is connected directly between terminals A and B. Thus:
$$ i_{short} = i_1 + i_{u1} $$
So you are half correct. The short circuit current consists of a part \$i_1\$ and a part \$i_{u1}\$ contributed by the voltage source.
