Address decoding

Question

I'm currently working on a task where I'm supposed to find the memory address of the following external bus interface of a microcontroller (logical 0, CS-signal)

However, I am uncertain of how to solve the issue. I managed to find the the memory address of ROM and RAM 2.

ROM (HEX): 0000 - 00FF (0000 0000 xxxx xxxx)

RAM 2 (HEX): 8000 - FFFF (1xxx xxxx xxxx xxxx)

Here's my idea of RAM 1: 8000 - FFFF (1xxx xxxx xxxx xxxx)

RAM 1 and 2 can't have the same memory address obviously, so what am I doing wrong? What is the memory address of RAM 1?

Thanks in advance!

Answer 1

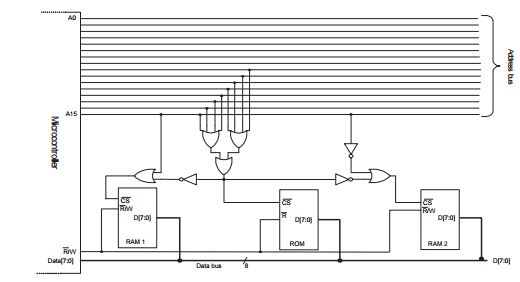
Let's start by labelling some bits of the diagram for easy reference:

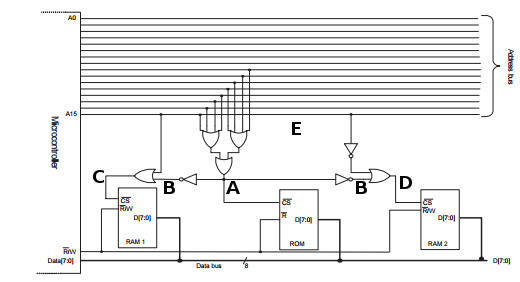
Now we can define what the different labels mean.

• A is HIGH if any of A8-A15 are HIGH.
• B is NOT A
• C is HIGH if either B or E (which is A15) is HIGH
• D is HIGH if B is HIGH and E is NOT HIGH.
• E (as I mentioned) is A15.

So we can make some boolean expressions now:

• A = A8+A9+A10+A11+A12+A13+A14+A15
• B = ¬A
• C = B+E
• D = B+¬E

Expanded:

• C = ¬A+E
• D = ¬A+¬E

Since CS is always active LOW you are interested in the FALSE values of those expressions - the values that make the expression result in an answer of FALSE.

So if any of A8-A15 are true then A must be true, ergo to get A to be false NONE of A8 to A15 can be HIGH. So A will be LOW for address range 0b00000000XXXXXXXX (or 0x0000 - 0x00FF)

If either B or E are true then C is true. Therefore B and E both have to be false for C to be false. E is A15, so we know now that A15 must be LOW to ensure that C can be false. A has to be true since it is negated to form B. So one of A8-A14 must be high to make A true and thus B false.

That leads us to the address 0b0YYYYYYYXXXXXXXX where at least one of Y must be high and we don't care about X. That is, anywhere between 0b00000001XXXXXXXX and 0b01111111XXXXXXX, or in hex: 0x0100 to 0x7FFF.

D is the same as C except for the fact that E is inverted. That means that A15 must be HIGH to make D able to be false. Since A15 is high we have the ability to set A8-A14 low and still be valid for this range. So we get 0b1YYYYYYYXXXXXXX, or 0b10000000XXXXXXXX to 0b11111111XXXXXXXX, or in hex: 0x8000 to 0xFFFF.

To put those address ranges in context let's build a full memory map:

Answer 2

You have a ·CS signal line in there. And you've got a "not" gate in between A15 and RAM2's chip select line, that isn't there between A15 and RAM1's CS. So, at any given time, only one RAM chip is "listening". There, thus, is no address collission, and the A15 bit selects the RAM chip.