How can I find the equation of current in the following circuit, when switch is closed at t=0. Capacitor is initially not charged.
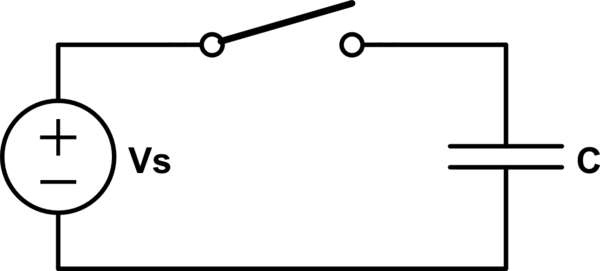
simulate this circuit – Schematic created using CircuitLab
How can I find the equation of current in the following circuit, when switch is closed at t=0. Capacitor is initially not charged.

simulate this circuit – Schematic created using CircuitLab
There is no equation.
With an ideal voltage source and ideal switch, wires and capacitor, infinite current is taken for an infinitesimal length of time to charge the capacitor to Vs instantly.
Your schematic makes only sense if theres a series resistor between the capacitor and the power source.
In this case the voltage and current waveforms for charging the capacitor are:
\$ V_{cap}(t) = V_s \cdot \left( 1 - e^{-t/\tau}\right) \$ and
\$ I_{cap}(t) = C \cdot \frac{dV_{cap}(t)}{dt} = C \cdot V_s \cdot \frac{1}{\tau}\cdot e^{-t/\tau} = V_s \cdot \frac{1}{R}\cdot e^{-t/\tau}\$
where \$ \tau = R \cdot C\$
It all results from the equation: \$ I(t) = C\cdot \frac{dV(t)}{dt}\$. This is the characteristic eqaution for a capacitor.
If you have a series resistance in your schematic and use the KVL You get:
\$ V_s = V_R(t) + V_{cap}(t)\$.
With
\$ U_R(t) = R \cdot I_{cap}(t) = R \cdot C \cdot \frac{dV_{cap}(t)}{dt}\$
you get:
\$ V_s = R \cdot C \cdot \frac{dV_{cap}(t)}{dt} + V_{cap}(t) \$
you can solve this differential equation using different methods, for example using Laplace Transform.
The solution is the equation above.
The circuit without resistance doesn't produce a waveform. If you close the switch, the voltage change on the cap would be infinitely high which results in an infinite current. The capacitor is charged to its full voltage instantly.