If a capacitive load is connected at the secondary coil of a transformer, a leading current flows and in turn, the secondary voltage is higher than the referred primary voltage. I learned that partial resonance is the reason behind this. However, I can't seem to understand why.
\$\begingroup\$
\$\endgroup\$
2
-
\$\begingroup\$ depends on the type of transformer and relative magnitudes. Do you have a source that describes this for a particular transformer, if so, post it. \$\endgroup\$– Neil_UKCommented Mar 1, 2017 at 7:58
-
\$\begingroup\$ During the first half of the cycle the capacitor will charge a little. Toward the end of the first half of the cycle once the voltage across the coil has dropped below the voltage it charged the capacitor to the current in the secondary will reverse before the primary current does. When the primary current reverses in the second half of the cycle there is already a current flowing in the secondary, so it reaches a much higher peak current since it is being driven by both the primary coil and the capacitor. \$\endgroup\$– TWizCommented Mar 1, 2017 at 9:50
Add a comment
|
1 Answer
\$\begingroup\$
\$\endgroup\$
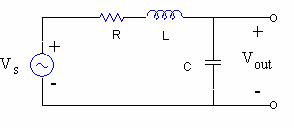
It usually boils down to leakage inductance. Leakage inductance is in series with each winding and it's that inductance (usually small) that doesn't couple either primary to secondary or secondary to primary. In a normal power transformer, the problem of leakage inductance manifests as: -
- Vout/Vin not exactly agreeing with what the turns ratio suggests
- The above problem getting worse under load conditions
So, it's a series component and can resonate with an output capacitor and here's a simplified example: -
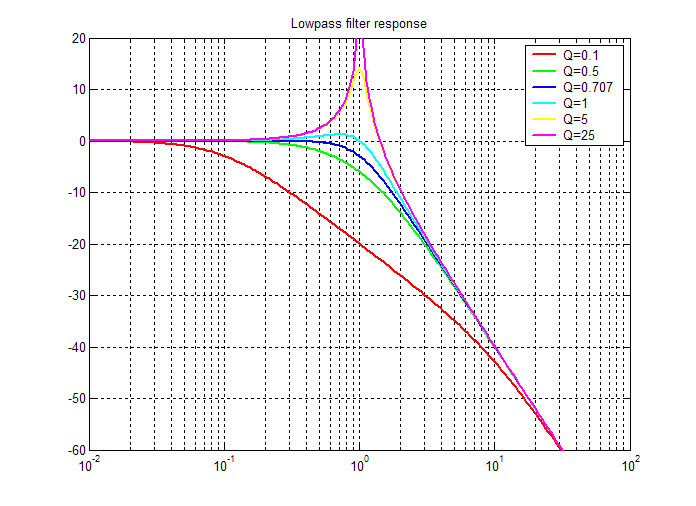
L is leakage inductance and C is the capacitor you loaded the secondary with. R is the equivalent copper losses. Depending on several factors you may get a very big resonance peak. Examples vary like this: -
If losses are very small, many dBs of amplification can be achieved. The graph above is normalized at 1 Hz just for convenience. Q is the quality factor of the leakage inductance and depends on resistive losses and can also be influenced by core losses.
