Op-amps, their impedance and current flow

Question

I'm an undergratuate student and I have a question about op-amps.

As I understand it you need infinite input impedance so there is voltage drop across the op-amp and not the signal device. However, doesn't infinite resistance means that no current will flow through the op-amp? Do you get any form of current at the output?

Thank you for taking your time to read this.

Answer 1

Infinite input impedance means that no current flows into the input terminals of an ideal op amp. The ideal op amp also has zero output impedance, and most certainly provides current.

The image above shows a non ideal op amp in an inverting configuration. To idealize this, \$Z_{in1}\$ and \$Z_{in2}\$ are equal to \$\infty\$, and \$Z_{out}=0\$, making \$ e_{out}=v_{out}\$. To finish off the ideal assumptions, \$A_{OL}\$ is the open loop gain of the op amp, and is equal to \$\infty\$

Answer 2

An OpAmp can be considered a voltage-controlled voltage source. You apply a voltage at both inputs, the OpAmp 'measures' the differential voltage \$v_D\$ and applies a voltage proportional to \$v_D\$ at the output. The proportionality is determined by the open loop gain of the OpAmp. It is usually very high, about 1E5, infinitely high for an ideal OpAmp.

The energy to drive the output comes from the supply rails, not from the input. This is the trick with an ideal OpAmp: It has inputs where no current flows in (aka high impedance input), but a voltage appears at the output that can supply some current (aka low impedance output). So it is an impedance converter.

As the open loop gain of an OpAmp is so high, you usually do not use it in this configuration for amplifier designs. Instead, you apply negative feedback (output somehow connected to inverting input). If there is negative feedback, you can make another important assumption of an ideal OpAmp: \$v_D\$ is zero. This means, the OpAmp will drive the output to whatever value is needed to achieve \$v_D=0\$. Additionally, still no current is flowing into the OpAmp.

So, the inputs of an ideal OpAmp with negative feedback employed show no current inflow and no voltage across them and are therefore neither a short (no voltage, maximum current) nor an open loop (maximum voltage, no current).

Keep in mind, that applying negative feedback inserts a connection from input side to output side and therefore influences input and output impedance. So the OpAmp is not anymore the near-to-ideal impedance converter mentioned above. Instead you have to analyze input and output impedance depending on the feedback circuitry.

Answer 3

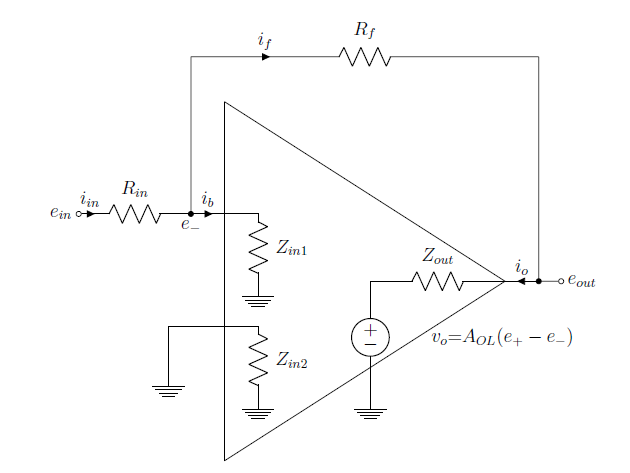
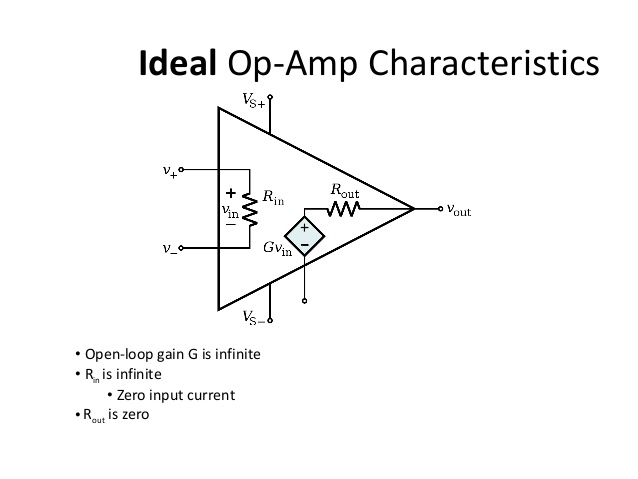
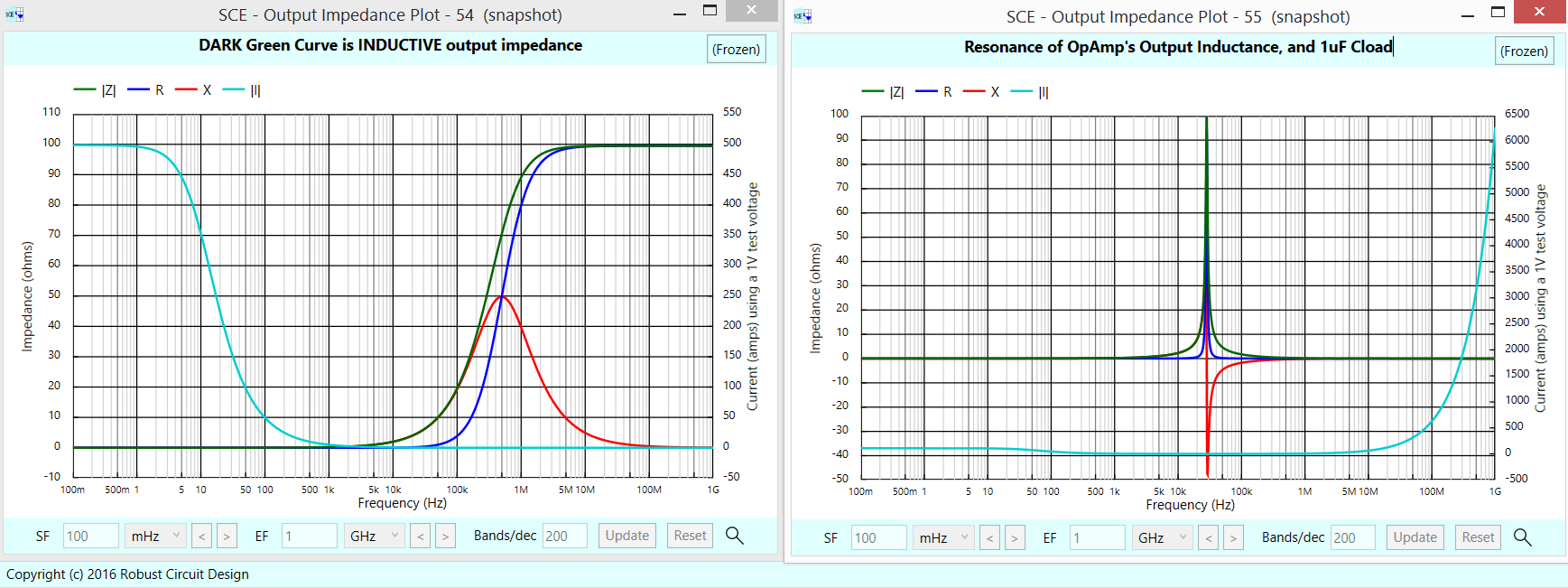
The output current of an OpAmp provides surprises. Over much of the useful range of frequencies, there is a 90 degree phaseshift between Vin (the difference between Vin+ and VIn-) and the Vout. You can see this phaseshift, in the left plot shown below. 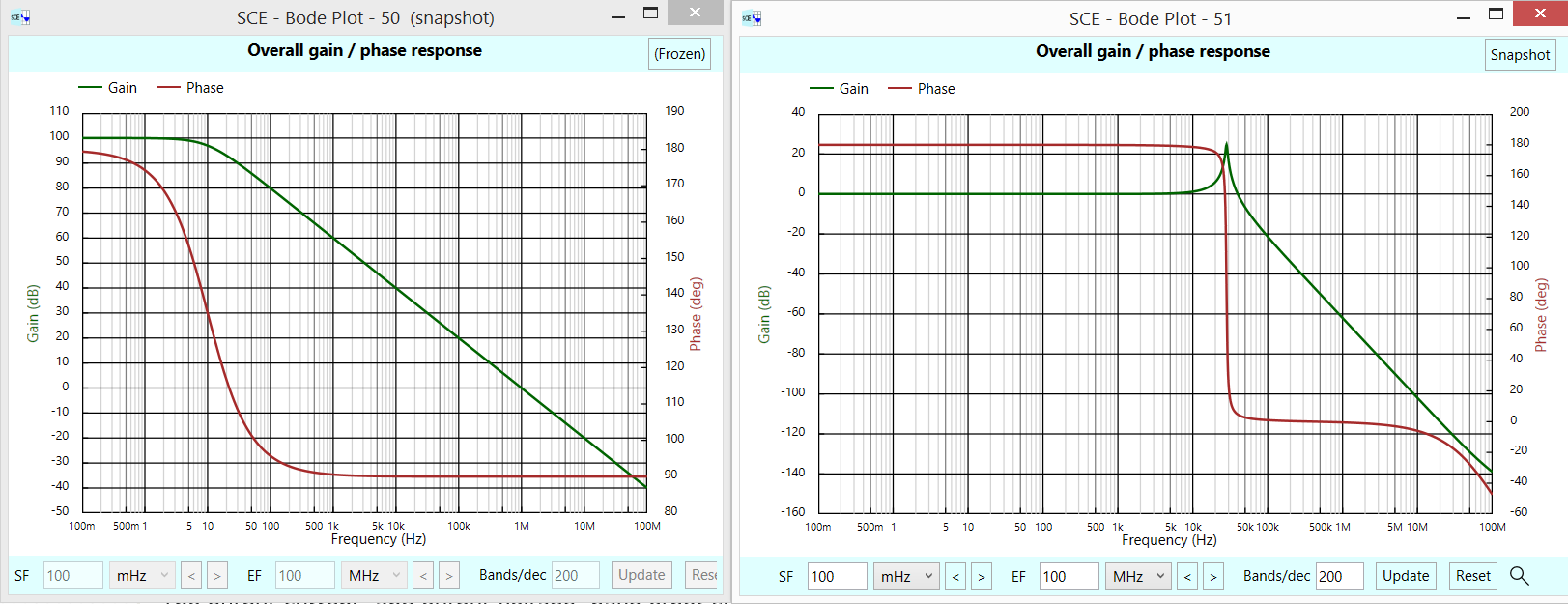What does this phaseshift do? It makes the Vout appear inductive, particularly with the output current dropping with frequency.

Add on a capacitor, and you get another surprise: peaking of frequency response, shown above in the right plot.

In this next set of plots, we see the OpAmps INDUCTIVE Zout on left (looking back into the OpAmp stage); then we look back into the Cload stage and see the combined effect with the sharp resonance of Lout and Cload.

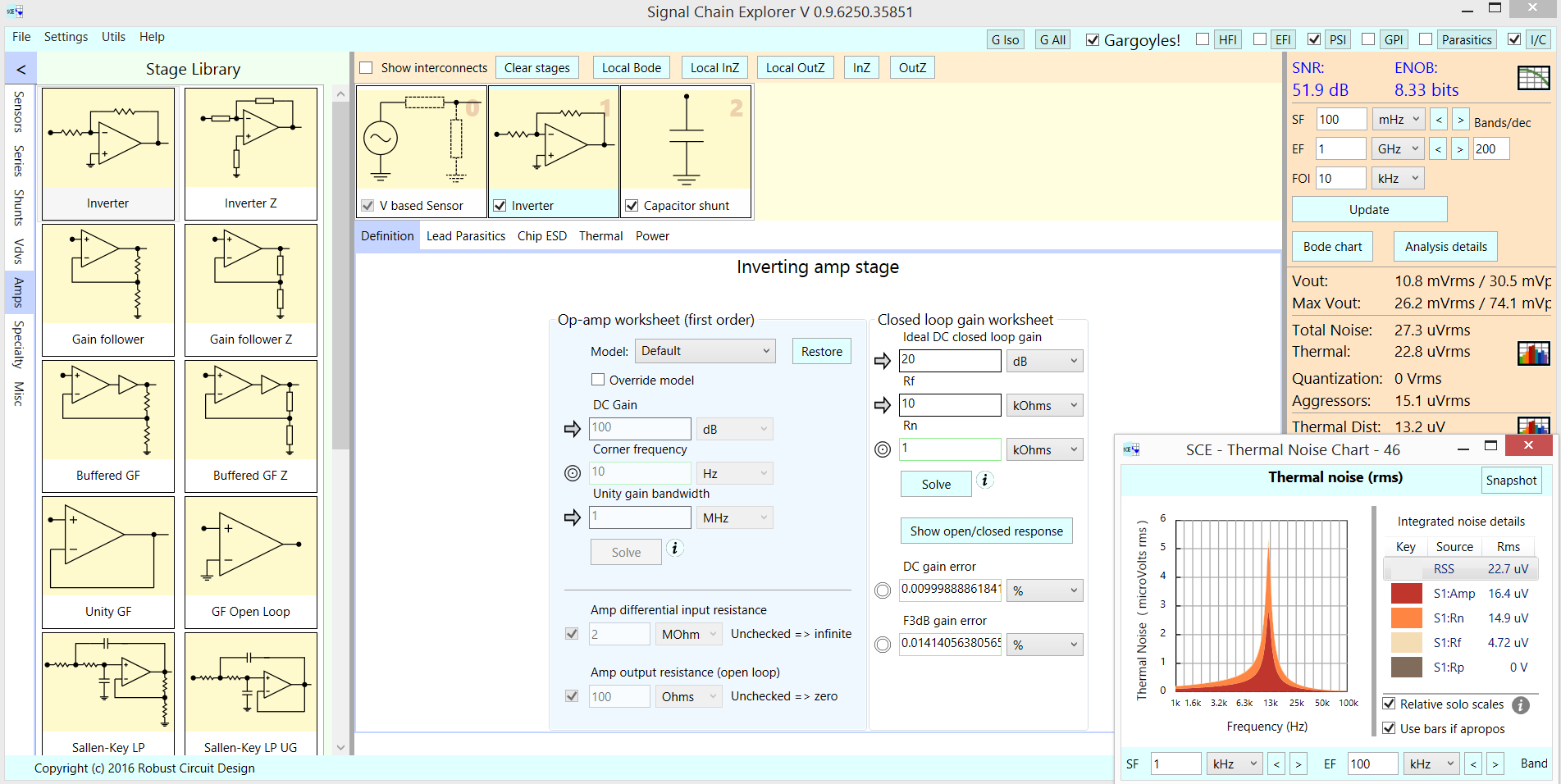
The output current, and output voltage, have other surprises: thermal noise and power supply (deterministic) noise. Those cause a wiggling of the output, even when Vin is fixed. In this next screenshot, look on the lower right, to read the error from ThermalNoise and from Aggressors (the only activated Aggressor is PSI --- power supply interferer --- show in topright checkbox). Notice the 22.8uV of Thermal Noise and the 15uV of (60Hz, 120Hz) Power Supply noise.

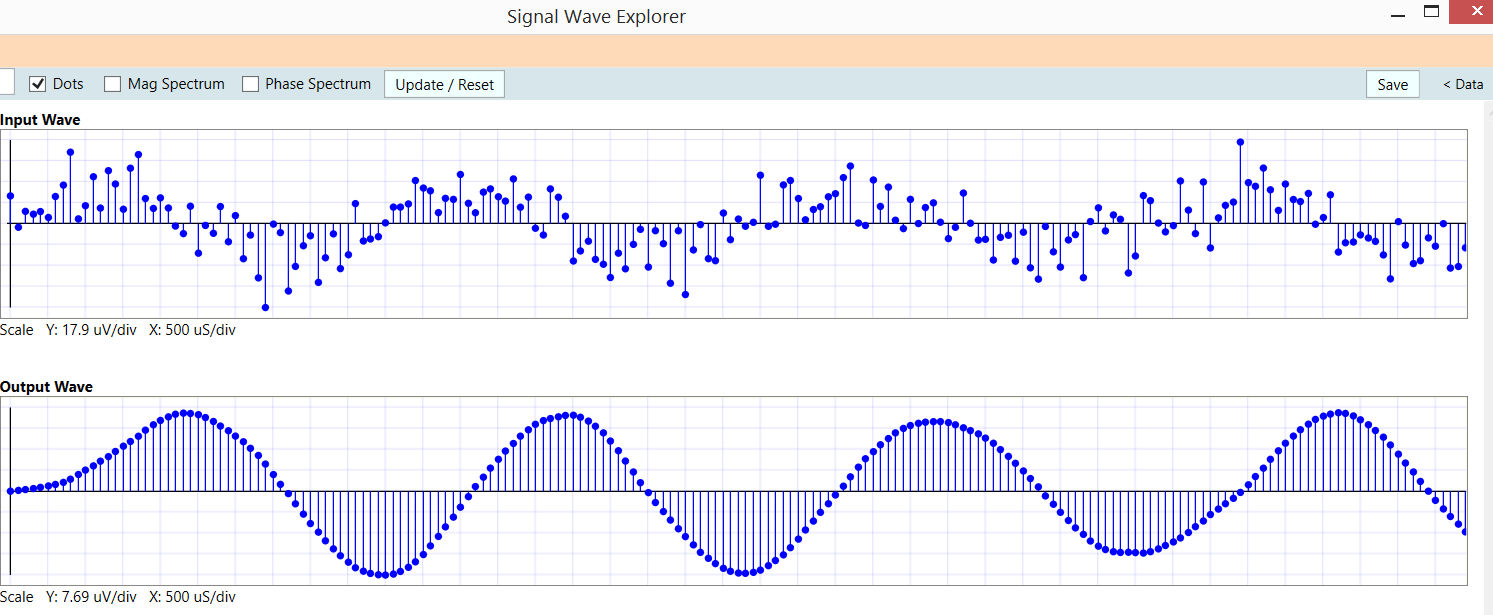
Here is what a 25uV peak signal, at 200Hz, with added 1:1 (0dB) noise power and signal noise, into a 200Hz LC filter. Notice most of the OpAmp noise is gone; we see some wandering of the sinusoid and some "distortion" which is just the noise not being totally removed so that energy upsets the sinusoid shape. The Operational Amplifier is very useful, on PCBs or on silicon, but the physics, and math, involved are worth learning so you do not expect too much yet can get excellent performance.

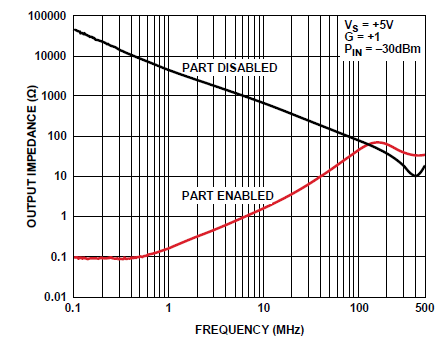
Here is a plot of Zout versus frequency, for an opamp with UGBW of 100MHz. The plot is particularly interesting because the opamp has a ChipSelect pin, thus we see Zout with output transistors controlling the output pin and with those transistors disabled. Up near 500MHz, the Zout nears 30 Ohms, which is impedance of 10pF. Also note the dip in impedance; 10pF and 10nH (Vout pin and VDD pin inductances) resonate at 500MHz.

I'm thinking the 30 ohms Zout is the NPN reac in parallel with PNP reac, where reac is 0.026/Ie_ma thus 0.5ma produces 52 ohms in both emitters, which in parallel become 26 ohms.

Answer 4

The model of the ideal op amp shows a dependent voltage source with no output resistance as the output of the device. Remember on practical devices you see 2 power supply pins.

Answer 5

Ideally, an opamp has an infinite input impedance (and thus zero input current) and an infinite open loop gain , along with infinite output voltage range, bandwidth and slew rate and zero impedance at output (and thus infinite output current) with zero noise. But in reality that is far from being true. An real opamp has:

1. High, but finite input impedance, so a little input current (a few tens nanoapms to only some picoamps I think);
2. Low, but non-zero output impedance, with an output current of a few miniamps to hundreds of miliamps (like 200 mA) for power opamps;
3. Finite bandwidth (it is easy to find one with over 2 MHz bandwidth for a small price);
4. Finite slew rate of, commonly, 5 to 100 V / microsecond;
5. Output voltage limited to input voltage.

Always check the datasheets before buying any component. That can even help you to understand what are the important parameters for a type of electrical or electronic component.