What is the amplification when both input terminals of an opamp are at same potential? [closed]

Question

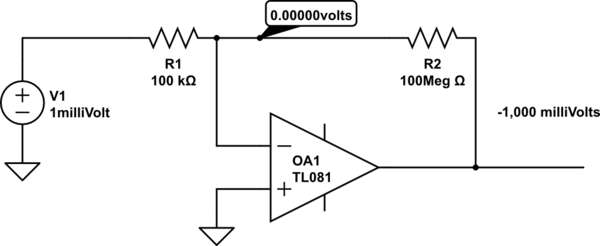
(Ideal opamp discussed here)

I know

Vd=Vo/(V1-V2) ....eq 1

where Vd is differential gain, V1 and V2 are the voltage at the inverting and non inverting terminals and Vo is the output voltage.

Again

A=Vo/Vd,(A = amplification)....eq 2

If V1=V2 then V1-V2=0 in equation 1 then Vd becomes infinite as eq1 becomes:

Vd=Vo/(V1-V2)

Vd=Vo/0 => Vd=infinite

As a result eq 2 becomes

A = Vo/Vd

A = Vo/infinity [ As Vd=infinity]

A = 0

But the book says the amplification is infinite in such a case.What is going on?

Answer 1

Gain is a property of a circuit, not a signal. We normally consider the gain of an opamp to be independent of its inputs. For an ideal opamp, the gain is always infinite.

You're probably confused because your math is wrong. If you do this:

$$V_O = A({V_+ - V_-})$$

Then you can divide \$V_O\$ by the differential voltage to get the gain. But it doesn't work when the output voltage is zero:

$$V_O = A(V - V) = A(0) = 0$$ $$A = \frac 0 0 = \mathrm{undefined}$$

We expect something like this because when the input and output are both zero, the gain could be anything! Infinity, 100, 2, -5...

Answer 2

Ideally the amplification is infinite. But such an amplifier would be unstable, oscillating, and thus unusable. We use that "infinite" model to allow back of envelop design, for the VirtualGround approach.

Numerous OpAmps have 120db (1,000,000x) amplification, needing only 1.000 microvolt to produce 1.000 Volts output.

Typically the amplification is not known to within 50%(6dB), and also varies with temperature or output current or VDD, by another 50%(another 6dB).

To produce predictable, even precise gains from the OpAmp+Resistors, we happily waste surplus amplification; below, we use OpAmp with DC gain of 150dB (31 Million) openloop, to provide predictably-accurate low frequency gain:

We use the formula $$Avcl = G/(1+GH)$$ and for LARGE G (G>>>>>H), we achieve accuracy limited by the resistor ratio and fluctuations in G. Multiply G*H, here G=31Million and H= 1.0e-4, thus GH = 3,100 and our DC error will be 0.03%. What happens if G varies +-6dB (2:1)?