Did I wire this correctly? Trying to understand Thevenin and Loading of circuits

Question

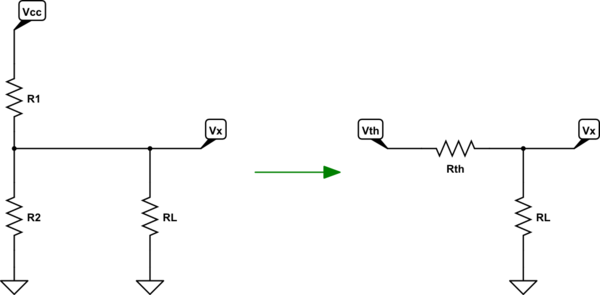
So right now I'm learning about RThevenin and VThevenin of a circuit and how to calculate what the voltage will be once you load the circuit. I am trying to build this circuit:

I am not using these values though. I am using Vin 12.8 volts from a 8AA battery pack. I picked this power supply because I wanted something that I know could fluctuate. For R1 and R2 I am using 1k ohms. For my RL load I am using 100k ohms. The Vout is 6.4 volts with the voltage divider of R1 and R2. Now here is my question... If I put the Rl load (100k ohms) also with the voltage divider shouldn't the Vout or the Vin become a lot lesser than 12.8 or 6.4? Here is how I wired up the circuit:

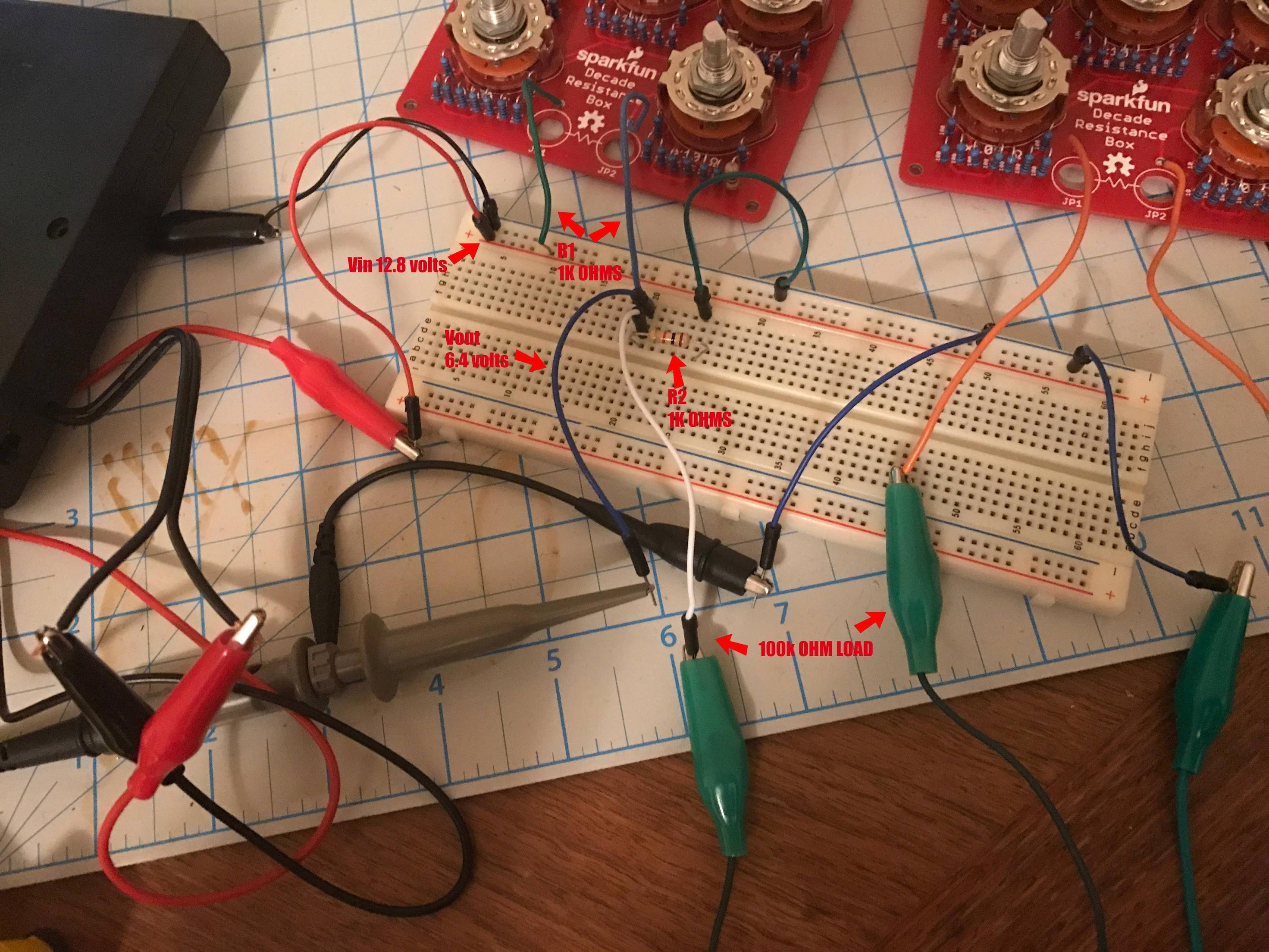
Thank you for any help guys :)

Answer 1

Assume the following schematic and that you are measuring your voltages relative to the ground symbol shown:

^{simulate this circuit – Schematic created using CircuitLab}

To use Thevenin, you first reduce the three items, \$R_1\$ and \$R_2\$ and \$V_{CC}\$, into two equivalents: \$V_{TH}\$ and \$R_{TH}\$. Ignore \$R_L\$ during this process. (As shown above.) To do that, given you've said that \$R_1=R_2=100\:\Omega\$ and \$V_{CC}=12.8\:\textrm{V}\$, simply find that \$R_{TH}=R_!\vert\vert R_2 =\frac{R_1\cdot R_2}{R_1+R_2}=500\:\Omega\$ and that \$V_{TH}=V_{CC}\cdot\frac{R_2}{R_1+R_2}=6.4\:\textrm{V}\$. That's your new circuit.

With \$R_L=100\:\textrm{k}\Omega\$, you now find that \$V_X=V_{TH}\cdot\frac{R_L}{R_L+T_{TH}}\approx 6.37\:\textrm{V}\$. Not much change. This should be obvious to you because \$R_L\$ is close to an open circuit, compared to \$R_{TH}\$. So it won't have much impact. Very little current will flow through it compared to what \$R_{TH}\$ can dish out.

But if you make \$R_L\$ closer in value, then you will see an effect. Suppose you use \$R_L=4.7\:\textrm{k}\Omega\$. Then you should find that \$V_X\approx 5.78\:\textrm{V}\$, which is enough to notice. Dropping \$R_L=1\:\textrm{k}\Omega\$, you should find \$V_X\approx 4.27\:\textrm{V}\$. Even more noticeable. Etc.

Answer 2

No, what you see is what you'd expect. As jonk points out in the comments, putting a 100k resistor in parallel with a 1k resistor gives you something very close to a 1k resistor.

Let's look at this in terms of Thevenin equivalents since that's what you're interested in. The Thevenin voltage from \$V_{in}\$, \$R_1\$, and \$R_2\$ is:

$$V_{th} = V_{in} \frac {R_2} {R_1 + R_2} = 12.8 \mathrm V \frac {1 \mathrm k\Omega} {1 \mathrm k\Omega + 1 \mathrm k\Omega} = 6.4 \mathrm V$$

The Thevenin resistance is:

$$R_{th} = R1 || R2 = \frac {1} {\frac 1 {R1} + \frac 1 {R2}} = \frac 1 {\frac 1 {1\mathrm k\Omega} + \frac 1 {1\mathrm k\Omega}} = 500\Omega$$

When you add the load, you get a voltage divider between \$R_{th}\$ and the load:

$$V_{load} = V_{th} \frac {R_{load}} {R_{th} + R_{load}} = 6.4\mathrm V \frac {100\mathrm k\Omega} {500\Omega + 100\mathrm k\Omega} = 6.37\mathrm V$$

So, 99.5% of the unloaded voltage -- practically the same.