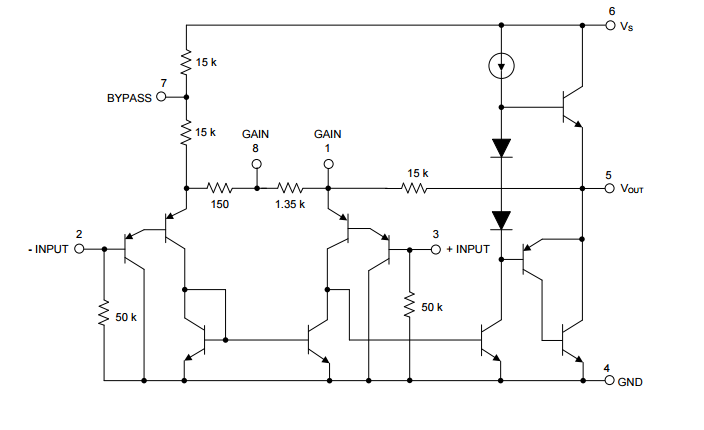
I want to solve this exercise in the figure below.The question is "Recognise as many known Sub-circuits as you can in LM386 circuit".I tried it and found only that the transistor down and in the left hand is a current mirror and active load.Also the output looks like Class A amplifier.Can anyone help me to find other sub-circuits.
\$\begingroup\$
\$\endgroup\$
6
-
1\$\begingroup\$ How about Szilaki pair, Darlington pair, differential pair with emitter degeneration. Class B output stage, Emitter follower. \$\endgroup\$– Kevin WhiteCommented May 2, 2017 at 21:20
-
1\$\begingroup\$ Try read this electrosmash.com/lm386-analysis But first you should study classical BJT op amp circuit. shop.emscdn.com/KitInstrux/741/741_principles_RevA104.pdf \$\endgroup\$– G36Commented May 2, 2017 at 21:40
-
1\$\begingroup\$ This also may be handy: ti.com/lit/an/snoa737/snoa737.pdf and from the same page electrosmash.com/jrc4558-analysis \$\endgroup\$– G36Commented May 2, 2017 at 21:51
-
1\$\begingroup\$ If you go to the bottom of my answer here, electronics.stackexchange.com/questions/281753/… , you will find some discussion that may help you some. \$\endgroup\$– jonkCommented May 2, 2017 at 22:12
-
1\$\begingroup\$ Oh. And I probably should have included this, as well: electronics.stackexchange.com/questions/273867/… Sorry about that. \$\endgroup\$– jonkCommented May 2, 2017 at 22:28
|
Show 1 more comment
1 Answer
\$\begingroup\$
\$\endgroup\$
1
We are not here to do your homework for you. If you really knew these sub-circuits, they would jump out at you.
However, look up things called differential pair, darlington, and cascode. You should not only just try to identify them, but study them to understand how they work in their own right. These can be useful building blocks when designing circuits, but more importantly, the concepts behind them are essential for designing a wide variety of circuits.
This is a worthwhile assignment, even if it appears to be just about silly naming conventions at first glance.
answered May 2, 2017 at 22:02
-
\$\begingroup\$ Mr Olin Lathrop thank you for being patient and helping me improve.I really appreciate your help. \$\endgroup\$– elecV1Commented May 3, 2017 at 7:04