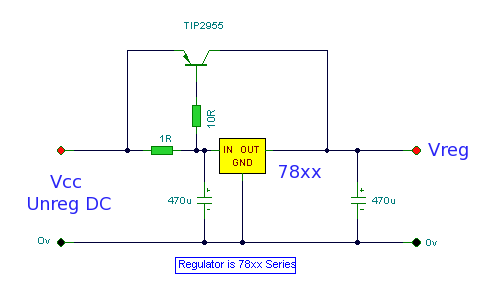
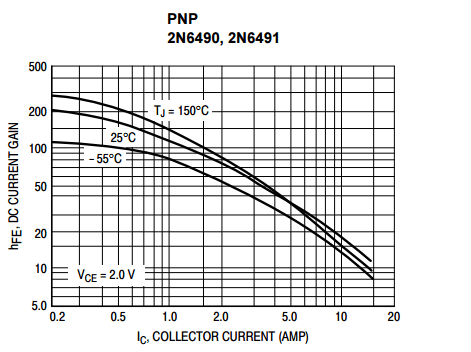
I get the following equation for computing \$R_1\$:
$$R_1=\frac{V_T\cdot \operatorname{ln}\left(\frac{I_C}{I_{SAT}}\right)+ \frac{I_C\cdot R_2}{\beta}}{I_L - I_C}$$
Which, given: \$V_T=26\:\textrm{mV}\$ (room temp), a model value I found of \$I_{SAT}=65\:\textrm{fA}\$, your \$\beta=50\$, \$I_C=3\:\textrm{A}\$, \$R_2=10\:\Omega\$, and \$I_L=6\:\textrm{A}\$, I get \$R_1\approx 473\:\textrm{m}\Omega\$. The nearest standard value would be \$470\:\textrm{m}\Omega\$, which leads to:
$$I_L=\frac{\beta\cdot V_T}{\beta\cdot R_1 + R_2}\cdot\operatorname{LambertW}\left(\frac{I_{SAT}}{\left(\frac{\beta\cdot V_T}{\beta\cdot R_1 + R_2}\right)}\cdot e^{I_L\cdot\frac{R_1}{V_T}}\right)\approx 2.988\:\textrm{A}$$
(If you are interested in what the LambertW function is [how it is defined] and in seeing a fully worked example on how to apply it to solving problems like these, then see: Differential and Multistage Amplifiers(BJT).)
Which may be close enough.
I'm sure you can work out the power dissipations for the components from here.
Just to make this more complete, using the above values and \$R_1=470\:\textrm{m}\Omega\$, I get the collector current for the bypass BJT to be:
$$\begin{array}{l|c|c|c}
_\beta\quad ^{I_{SAT}:} & 30\times 10^{-15}\:\textrm{A} & 65\times 10^{-15}\:\textrm{A} & 100\times 10^{-15}\:\textrm{A}\\\hline 40 & 2.76\:\textrm{A} & 2.78\:\textrm{A} & 2.80\:\textrm{A}\\50 & 2.96\:\textrm{A} & 2.99\:\textrm{A} & 3.00\:\textrm{A}\\60 & 3.11\:\textrm{A} & 3.14\:\textrm{A} & 3.16\:\textrm{A} & \\ 80 & 3.33\:\textrm{A} & 3.36\:\textrm{A} & 3.38\:\textrm{A}
\end{array}$$
As you can see, substantial \$\beta\$ variations probably have the largest effect on the current sharing. Probably not a surprising result. But variations on \$V_{BE}\$ due to the saturation current variations, \$I_{SAT}\$ are of much less importance (when room temperature is held constant.)
None of the above deals with temperature variations as the BJT heats up under load. You'll need to examine the equations with variations in \$V_T\$. I expect the temperature issue to be an important consideration. So you may want to figure out the expected temperature rise in your BJT, based upon the value for \$I_L\$, the \$V_{CE}\$ drop and the base current and the \$V_{BE}\$ drop, together with the thermal resistance you expect, to work out that temperature. Once you have that estimate, plug that into the above equations and see what you get. Keep in mind that both \$V_T\$ and also \$I_{SAT}\$ are functions of temperature and that the latter one dominates and overwhelms the other, so that you can expect a shift in the \$V_{BE}\$ of about \$-2\:\frac{\textrm{mV}}{^\circ C}\$ to \$-2.4\:\frac{\textrm{mV}}{^\circ C}\$.
It was interesting enough for me to check on the thermal details. So worked out the saturation current differences (it goes by a power of 3.) A \$45\:^\circ\textrm{C}\$ rise over ambient would shift \$V_T\$ to about \$29.6\:\textrm{mV}\$ but it would also shift \$I_{SAT}\$ from \$65\:\textrm{fA}\$ to about \$150\:\textrm{fA}\$. Hence, the collector current in the bypass BJT goes from about \$3\:\textrm{A}\$ to about \$2.9\:\textrm{A}\$, instead. That provides a rough idea what to expect with temperature changes, I think.