Is there a reason why we don't use higher rated voltage capacitors?

Question

Recently purchased some components from a BOM for restoring an old tube radio, however it got me wondering about the capacitors and the voltage ratings..

Part of the order contained two 10uF electrolytic capacitors but at different ratings, one at 1000V and one at 630V (for what it's worth, the voltage I'm working with here is ~440V). Would it matter if I were to use just two 1000V ones? What are any downsides in using a higher rated capacitor than what is called for?

I also stumbled upon this in a similar question that was asked and would appreciate any help in understanding it:

My assumptions are: these are all the same ~4.7uF capacitance, just at varying voltage ratings; and a capacitor is inoperable at voltages higher than what it's rated for. So let's compare the two extremes, 0603 and 1812, at ~3V. It seems the capacitance of the 0603 is now ~2.5uF meanwhile the 1812 is still high up at ~4.7uF. This indicates (to me) what should be a noticeable change in behaviour when comparing the two in a 1-5V application. So what would it be?

I know this is pretty loaded and there's a lot of questions going on. I definitely digress here and there, please forgive me. I'd appreciate as much info at a noob-friendly level as possible. Thanks!

EDIT: Here is the post I've already read, also source of image used.

Answer 1

Part of the order contained two 10uF electrolytic capacitors but at different ratings, one at 1000V and one at 630V (for what it's worth, the voltage I'm working with here is ~440V).

If you're sure both have 440V on them, you can use 600V for both, it's likely to be cheaper. I wonder why the original was rated at 1kV.

Would it matter if I were to use just two 1000V ones? What are any downsides in using a higher rated capacitor than what is called for?

Downside is price and bulk. Although modern caps are likely to be smaller than the ones you are replacing, so this shouldn't be a problem.

Now, to add to the other answers...

High-K ceramics like X7R etc are crap dielectrics if you consider the dC/dV, the capacitance varies a lot, it also varies with temperature. Also they are piezoelectric. Never use those in the signal path, for filtering, or for decoupling a high impedance node like a VREF (you'd get a piezo microphone).

So you may wonder why people use them so much. The reason is that they are very good for decoupling power supplies. Since the voltage is constant, dC/dV distortion doesn't matter. And ceramics have many advantages:

They are very cheap and give a high capacitance per volume. They withstand very high temperatures, so they can be surface-mounted directly on the board. This results in very low inductance, which is excellent for decoupling.

Note NP0 ceramics are another story, they are extremely linear and accurate.

EDIT

"High-K" means "High dielectric constant". \$ \kappa \$ is basically \$ \epsilon_r \$ Two plates with a bit of dielectric between them make a capacitor of value:

\$ \frac{Area * \epsilon_0 * \epsilon_r}{Thickness} \$

A material which is a good insulator can be thinner, so you get more capacitance per volume.

And a material with high dielectric constant \$ \kappa \$ or \$ \epsilon_r \$ also gives higher capacitance per volume.

Polypropylene has a dielectric constant of 2.2.

Barium Titanate (one of the High-K ceramics) has 7000. So, it packs a lot more capacitance into much less volume.

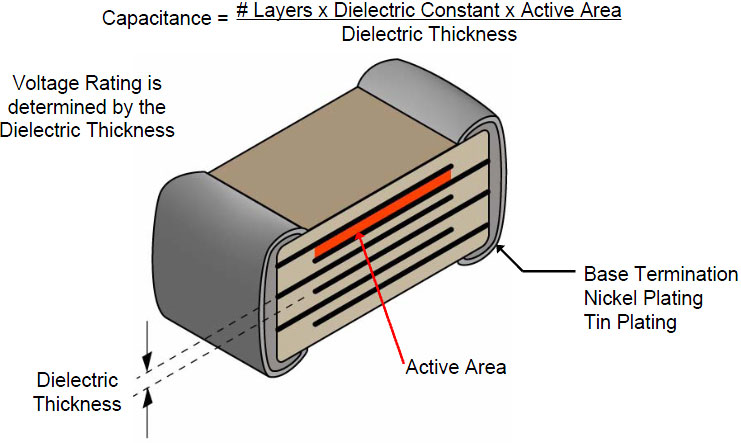
Plate thickness can get down to 0.5µm these days.

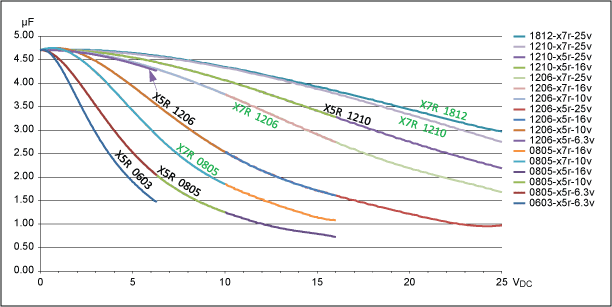
Drawback of these materials is that dielectric constant gets lower with higher electric field. A higher voltage rated X7R ceramic cap (say, 25V versus 6V) will have thicker plates, therefore electric field is lower, therefore its capacitance drops less at the same voltage (say, 3.3V for both caps).

Same if you buy a larger part (1206 is physically larger than 0603 for example) You get thicker plates, and possibly the manufacturer can use a less "extreme high K" material so capacitance drops less.

This explains the curves you posted. Note 1812, 1206 etc are package sizes.

This is off topic relative to your electrolytic caps, but since you asked ;)

Answer 2

Several unrelated questions.

Ceramic capacitors suffer from what's called DC bias. The higher the DC voltage across them, the less actual capacitance they have. So your 0805 capacitor at 10V is only 1.5uF effective capacitor. This has to do with how the crystals align in the dielectric which is above and beyond the scope here. Small ceramic caps (NP0/C0G) do not exhibit this effect. Larger caps show this effect less because they have larger volume and hence less capacitance per cubic millimetre than the smaller physical sizes. They can also be manufactured for higher voltage tolerance for the same reason.

With electrolytes it depends if there's any downside. You'd have to check the capacitor datasheet. As a rule of thumb, same diameter cans have similar ESR and hence would work more or less the same in high-current-ripple applications (=SMPS). I don't think tube radio qualifies as high ripple current.

Nb, capacitors at large voltages are dangerous, you can kill yourself with a 47µF capacitor charged to 440V. I'd hate to try that with 4.7µF either. Pain threshold at 400V is 28 nano farads.

Answer 3

To answer the ceramic capacitor part of your question ...

As the manufacturer tries to push more uFs into a smaller space, he has to use a higher K ceramic. Generally, the higher the K, the worse the voltage sensitivity.

This is why it's essential when specifying ceramic capacitors, if capacity reduction with voltage is an issue, that you specify a specific case size from a specific manufacturer in a specific value and voltage rating.

In the same voltage and capacitance rating, as the case size falls, the voltage sensitivity will increase, even if the ceramic has the same designation ( the X7R or Y5U bit). This designation covers only the tempco, NOT the voltage sensitivity.

Answer 4

Can you say "distortion"? The classic equation Q = C * V, differentiated against time, becomes

$$ dQ/dT = C * dV/dT + V * dC/dT$$

However, we can differentiate against voltage (change in voltage), and we get

$$ dQ/dV = C * dV/dV + V * dC/dV$$

The first term is "C * 1" as we expect to describe stored charge.

That second term "V * dC/dV" is the distortion. For that leftmost capacitor, in the 2 volt and 3 volt region, the dC/dV is 30% per volt. Lots of charging error there.