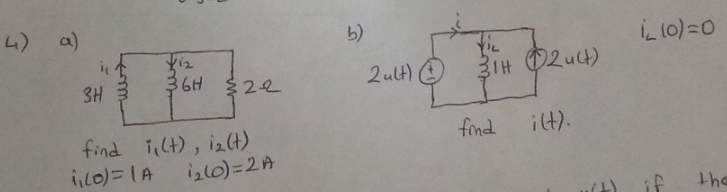This is a question from my intro circuits class that I can't figure out the answer to:
Of course it's very simple, but I had a professor who was incomprehensible most of the time, and my textbook doesn't have any examples similar to this. Would someone please either point me in the right direction to answer this or suggest a good and free resource for me to use to teach myself?
Since this is homework, I won't solve this completely for you, but I'll show you how to set up the equations:
First question "a". Let's name the bottom node "ground", and the top node "node 1", and call its voltage "v1". Now for each of the elements you can write a branch equation:
\$\dfrac{\mathrm{d}i_1}{\mathrm{d}t} = -\dfrac{v_1(t)}{3H}\$ (\$i_1\$ directed opposite of passive reference convention)
\$\dfrac{\mathrm{d}i_2}{\mathrm{d}t} = \dfrac{v_1(t)}{6H}\$
\$i_R(t) = \dfrac{v_1(t)}{2\Omega}\$
You also have a node equation for node 1:
\$i_1(t) = i_2(t) + i_R(t)\$
Given the initial conditions, you can also work out that the resistor current at t=0 is -1 A, and so \$v_1(0) = -1A \cdot 2 \Omega = -2V\$
From here you should be able to work out a solution for the different currents over time. Since you only have one storage element (the two inductors in parallel are equivalent to a single inductor with 6*3/(6+3) = 2 H inductance), you'll most likely end up with v1(t) decaying exponentially, and then being able to work out the individual inductor currents from there.
For equation (b), follow the same method: write all the independent branch and node equations you can, then combine and simplify until you have a solvable set of equations.
Since The Photon has covered the 1st problem, I'll chime in with a hint on the 2nd problem: the current source should not be a factor in your calculation of the inductor current, i.e., the inductor current is unaffected by the current source.
