Hint 1) Installing a short circuit across nodes A and B places a zero-ohm resistance in parallel with resistor R2, which effectively eliminates (removes) R2 from the circuit:
$$
R_{EQ}=R2\,||\,0\,\Omega=\frac{R2\cdot 0\,\Omega}{R2+0\,\Omega}=0\,\Omega
$$
So redraw the circuit with the short circuit installed between nodes A and B, and with resistor R2 removed.
Hint 2) After the short circuit is installed between nodes A and B, by inspection we know that the voltage at node 'A' (relative to the reference potential at node 'B') must be zero volts—i.e., \$V_{AB}=0\,V\$. In other words, the voltage across current source I3 is zero volts. (n.b. An ideal current source is a mathematical model, and the voltage across an ideal current source can range from zero volts to infinite volts.)
Hint 3) From Kirchhoff's Current Law (KCL) we know that the sum of all currents entering node 'A' must equal the sum of all currents exiting node 'A'. Or alternatively, the sum of all currents entering and exiting node A must equal zero.
$$
\Sigma(currents\;entering\;A)=\Sigma(currents\;exiting\;A)\\
\Rightarrow \Sigma(currents\;@\;A) = 0
$$
With AB shorted we see by inspection that the voltage at node A is zero volts—i.e., \$V_{AB}=0\,V\$. Therefore, the voltage polarity across resistor R1 is such that a current \$I_{R_1}\$ flows from voltage source V1, through R1, and into node A. You can use Ohm's Law to calculate the value of current \$I_{R_1}\$. The current produced by current source I3 also flows into node A. And according to KCL the the sum of the currents flowing into node A must equal the sum of the currents flowing out of node A, through the short, and into node B, \$\therefore I_{R_1}+I3=I_{AB}\$.
Hint 4) Thevenin Equivalent circuit. In your original circuit with R2 installed and AB open, let current \$I_{R_1}\$ flow from V1, through R1, into node A. Also, let current \$I_{R_2}\$ flow from node A, through R2, into node B. Find \$R_{TH}\$ by opening AB, turning OFF all independent sources (V1=0V, I3=0A), and calculating the equivalent resistance across AB:
$$
R_{TH}=R1\,||\,R2=\frac{R1 \cdot R2}{R1+R2}
$$
Find \$V_{TH}\$ by applying nodal analysis (KCL) at node A with AB open:
$$
\Sigma(currents\;entering\;A)=\Sigma(currents\;exiting\;A)\\
\Rightarrow I_{R_1}+I3=I_{R_2}\\
\Rightarrow \frac{V_{R_1}}{R1}+I3=\frac{V_{R_2}}{R2}\\
\Rightarrow \frac{V1-V_{AB}}{R1}+I3=\frac{V_{AB}}{R2}
$$
Noting that \$V_{TH}=V_{AB}\$ with AB open, solve for \$V_{AB}\$. After solving for \$R_{TH}\$ and \$V_{TH}\$, place a short across AB and calculate the current through the short, \$I_{AB}=V_{TH}/R_{TH}\$.

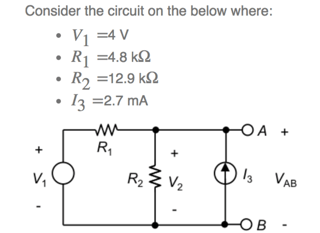
I thought that is would be 2.7 x 10^-3 A... that is 2.7mA .... what else is connected? \$\endgroup\$