4
\$\begingroup\$

Resistors come in a variety of different ratings based on wattage. As a rule, lower wattage resistors are smaller than higher wattage resistors. I understand the wattage determines roughly how much current it can handle before it burns up.

I am a bit confused, however. Shouldn't the area of resistor also determine its conductivity? If you supplied a 1000 watt resistor with 3.3v and 100ma, for instance, would you get the same reading as you would get if you passed the same current through a 1/8 watt resistor? Furthermore, if you do get the same reading, shouldn't the area of the larger resistor affect the resistance? If you get a different reading, shouldn't resistors specify a power range instead just a maximum rating?

\$\endgroup\$
5
  • 1
    \$\begingroup\$ Physical size is not the only thing contributing to a resistor's power capacity. Material is crucial. Lower-rated resistors are carbon, and higher-ones are often wire-wound. \$\endgroup\$
    – Reinderien
    Commented Sep 24, 2018 at 18:06
  • 1
    \$\begingroup\$ The part you see is just the "package". The actual electrical resistance (that one measures with an ohm meter) is created by "stuff" internal to the package. Cut one open and see! \$\endgroup\$
    – mike65535
    Commented Sep 24, 2018 at 18:14
  • \$\begingroup\$ Yes, that's true, but there would be no reason to make them larger than they have to be if the resistive material is the same size. \$\endgroup\$
    – user148298
    Commented Sep 24, 2018 at 18:16
  • \$\begingroup\$ You would need to make them larger to dissipate a larger amount of heat. A resistor at 1W will need to dissipate a lot less heat than a 100W if ran at full power. Surface area has a lot to due with how much heat you can dissipate. \$\endgroup\$
    – Robert Fay
    Commented Sep 24, 2018 at 18:46
  • \$\begingroup\$ Also it makes economic sense (for part manufacturers and assemblers) to have a fixed range of sizes. So you can buy plenty of things that don't allow you to meet all the specs at once, like a 1/4W 10MΩ resistor with a max voltage that only allows it to dissipate 1/40W \$\endgroup\$
    – Chris H
    Commented Sep 25, 2018 at 8:47

3 Answers 3

Reset to default
6
\$\begingroup\$

enter image description here

Figure 1. A carbon film resistor. Photo by Shaddack from Wikimedia Commons.

This photo shows the internal construction of an unpainted carbon film resistor. A spiral cut has been made in the film through to the ceramic former. For a given film resistivity a range of resistance values can be created by varying the pitch and width of the cut in the film. This one is a little suspect as all the heat will be dissipated in the high-resistance section where the spiral is so they are not spreading it evenly across the film. Presumably this is taken care of in the design calculations.

Shouldn't the area of resistor also determine its conductivity?

The resistivity and thickness of the uncut material is controllable in manufacture. Then the cutting operation can fine-tune the resistance value.

If you supplied a 1000 watt resistor with 3.3v and 100ma, for instance, would you get the same reading as you would get if you passed the same current through a 1/8 watt resistor?

Only if both are \$ R = \frac {V}{I} = \frac {3.3}{0.1} = 33 \ \Omega \$. The power dissipated in each would be \$ P = I^2R = 0.1^2 \times 33 = 33\ \text {mW} \$ so both would be fine.

Furthermore, if you do get the same reading, shouldn't the area of the larger resistor affect the resistance?

schematic

simulate this circuit – Schematic created using CircuitLab

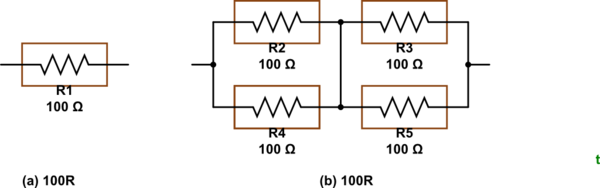
Figure 2. (a) A small 100 Ω resistor and (b) another one that can handle four times as much power.

No. This is taken into account in the design. As shown in Figure 2, if we doubled the width of the track (R2 in parallel with R4) we would halve the resistance but if we series connected another pair (R3 and R5) we would be back at 100 Ω. It's just a matter of design.

If you get a different reading, shouldn't resistors specify a power range instead just a maximum rating?

Everything sorted?

Note that the power rating is determined by the maximum temperature the film can handle. This temperature is reached when the energy gain due to electric heating is equal to the energy lost due to cooling by convection, radiation and conduction. The convection and radiation will be determined by the surface area, \$ A = \pi r^2 l \$ where r is the radius and l is the length. Conduction will be determined mostly by the conduction of the leads and the solder pads.

Videos:

\$\endgroup\$
2
  • \$\begingroup\$ I get it now. I made the assumption that the material and other factors were the same, but the area is larger. If change the area, you must also change the other factors to have the same resistance. \$\endgroup\$
    – user148298
    Commented Sep 25, 2018 at 0:28
  • \$\begingroup\$ I meant the length and thickness of the actual material and geometries. The material may have to change as well. \$\endgroup\$
    – user148298
    Commented Sep 25, 2018 at 0:48
6
\$\begingroup\$

The size or surface area of a resistor is not related to its resistance. It only determines the resistor's power-handling ability.

A 1000 Ohm resistor will measure 1000 Ohms and have the same effect on current in a circuit regardless of its physical size.

Edit: For most resistors, what you see is mostly just a package that protects or covers the actual resistor element. However, the actual resistor element for a high power resistor will be much larger than one for a low power resistor. The resistance is determined by the resistivity and dimensions of the resistor element - a 1000 Ohm 1/4 Watt resistor will be made with a higher resistivity material than a 1000 Ohm 10 Watt resistor.

\$\endgroup\$
3
  • \$\begingroup\$ Can you address @Reinderien's comment? Very interesting. \$\endgroup\$
    – user148298
    Commented Sep 24, 2018 at 18:07
  • \$\begingroup\$ @user148298 how do you think that the comment and the two answers "mesh"? \$\endgroup\$
    – Solar Mike
    Commented Sep 24, 2018 at 18:26
  • \$\begingroup\$ Well, he mentioned material. I guess if you go bigger, then you need to change the material. Correct? \$\endgroup\$
    – user148298
    Commented Sep 25, 2018 at 0:23
4
\$\begingroup\$
Shouldn't the area of resistor also determine its conductivity?

No. Resistors with the same external dimensions can have different thicknesses, width, or other geometries of different materials inside.

For example, a soda straw and a steel shaft may have the same dimensions, but would have vastly different resistance.

\$\endgroup\$
1
  • \$\begingroup\$ Yes. I get it now, I forgot about the material of the actual element. If it's area becomes larger, than the choosen material of the element along witg other factors must compensate for it in order to yield the same resistance. I assumed the material was the same. \$\endgroup\$
    – user148298
    Commented Sep 25, 2018 at 0:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.