How to read a thermocouple chart?

Question

Studying for the PE exam, everything seems to be a breeze, but I cannot for the life of me read a TC chart. Specifically, I have a sample problem in a study guide where the measured voltage is 21.57 mV with a 20C junction and a Type J thermocouple.

How to solve the problem is straight forward. I know I have to account for the junction temperature by adding the voltage at 20C to my measured voltage, then looking up the result in the chart. I, however, cannot read a chart. Just can't do it. I seem to also have found multiple charts and online calculators that contradict eachother, so if somebody could please help me work this out it would be greatly appreciated.

As far as I can tell, my sources of confusion come from these questions:

1. Some charts number from -10 to 0 in columns, others number from 0 to +10 in columns. These appear the same, but the value that I add to or subtract from is always a mystery, specifically because all charts seem to have a far left value and far right value that are the same, and for me it would be intuitive if the right value was always +10.

2. Related to #1, is there a case to use one or the other above?

3. Is looking up a cold junction offset value in any way different than looking up a regular value? In my example problem, I went to my chart to find the mV for 20C, should I be looking at a different chart for cold junctions?

From my sample problem textbook, they found 20C -> 1.537 mV:

From an online chart, I also found 20C -> 1.537 mV:

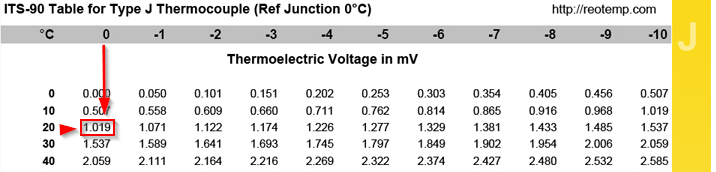
From another chart online, I found 20C -> 1.019 mV:

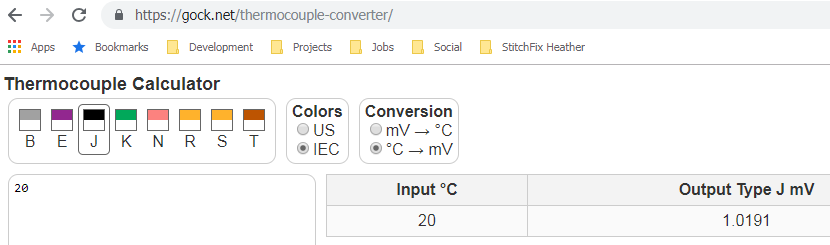
An online calculator I found says 20C -> 1.019 mV:

Fluke's online calculator agrees 20C -> 1.019mV:

The reference book I have available to me was in Fahrenheit, but 68F -> 1.306mV:

All of the values seem to differ by 10C (1.019 mV vs 1.537 mV), except the Fahrenheit value seems to be about halfway, so I'm sure it's no coincidence that my interpretations of the charts are wrong, but using the calculators I just punched it in. Where am I going wrong here?

Answer 1

I realise this thread is kinda old but in case anyone else in the future is curious I will explain why there is confusion and how these charts work.

I won't go into a lot of depth about how thermocouples work because it involves quantum mechanics and would sort of stray from the original point of the question. However at the bare minimum to clear things up you must know that thermocouples operate on the seebek effect, and essentially produces a voltage based on a difference in temperature between 2 different metals.

The confusion is coming from the fact that you cannot get the information you want with the information you are using. Remember that a thermocouple produces a voltage because of a difference in temperature, and so because we are looking for a difference, we must have something to reference against. Otherwise you are just producing a meaningless voltage reading.

Hence the confusion on different temperatures producing the same voltage, but also explains why the voltages are mirrored over 0°C, because you should really think of it as the difference in temperature between the 2 metals in the thermocouple. Rather than a voltage correlating to the temperature of the thermocouple.

Now in order to measure the temperature of the thermocouple you need another way to measure temperature. There are a few ways to do this. 2 of the most common are to either, use another thermocouple in the same circuit with a known temperature using a thermeter or use a thermistor.

There are advantages and disadvantages to both options but which is better is dependent on the use case. Thermocouples have a wide temperature range, low cost, and many different types for many different applications. The thermistor is highly accurate, but is more expensive, and has a much smaller temperature range.

If you were to use 2 thermocouples, you would

#1 take the known thermocouples temperature.

#2 locate the associated voltage on the chart.

#3 subtract the voltage of the known thermocouple from the voltage of the unknown temp thermocouple.

#4 find the sum of the voltage equation and reference the chart for the associated temp.

Hope this is helpful.

Answer 2

I think that the second table in your example is confusing and the first one is wrong.

The clue is to look at what voltage you read from the chart for a temperature difference of zero, because the answer has to be zero volts. In the second chart there are two rows for 0 °C; the first row has 0 V at the right-hand end and negative voltages in the preceding columns, but the second row shows 0 V at the left-hand end followed by positive voltages in the subsequent columns. Therefore the first '0 °C' row must show values for temperature differences from -10 to 0 °C, and the second row for temperature differences from 0 to +10 °C. In between the two '0 °C' rows there should be a new set of column headings reading 0 1 2 3 etc. That would show you that the correct voltage for a +20 °C difference between two type J thermocouple junctions, when the reference junction is at 0 °C, is 1.019 mV.

Your first table seems to be copied from the second one, but without the negative temperature rows, so its column headings are simply wrong for the positive temperature rows that are shown.

Answer 3

The sample problem textbook has incorrectly labeled columns. They should be labeled "+0 +1 +2 +3 ... +9", not with those negative values.

The thermoelectric voltage for 0+0C temperature differential is 0.000V. Thus the leftmost column cannot be labeled -10 - thermocouples don't treat 10C difference in any special way.

You can fix any such table errors by noting that 0C differential must map to 0.000V.