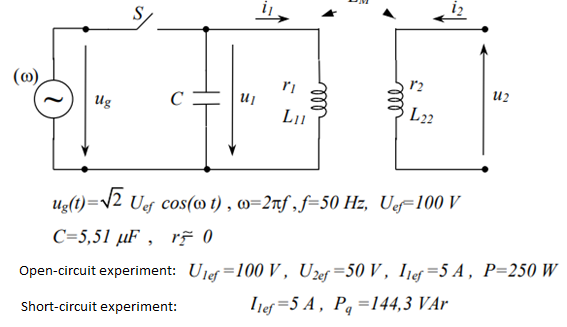
Ok I have the following circuit and data (when the subscript is "ef" it means "rms" values):
I am asked to determine the paramenters of the transformer r1 L11, L22 and LM with the given experimental data.
I had no problem extracting data from the open circuit experiment. Using the fact that the active power is given by $$P=r_1 I_{rms}^2$$
I found $$r_1=10 \Omega$$
Then applying induction law in both primary and secondary leaves us with:
$$u_1(t)=r_1i_1(t)+L_{11}\frac{di_1(t)}{dt}$$ $$u_2(t)=-L_{M}\frac{di_1(t)}{dt}$$
Applying phasor notation and taking the rms values will lead us to obtain
$$L_M=\frac{U_{2_{rms}}}{\omega I_{1_{rms}} }=31.83 mH$$ $$L_{11}=\sqrt{(\frac{U_{1_{rms}}^2}{I_{1_{rms}}^2} - r_1^2) \frac{1}{\omega^2}}=55.13 mH$$
Ok and there is no more data we can extract form the open-circuit experiment.
Passing to the short-circuit experiment I will obtain from induction law again:
$$0=-L_{M}\frac{di_1(t)}{dt}-L_{22}\frac{di_2(t)}{dt}$$
Which leads to
$$L_{22}=\frac{L_M I_{1_{rms}}}{I_{2_{rms}} }$$
Problem now is I don't know the value of the root-mean square of current 2 and have no idea how to find it out. My guess is that I need to use the reactive power. But how? I know from Poynting complex theorem:
$$P_Q= 2\omega ((W_e)_{av} - (W_m)_{av})$$
But, and that is another question I have and would like to get ans answer on? How should I apply this formula. For the electrical energy, should I take the capacitor? But what's the voltage value? The same as the open-circuit experiment? And for the magnetic energy? What inductances should I consider? Do I need to calculate an equivalent circuit?
I'm really confused and would appreciate some help. Thanks!