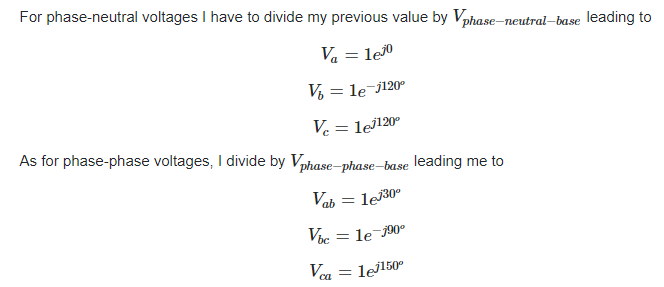Ok, so I had a problem about a three-phase system with the following data
$$V=220kV$$
$$S=150MVA$$
$$\cos \phi=0.85$$
where V is the phase-phase voltage, S is apparent power, and \$\phi \$ is positive (inductive load).
Ok I was asked to find out the phase-neutral voltages, the phase-phase voltages and the currents.
I had no trouble doing it so I just post the outline of my work.
Phase-neutral voltages
$$V_a = \frac{220}{\sqrt{3}} e^{j0}$$ $$V_b = \frac{220}{\sqrt{3}} e^{-j120^o}$$ $$V_c = \frac{220}{\sqrt{3}} e^{j120^o}$$
Phase-phase voltages
$$V_{ab} = V_a-V_b= 220 e^{j30^o}$$ $$V_{bc} = V_b-V_c= 220 e^{-j90^o}$$ $$V_{ca} = V_c-V_a= 220 e^{j150^o}$$
Currents
$$I_{a} = \frac{S}{\sqrt{3}V} e^{-j31.7^o}= 393.6 e^{-j31.7^o}$$ $$I_{b} = 393.6 e^{-j151.7^o}$$ $$I_{c} = 393.6 e^{j88.3^o}$$
And the impedance for each load (a,b and c) is $$Z= \frac{V_a}{I_a}= 322.7 e^{j31.7^o}$$
Now they say for me to imagine that the system becomes unbalanced with b becoming 1.1Z and c becoming 0.9Z
$$I_n= I_a+ I_b + I_c = \frac{V_a}{Z} + \frac{V_b}{1.1Z} + \frac{V_c}{0.9Z} = 68.5 e^{j61.8^o}$$
Now I was asked to redo this using the pu system with given base values
$$V_{phase-phase-base}=220kV$$
$$S_{base}=100 MVA $$
This lead me to
$$V_{phase-neutral-base}=220/\sqrt{3} kV$$ $$I_{base}= \frac{S_{base}}{\sqrt{3}V_{phase-phase-base}}=262.4A$$ $$Z_{base}= \frac{V_{phase-phase-base}}{\sqrt{3}I_{base}}=484 \Omega$$
For phase-neutral voltages I have to divide my previous value by \$ V_{phase-neutral-base}\$ leading to
$$V_a = 1 e^{j0}$$ $$V_b = 1 e^{-j120^o}$$ $$V_c = 1 e^{j120^o}$$
As for phase-phase voltages, I divide by \$V_{phase-phase-base}\$ leading me to
$$V_{ab} = 1 e^{j30^o}$$ $$V_{bc} = 1 e^{-j90^o}$$ $$V_{ca} = 1 e^{j150^o}$$
Now my question is: why if I apply the formulas $$V_{ab} = V_a-V_b$$ $$V_{bc} = V_b-V_c$$ $$V_{ca} = V_c-V_a$$ using pu values the equations don't verify. Is it because I use different base values for each and this is just a question of proportionality?
However, then I continued my problem and obtained the rest of the values:
$$S=\frac{150}{100}=1.5$$
$$I_{a} = \frac{S}{V} e^{-j31.7^o}= 1,5 e^{-j31.7^o}$$ $$I_{b} = 1.5 e^{-j151.7^o}$$ $$I_{c} = 1.5 e^{j88.3^o}$$
Everything looks fine here, because if I multiply the currents by \$I_{base}\$ I obtain the values I did before.
$$Z= \frac{V_a}{I_a}= \frac{1 e^{j0}}{1,5 e^{-j31.7^o}} = 0.667 e^{j31.7^o}$$
Then again, multiplying by \$Z_{base}\$ we obtain the value before.
Finally,
$$I_n= I_a+ I_b + I_c = \frac{V_a}{Z} + \frac{V_b}{1.1Z} + \frac{V_c}{0.9Z} = \frac{1 e^{j0}}{0.667 e^{j31.7^o}} + \frac{1 e^{-j120^o}}{1.1 \times 0.667 e^{j31.7^o}} + \frac{1 e^{j120^o}}{0.9 \times 0.667 e^{j31.7^o}} = 0.261 e^{j61.8^o}$$
And multiplying by \$I_{base}\$ I obtain the same value as before.
So in this last calculations using the pu values works pretty well and I obtain values equivalent to the calculations I did before. The only calculation that's failing is the one about the voltages phase-phase. Why does this happen? What is the mathematical subtlety I am missing here?
Thanks!