I am looking for a way to calculate the quality factor in arbitrary RLC circuits not just standart series or parallel circuits.
It would be great, if anybody could provide a formula and some further readings.
As asked for, here are two examples of my interest:
simulate this circuit – Schematic created using CircuitLab
Well, there can be no general answer.
For a second order lowpass, the Q value is defined as the "quality factor of the complex pole" in the s-plane ("pole Q", symbol Qp). In this case, it is defined as Qp=wp/|2sigma|. Here the quantity wp is the "pole frequency" (magnitude of the vector from the origin to the pole location) and "sigma" is the (negative) real part of the pole.
The same definition applies for a second-order bandpass. However, this Q value is identical to the "bandwidth-Q" with Qp=Q=fo/BW (midfrequency/3dB-bandwidth).
For all higher order systems with n>2, we have more than one pole pair and we can give only the pole-Q for each pole pair, but we cannot define something lke an "overall Q". Exception: For higher-order bandpass you can use the definition as given for n=2 (fo/BW).
Example 1: Your first circuit is a 3rd-order system with one real pole (Qp1=0.5) and a complex pole pair with Qp2 as defined for a 2nd-order system.
Example 2: This is a 4th-order system with two pole pairs and two associated Qp values.
Comment: For finding the Q-values (pole Qs) of the circuit, it does not matter where the output is defined. The Q values are a property of the circuit alone. This is, because only the zeros of the circuit determine if the circuit will act as a lowpass, highpass or bandpass. The pole distribution is independent on the selection of input and output nodes.
Not a complete answer, but if you can compute or infer the location of the poles and/or zeros of the network response in the complex plane, the Q factor is related to their location(s) (heights and distances from the unit line).
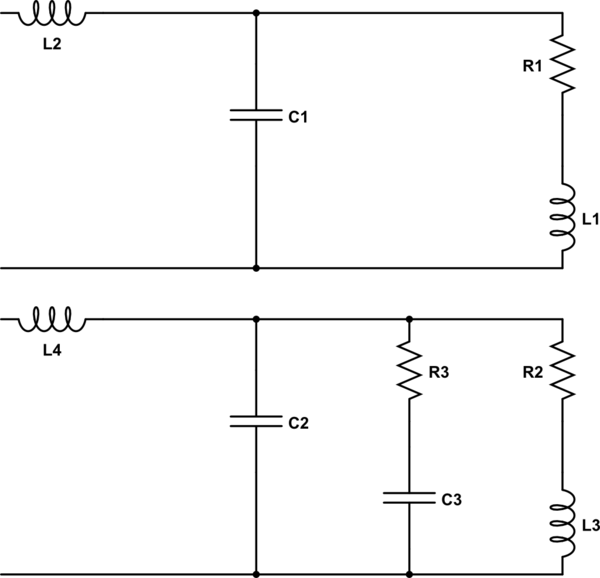
To determine the transfer functions of the two circuits, you can apply the fast analytical circuits techniques or FACTs. Basically, you turn the excitation off and "look" at the energy-storing element's terminals to determine what resistance \$R\$ they offer. Then, combine \$R\$ with the involved \$L\$ or \$C\$ to form time constants. Finally, you assemble the time constants to form the denominator and numerator.
In the first example, I assumed the excitation is a voltage source and you observe the response across \$C_1\$. With the FACTs, I can already see a zero formed by \$L_3\$ and \$R_1\$. Immediately, you have \$N(s)=1+s\frac{L_3}{R_1}\$ (your \$L_1\$ is relabeled \$L_3\$ to have three distinct times constants).
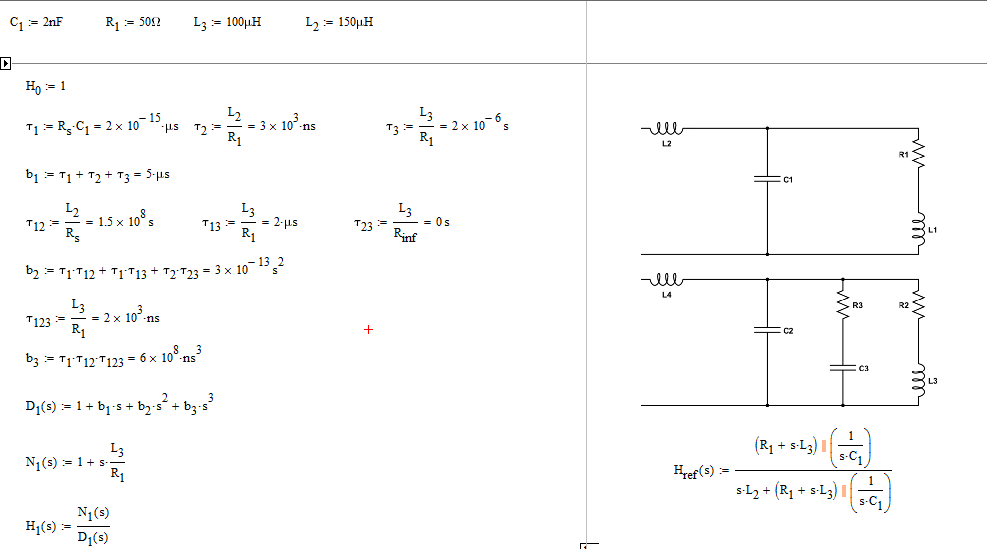
If you do things right, you obtain the results shown in the below Mathcad sheet in which I have quickly factored a quality factor and a resonant frequency:
Finally, you can plot the reference transfer function and the one obtained with the FACTs, they perfectly agree:
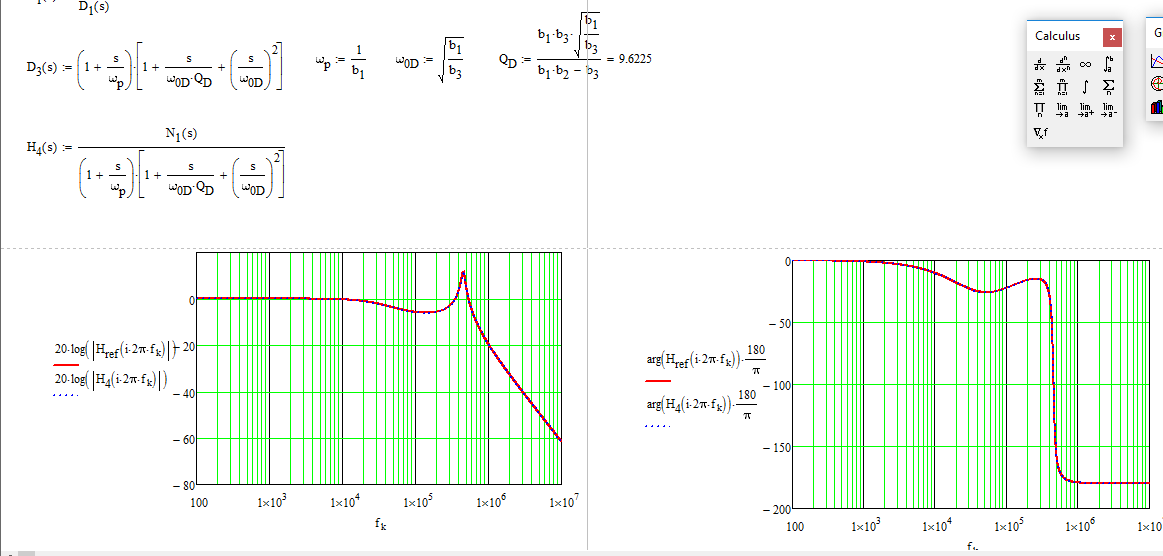
The difficult part is to find the dominant pole and the double poles. You have to check on the time constants to see which dominate the response. An interesting presentation is available here, written by Prof. Bob Ericsson from CoPEC. Actually, writing the transfer function in a factored form is truly the basis of low-entropy formatting. Without this approach in which you can see a quality factor and a resonant frequency, there is no way you can select component values to meet design goals.
Another way to think of "Q" is the ratio of the reactance/resistance. Mearly calculate the complex impedance of those circuits and determine the above ratio and you have its Q.
