Loaded Q-factor of parallel RLC with series resistive load

Question

So I have been studying resonant RLC-circuits, and have come to loaded Q-factors. At present I am trying to figure out the Q-factor of a circuit like this:

^{simulate this circuit – Schematic created using CircuitLab}

My textbook (or lecture notes, rather) claims that the Q-factor of the above circuit will be \$Q_L =\omega_0 C (R // R_{load})\$, i.e. the same as if the load resistor was connected in parallel with the resonator. The only online resource I've found seems to agree (see page 5).

When I try to calculate the Q-factor I instead get

$$Q_L=2 \pi \frac{\mbox{Max energy stored}}{\mbox{Energy lost per cycle}} = 2 \pi \frac{v_2^2 C/2}{(v_1/\sqrt{2})^2/((R+R_{load})f_0)} = \omega_0 C (R_{load} + R) \left(\frac{R}{R+R_{load}}\right)^2=\omega_0 C \frac{R^2}{R+R_{load}}$$

since \$v_2 = \frac{R}{R+R_{load}}v_1\$ at resonance. Have I misunderstood the Q-factor, or messed up my reasoning somewhere?

Answer 1

Regarding any AC analysis on this circuit, the effect of R and Rload are effectively in parallel and will therefore produce the same Q irrespective of whether their parallel value is in the feed position or the shunt position.

Think about the voltage source and its equivalent circuit with R and Rload - forget about L and C for the moment. Try this for size: -

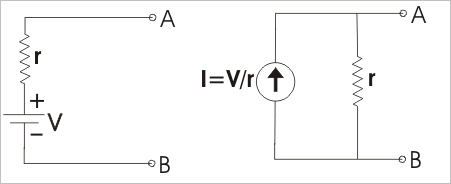
The two circuits are identical and the little_r resistor has changed to be across a current source. This puts it in parallel with any resistor across terminals A and B.

In fact if V1, Rload (or R1) were inside a box and you were not allowed to look inside, you could never know that what was contained was a voltage source in series with a resistor OR a current source in parallel with a resistor - there's no way of telling.

Here's an even more complex scenario: -

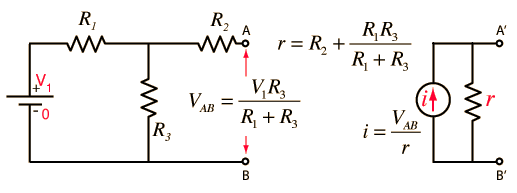
Now, if R2 were zero, the equivalent output impedance is the parallel arrangement of the two other resistors. Does that ring a bell?

It's called Norton's theorum - try googling it - also look up Thevenin's theorum - it operates in the reverse: -

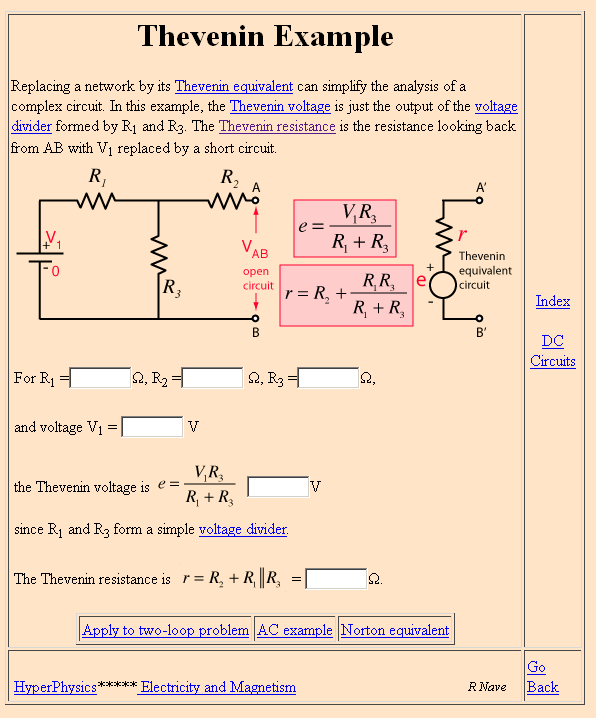
Picture stolen from here. Does this make sense now?

So if you agree that Q = \$\omega C R\$ then you know what value to use for R.

---

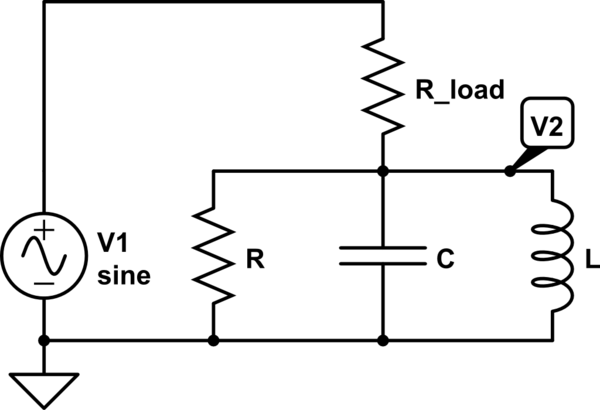
Calculating Q for a parallel LC fed by a voltage source via a resistor. Start with the impedance of a pure LC tuned circuit. This is: -

\$\dfrac{sL}{s^2LC+1}\$ then calculate what Vout would be i.e. the transfer function: -

H(s) = \$\dfrac{\dfrac{sL}{s^2LC+1}}{R + \dfrac{sL}{s^2LC+1}}\$

A bit of algebra and this becomes: -

\$\dfrac{\dfrac{s}{CR}}{s^2+\dfrac{1}{LC} +\dfrac{s}{CR}}\$

The bottom line is clearly (to some LOL) recognizable as the denominator in any damped resonant circuit where the various artefacts are: -

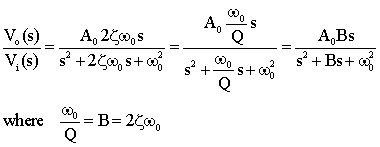
See this document and read the bandpass section to confirm. The above picture is an extract.

So \$2\zeta\omega_0 = \dfrac{1}{CR}\$ and, because Q is \$\dfrac{1}{2\zeta}\$, Q = \$CR\omega_0\$