Calculating load regulation in this zener diode circuit

Question

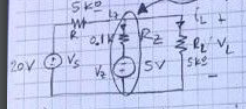
I'm trying to find a good method to calculate the following two values of the circuit below:

$$\bigg(\frac{dV_L}{dV_S}\bigg)_{i_L=constant}=?$$ $$\bigg(\frac{dV_L}{di_L}\bigg)_{V_s=constant}=?\\ \\$$

I'm not sure if my method is correct, but here is what I did for the first one(KCL on node above Rz):

$$\frac{V_s-V_L}{R}=\frac{V_L-5}{R_z}+i_L\\=>(R+R_z)V_L=R_zV_s+5R_L-RR_zi_L\space \space (1)$$

Taking the derivative of VL with respect to Vs and handling iL as a constant, I get the result:

$$\bigg(\frac{dV_L}{dV_S}\bigg)_{i_L=constant}=\frac{R_z}{R+R_z}$$

However (!), if I'd written the last term in KCL like this :

$$\frac{V_s-V_L}{R}=\frac{V_L-5}{R_z}+\frac{V_L}{R_L}$$

I would get a different result. Why? How do I know if I have the correct equation to take the derivative?

Now for the second part.

Why can't this derivative be equal to RL? I can start from equation (1) and get the correct result but again,Ohm's equation is valid as well. How do I know what equation to start from? Each one leads to a different result. I've obviously understood something wrong.

Answer 1

Taking the derivative of VL with respect to Vs and handling iL as a constant, I get the result [...]
However (!), if I'd written the last term in KCL like this: [...] I would get a different result.
Why? How do I know if I have the correct equation to take the derivative?

The difference is that you cannot assume i_L as a constant.
i_L changes when V_s changes. So, the assumption i_L could be handled as a constant is incorrect.