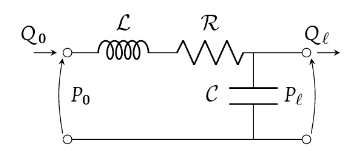
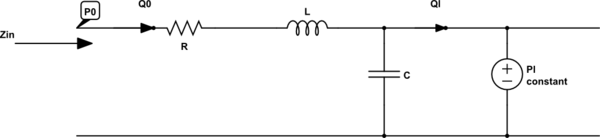
I'm trying to find the impedance of this RLC circuit (sorry for awkward notation of Q instead of I and P instead of V due to that this scheme comes from an analogy of electrical circuits with 0D lumped models for blood flow simulations):
Due to the this circuit, we have:
$$Q_{0} - Q_{\ell} = \mathcal{C} \frac{d P_{\ell}}{dt}$$
$$P_{0} - P_{\ell} = \mathcal{L} \frac{d Q_{0}}{dt} + \mathcal{R} Q_{0}$$
Or in frequency domain:
$$\tilde{Q_{0}}(\omega) - \tilde{Q_{\ell}}(\omega) = j\omega \mathcal{C} \tilde{P_{\ell}}(\omega)$$
$$\tilde{P_{0}}(\omega) - \tilde{P_{\ell}}(\omega) = (\mathcal{R} + j\omega \mathcal{L})\tilde{Q_{0}}(\omega)$$
Or finally:
$$\tilde{P_{0}}(\omega) = (\mathcal{R} + j(\omega \mathcal{L} - \frac{1}{\omega \mathcal{C}}))\tilde{Q_{0}}(\omega) + \frac{j}{\omega\mathcal{C}} \tilde{Q_{\ell}}(\omega)$$
So, I'm stuck here cause I don't how to proceed and find the impedance. I'm not sure if it's correct to take $$\mathcal{R} + j(\omega \mathcal{L} - \frac{1}{\omega \mathcal{C}})$$ as impedance or not. Note that in my scheme I don't have any information about $$\tilde{Q_{\ell}}(\omega)$$ but I might assume that $$P_{\ell}$$ is just a constant value in time-domain (or probably a Dirac delta function in frequency domain).