Error in the direct approach for power flow calculation

Question

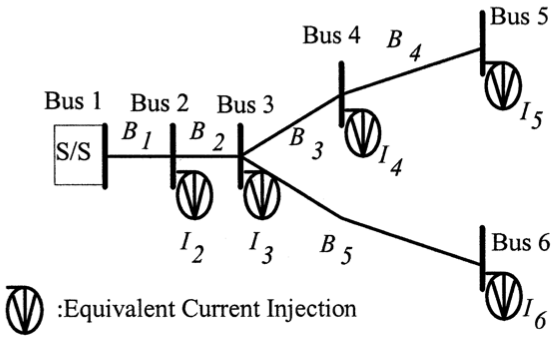
In the famous paper [teng2003direct] on direct formulation of power flow calculation for power distribution networks, there is a simplification in converting nodal current injections to line current flows. In short, current loss along lines is ignored. I am wondering if the error introduced by this simplification is significant, which is not discussed in detail in the paper.

We can focus on radial distribution feeders only. There is an example in the paper:

The relationship between the bus current injections and branch currents can be expressed as: $$ \left[\begin{array}{l} B_{1} \\ B_{2} \\ B_{3} \\ B_{4} \\ B_{5} \end{array}\right]=\left[\begin{array}{lllll} 1 & 1 & 1 & 1 & 1 \\ 0 & 1 & 1 & 1 & 1 \\ 0 & 0 & 1 & 1 & 0 \\ 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 1 \end{array}\right]\left[\begin{array}{l} I_{2} \\ I_{3} \\ I_{4} \\ I_{5} \\ I_{6} \end{array}\right] $$ where \$B\$ represents complex current flows along lines and \$I\$ represents complex current injections at buses.

The relationship should be $$ \left[\begin{array}{l} B_{1} \\ B_{2} \\ B_{3} \\ B_{4} \\ B_{5} \end{array}\right]=\left[\begin{array}{lllll} 1 & 1 & 1 & 1 & 1 \\ 0 & 1 & 1 & 1 & 1 \\ 0 & 0 & 1 & 1 & 0 \\ 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 1 \end{array}\right]\left[\begin{array}{l} I_{2} + I_{\text{loss}, 1} \\ I_{3} + I_{\text{loss}, 2} \\ I_{4} + I_{\text{loss}, 3} \\ I_{5} + I_{\text{loss}, 4} \\ I_{6} + I_{\text{loss}, 5} \end{array}\right] $$ where \$I_{\text{loss}, k}\$ represents the current loss along line \$k\$.

---

In a related paper [zad2018new], the problem is mentioned when converting nodal power injections to line power flows. $$ \left[\begin{array}{l} P_{12} \\ P_{23} \\ P_{34} \\ P_{45} \\ P_{36} \end{array}\right]=\left[\begin{array}{lllll} 1 & 1 & 1 & 1 & 1 \\ 0 & 1 & 1 & 1 & 1 \\ 0 & 0 & 1 & 1 & 0 \\ 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 1 \end{array}\right]\left[\begin{array}{l} P_{2} + P_{\text{loss}, 1} \\ P_{3} + P_{\text{loss}, 2} \\ P_{4} + P_{\text{loss}, 3} \\ P_{5} + P_{\text{loss}, 4} \\ P_{6} + P_{\text{loss}, 5} \end{array}\right] $$

---

• [teng2003direct]: Teng, J. H. (2003). A direct approach for distribution system load flow solutions. IEEE Transactions on power delivery, 18(3), 882-887.
• [zad2018new]: Zad, B. B., Lobry, J., & Vallée, F. (2018). A New Voltage Sensitivity Analysis Method for Medium-Voltage Distribution Systems Incorporating Power Losses Impact. Electric Power Components and Systems, 46(14-15), 1540-1553.

Answer 1

I find this question asked by me 2 months ago is very stupid. There is no concept called current loss in such networks or DC networks. Only power is lost along cables, and voltage drops. So the formulation in [teng2003direct] is correct. That formulation can be found in other paper as well, like [conti2006voltage].

---

• [conti2006voltage] Conti, S., Greco, A. M., & Raiti, S. (2006, December). Voltage sensitivity analysis in mv distribution networks. In Proceedings of the 6th WSEAS/IASME International Conference on Electric Power Systems, High Voltages, Electric Machines, Tenerife, Spain (pp. 16-18).