Ideal opamp closed loop problem

Question

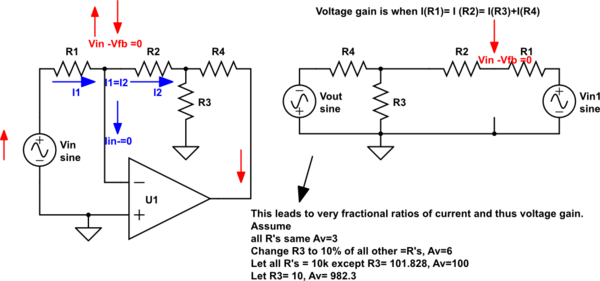
The inverting circuit with the T network in the feedback is redrawn in Fig. P2.30 in a way that emphasizes the observation that R2 and R3 in effect are in parallel (because the ideal op amp forces a virtual ground at the inverting input terminal). Use this observation to derive an expression for the gain by first finding and For the latter use the voltage-divider rule applied to R4 and (R2 || R3)

Above is the question I cant understand why i cant an Req=R2||R3+R4 in feedback circuit. But using a volatge divider considering R2||R3 and R4 to calculate Vx is valid.

Answer 1

Hints: -

why i cant an Req=R2||R3+R4 in feedback circuit.

Your formula finds the Thevenin equivalent resistance but looking into the R4 resistor from the op-amp output node. That will be of some use in your derivation.

You also need to recognize that the Thevenin equivalent voltage at the junction of R3 and R4 is a fraction of that at the op-amp's output. So, find Vx in terms of Vout and then recognize that Vx feeds via R2 creating the negative feedback.

Or regard the op-amp output as being Vx and derive the op-amp gain to Vx (R2 as feedback) then, when you have that formula, convert it to the real op-amp output using the reverse of the attenuation made by R4 and R3||R2.

Then you are good to go.

Answer 2

Absolute gains shown by example (*-1)

^{simulate this circuit – Schematic created using CircuitLab}