How does radio work? There's certainly no loop between the transmitter and the receiver, and yet they communicate, somehow.
How does a circuit with a capacitor, which has two plates separated by an insulator, work? If you start pulling the two plates apart, at what point does it stop being a capacitor and start being an open circuit?
How does the current know how much to flow, before having seen the resistor?
In high school we were all taught that electricity only flows in a loop.
Rigorously, what does this mean? What "electricity" are we talking about, anyway? This statement doesn't mean much because it doesn't even really define what it's talking about.
Kirchoff's circuit laws are more rigorous. Specifically, Kirchoff's current law states:
The algebraic sum of currents in a network of conductors meeting at a point is zero.
If you can't draw a loop, it's because you have a node somewhere that connects to only one thing. And the only way for one thing to sum to zero is for that one thing to be zero. So, current can't flow through an open circuit.
But here's the thing: a schematic is a mathematical model: it is not a physical electrical device. The lines are not real wires and the capacitors are not real capacitors. Rather, the things on a schematic represent idealized components which obey simple mathematical rules which may or may not be sufficiently representative of the real world.
The paradox arises when you take a wire connected to a TDR and model it as a line on a schematic connected to a TDR and nothing else:

simulate this circuit – Schematic created using CircuitLab
According to the rules of schematic evaluation, no current can flow. Yet the TDR does indeed work.
The paradox is resolved when the schematic is updated to more completely model the real world. Every section of a real wire is a small inductor. Likewise, every section of that wire also has some capacitance to all the things around it, like Earth. So a more complete schematic looks something like this:
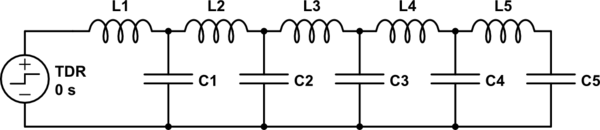
simulate this circuit
Now there is a loop in which current can flow, and the paradox is resolved. Physically, the circuit is still just a TDR and a wire, but now the schematic more accurately models the real-world behavior of a real wire.
Continuing down this path you might want to model the resistance of the wire and other things. Eventually you will arrive at a transmission line model.
The moral of the story is that schematics are only models, and the model must include all the real behaviors of the physical device it describes. If you're just powering a small light with a wire, neither the current nor the voltage change rapidly, and so the inductance of the wire and its capacitance to the surrounding environment or other things in the circuit aren't really interesting, so you can leave them out of the schematic. Assuming low current for the gauge of the wire, you can also neglect the resistance of the wire. But a TDR is explicitly designed to send a very fast step down the wire, and now that inductance and capacitance is relevant, so omitting it from the schematic means the model doesn't sufficiently capture the real behavior of the device.


