Assume that \$V_i = 1\$. It doesn't change the essence of the problem since a linear time invariant system is assumed. Just multiply the final answer with \$V_i\$.
The step response is approximated as \$k\cdot t\$ in equation (6.2), where \$k\$ is some constant (\$\frac{g_m}{C_0}\$). So the corresponding (approximate) impulse response is \$k\ u(t)\$, the unit step function. So, you need to convolve the output of previous stage with this impulse response to get output of next stage.
Input of first stage is unit step. Output of first stage is \$k\ t\$ equation (6.2).
So, the input of second stage is \$k\ t\$. When convolved with \$k u(t)\$ we get the output as
$$
\int_0^t k \cdot u(\tau) \times k\cdot (t-\tau)\ d\tau = k^2 t^2 - k^2 t^2/2 = k^2 t^2/2
$$
This is the input of the third stage. When convolved again with \$k u(t)\$ we get the output as (checked via Wolfram Alpha)
$$
\int_0^t k \cdot u(\tau) \times k^2 \cdot (t-\tau)^2/2\ d\tau
= k^3 t^3/(2\cdot 3)
$$
Continuing, the nth stage output is \$k^n t^n / n!\$.
This is what is given in (6.4).
note
If you are familiar with Laplace transform, the difficult to perform convolutions simplify to multiplication. Try that route if convolutions are difficult for you.

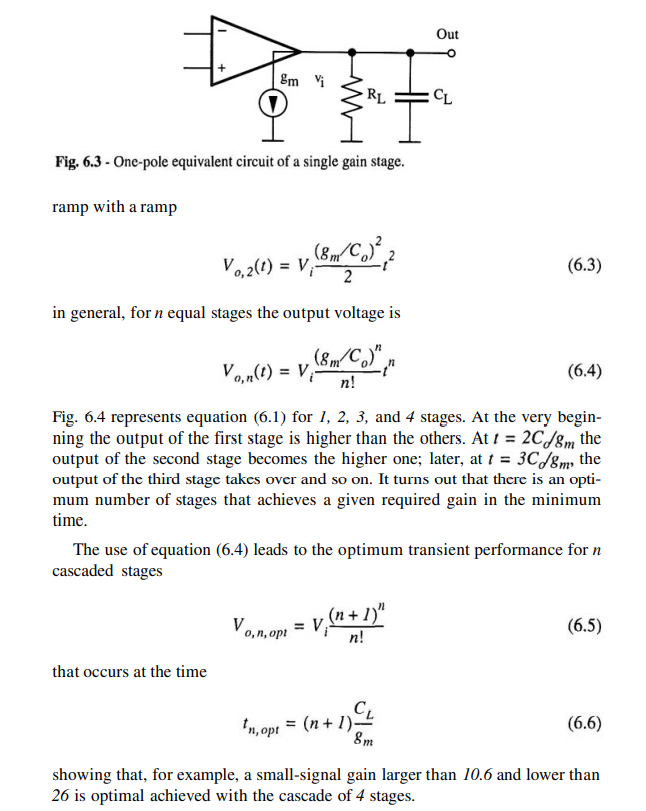 I wanted to make an comparator with cascade of amplifiers of equal gain, output impedance etc. We know that we can approximate it as single pole system , thus a resistor and a capacitor in the output will do the approximation. I was able to calculate the gain for first stage i.e $$-g_m * R_o * \frac{t }{R_o C_o} $$
I wanted to make an comparator with cascade of amplifiers of equal gain, output impedance etc. We know that we can approximate it as single pole system , thus a resistor and a capacitor in the output will do the approximation. I was able to calculate the gain for first stage i.e $$-g_m * R_o * \frac{t }{R_o C_o} $$
speedanddelay. Usually, we talk about phase delay for a given frequency. For that, you need to express the gain as a transfer function which depends on frequency and not tie it to the time variablet. By the way how did you calculate the expression for the gain given in the question? Does it take into account the effect of the input impedance of the next stage? I see only \$R_o\$ in the expression. Does that include the effect of loading due to the next stage? \$\endgroup\$convolution; but it is not mentioned in the snippet. The previous page of the snippet probably has that part. Did you understand the derivation of the impulse response and the calculation of amplifier response that would have been given in the previous page? \$\endgroup\$