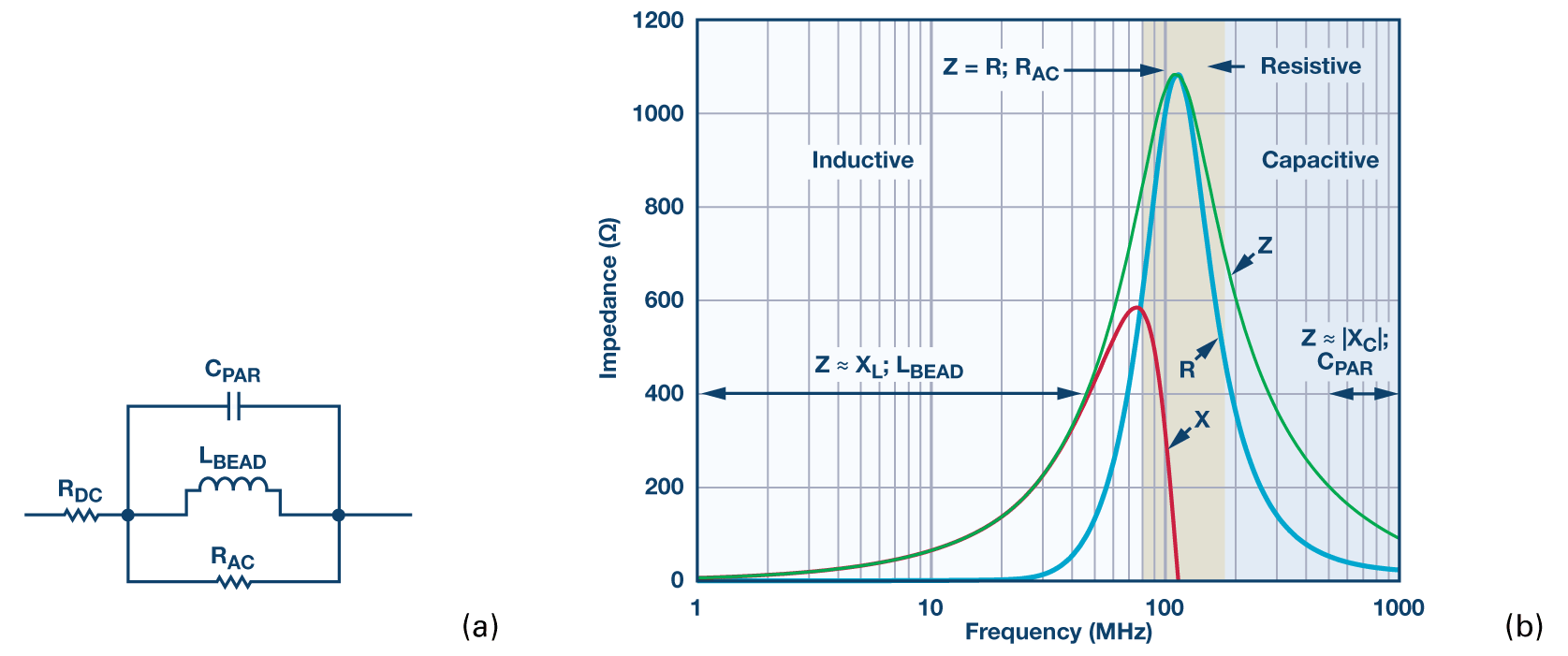
Sorry, if this is obvious or stupid, but what is the point of ferrite beads with more than \$Z\approx100\space\Omega\$ of impedance? I mainly see their application as serial filters in either power lines (impedance usually \$Z\approx10\space\Omega\$ or even lower) or signal lines (impedance \$Z\approx50\space\Omega\$).
Therefore, when placing beads with large resistance of several \$100\space\Omega\$ in such situations, won't they essentially work like a rather usual high Q inductor that will nicely resonate with the surrounding capacitances? From what I understand, it is exactly the low parallel resistance that damps resonances...
I am talking about "typical" (to me) beads chips in e.g. 0603 footprints, which have several 100 mA to A rating and give their impedance value at 100 MHz typically, while the impedance could peak at a few 100 MHz.