6
\$\begingroup\$

I have been simulating a ferrite bead on LTSPice, however, I so far have been using the LTSpice database to select the Ferrite bead, I now want to use a ferrite that is not on the database.

However, how do I get the parameters needed to simulate the ferrite bead?

For example for the following ferrite bead on the database we have the following values:

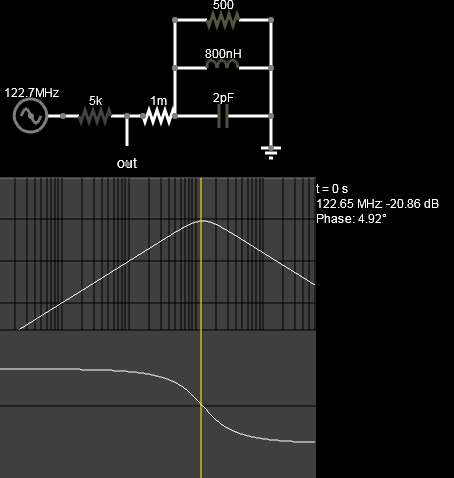
enter image description here

But all that is given in the datasheet found,here is:

enter image description here

How do I calculate those parameters using that graph or do I need more information?

\$\endgroup\$

2 Answers 2

Reset to default
6
\$\begingroup\$

From an answer to an earlier question you asked is this circuit: -

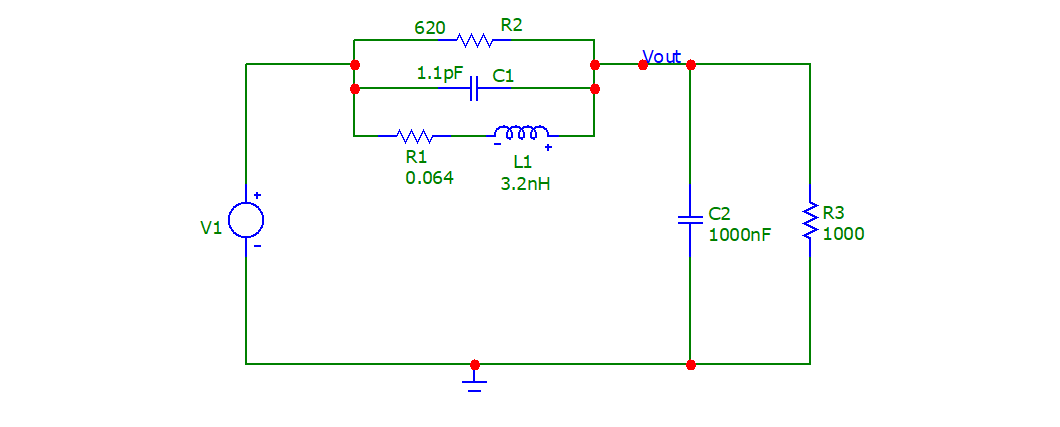
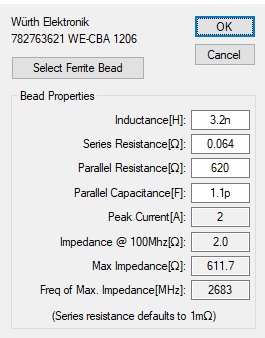
enter image description here

The values for R1, R2, L1 and C1 were taken from this ferrite bead table: -

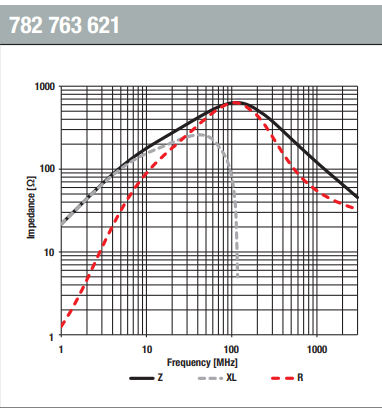
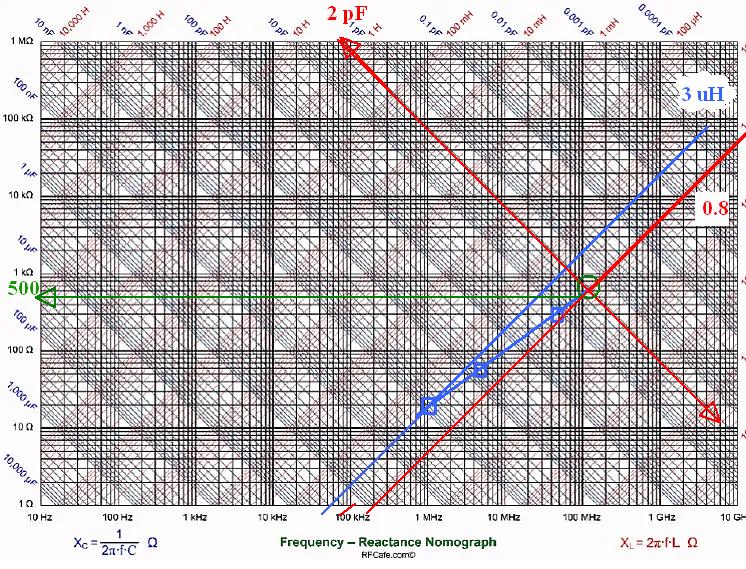
enter image description here

But, if all you have is the graph shown below, you can calculate L1 using XL (20 Ω) at 1 MHz and, you'll get \$L_1 = \frac{X_L}{2\pi 10^6}\$ = 3.18 μH.

However, in your original question, you had stated (or rather LTSpice appeared to state) that L1 was 3.2 nH and that now seems a flawed value. Clearly the ferrite beads are the same part number and also, the maximum impedance does not occur at 2.683 GHz but occurs at 100 MHz.

So, something is awry with the values in that question you posed earlier. Clearly, maximum impedance occurs at around 100 MHz (not 2.683 GHz) and, I trust this graph rather than LTSpice: -

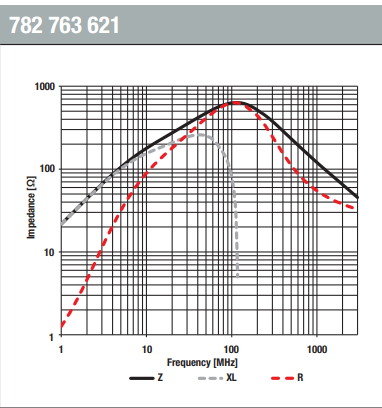
enter image description here

So, accepting that the inductance L1 is 3.2 μH, the capacitance can be found using the maximum impedance frequency of 100 MHz (the graph above shows that) and the value of L1: -

$$\text{100 MHz} = \dfrac{1}{2\pi\sqrt{L_1C}}$$

I get C1 = 0.792 pF.

For R2, the clue is in the part number's last three digits 621 and in the table on page 12 of the data sheet. R1 is stated as being 0.1 Ω.

\$\endgroup\$
6
  • \$\begingroup\$ Is there a reason why L1 is not calculated at say 20MHz where XL=200, which makes L1=1.59uH @ 20MHz? Is it always calculated at 1MHz \$\endgroup\$
    – JoeyB
    Commented Apr 19, 2022 at 20:38
  • 1
    \$\begingroup\$ Yes, because as you rise higher and higher in frequency and closer to the resonant frequency, the effect of the parasitic capacitor (0.792 pF) degrades the pure inductive reactance to a mixture of both capacitive and inductive reactances. \$\endgroup\$
    – Andy aka
    Commented Apr 19, 2022 at 20:40
  • \$\begingroup\$ I see, so at the crossover point at XL=R we have a resistive part and after the max Z=max R @ 100MHz the bead behaves has a cap with lower impedance has the freq. goes greater than 100MHz, so the bead then starts allowing high frequencies through since the cap elememt is in "series". Is all of this correct? \$\endgroup\$
    – JoeyB
    Commented Apr 19, 2022 at 20:48
  • 1
    \$\begingroup\$ Yes, @JoeyB that is correct; to the left of the resonant peak the device is net-inductive and, to the right (higher frequencies), the device is net-capacitive. \$\endgroup\$
    – Andy aka
    Commented Apr 19, 2022 at 20:49
  • 1
    \$\begingroup\$ Yes, those pesky caps turn net-inductive above their resonance!! \$\endgroup\$
    – Andy aka
    Commented Apr 19, 2022 at 21:18
5
\$\begingroup\$

Normally you can model any bead with any combination of RLC parts.

Since this follows the standard RLC parallel resonance at; fo ~ 110 Mhz and Zo ~ 500 ohms.

Fast & easy method to get close

Step 1 . Locate the Z and f at the peak and read the LC parts at fo, Zo intersection get L, C

Step 2. Chose Rp = 500 Ohms

Step 3 Plot using an approximate current source sweep using a source impedance 10x higher or -20 dB

R = 500 , L =0.8 uH , C = 2pF

enter image description here

enter image description here

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.