implementing dot convention

Question

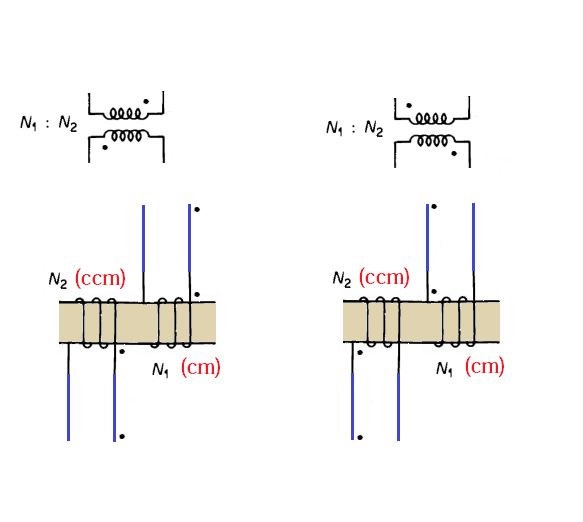
in the following topology, a boost inductor with secondary winding is operated in which the windings are in opposite directions. Implementing this structure, in reality, made me confused. Based on my efforts to find a solution, I have come to the conclusion that CM or CCM windings are not structurally different and depends on which end we consider as the beginning of the winding. In the same coil, if we look at it from one end, the direction of winding is CCM, while if we look at the same coil from the other end, the direction of winding is CM. Therefore, by changing the terminals, we can make the coil CM or CCM, which is shown in the following figures. In the first figure, both windings are wound CM, but we have changed the terminals of the second winding. Is it the same as in the second figure, which is one CM and one CCM without changing the polarities?

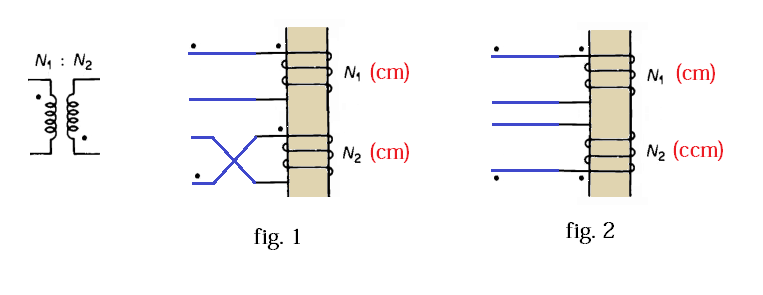
Question 2: The choice of dotted ends is a contract and we can consider them on other ends as shown below. in better words, there is not any difference between the ends of winding to connect in a practical circuit. Is this sentence correct?

Answer 1

As far as I know, you are correct.

In power transformers, eventually, one of the ends may have less insulation than the other and, in this case, it is important to observe the correct connection. There are also some considerations in three-phase transformers, which lead to angular displacement. However, your statement is entirely valid for the cases you cited.