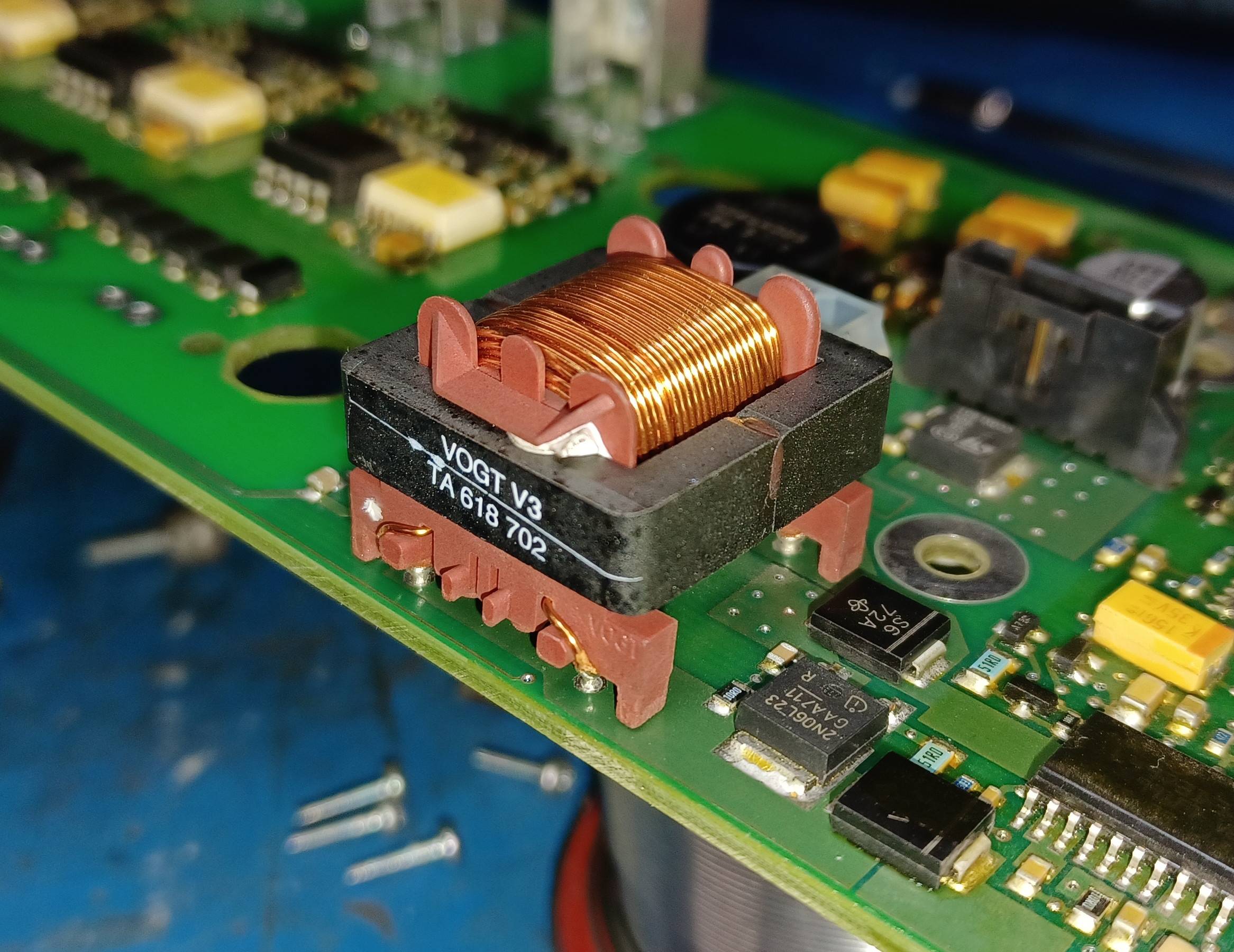
Impossible to tell by inspection. Given some more context (pinout, surrounding circuit), or measurements (windings and pinout, inductance, estimated number of turns), the internal gap can be estimated.
Inductivity is given by: \$A_L = \frac{\mu_0 A_e}{l_c / \mu_r + l_g}\$. It looks like it may be a 1:1 transformer with same size and length wire, and, so nice of them not to wrap it in tape so it can be seen clearly as-is -- an estimate of turns should be feasible here. Basically count layers and turns per layer, multiply, then halve for the two windings and that should be close. We can assume at first it's generic power ferrite, \$\mu_r \approx 2000\$, and measure \$A_e\$ and \$l_c\$ from the outer dimensions of the core. (The center peg will be twice the width of the side legs, and the E core is the same height all around.) \$A_e\$ is height times width of the center peg. For path length \$l_c\$, take the perimeter around one loop (center peg to one leg and back), along the midline. Roughly, the outer dimensions of the core, minus center peg width, divided by two. And \$\mu_0\$ is the inductivity of free space, about 1.257 nH/mm. (Doing everything in mm gets the result in nH/t^2, or you can use whatever system of units you like, given suitable conversion. Google Calculator handles these expressions just fine.) Finally, \$A_L\$ is in units of inductance per squared turn; multiply by the number of turns squared, to get total measured winding inductance. Or, in this case, to go backwards and solve for \$l_g\$.