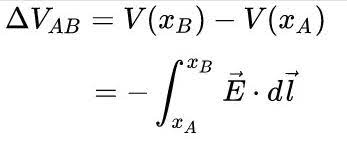How do transmission lines carry both high voltage and low current

Question

I've read several other previous posts about this, and none of them seem to address the exact conceptual doubt I'm having, so I'll take a shot at posting this...

Question: How can we have high voltage as well as low current?

Situation: a hydroelectric generating dam generates 13.8kV and steps it up to 138kV, so winding ratio is 1:10. Neglecting losses, by conservation of energy we have that

P_in = P_out.

Or

I_inV_in = I_outV_out, I_out = (V_in/V_out)I_in => 0.1I_in = I_out

--> So we have 10% the current but at the same power, which is great because this reduces I^2*R losses and we can therefore use cables rated at lower ampacity, saving $, materials, and energy. I get all of this, and I think I understand how transformers and generators work, but...

Here's where I get confused:

Now my understanding of voltage comes from the path-integral definition, which states that it's the work necessary to move a charge, per unit charge, along a path against a conservative electric field (very analogous to gravitational potential energy):

(1)

And my microscopic understanding of charge carrier motion comes from the relation of drift velocity to the electric field

(2) V_d = uE,

where V_d is the average drift velocity, u is the electron mobility, and E is the electric field.

And finally my microscopic understanding of current is a function of drift velocity

(3) I = neAV_d,

(where n is electron density, e electron charge, A cross sectional area of conductor).

My confusion:

A) If we increase voltage V, we must increase electric field E, since V is proportional to E (1).

B) If we increase E, then we must increase V_d, since V_d is proportional to E (2).

C) If we increase V_d, we must increase I, since I is proportional to V_d (3).

Therefore: if we increase V, since A)--implies-->C), we must increase I.

==> So -- how can we have high voltage as well as low current, or how can we raise the voltage without raising the current?

(I must be missing something, because I know we do it, and I get how transformers exploit Faraday's law to transform voltages. Perhaps is it the load at the end of the line that has a large impedance, and this is what limits the current on the secondary? All of the residences, businesses, etc, that are drawing power? Is this one of the reason why supply must match demand?)

Answer 1

You may use a step-up transformer to increase the voltage between the wires which carry the voltage to the city (where the voltage is converted again down for the electricity consumers). But you are not going to increase the voltage between the ends of one wire, you prefer that the voltage drop in a wire reduces. Consequence: No growth of longitudal electric field in a wire. That electric field and the mobility of electrons are related to current in a wire.

The transversal electric field between the wires is related to the transfer voltage and it shouldn't used to calculate the current in a wire by applying it only to the electrons in the wire.

Answer 2

You are overlooking where voltage is increased. The voltage is increased between power line and the ground. Your charges move perpendicular to that which takes no energy at all concerning the electric field pointing to the ground.

The voltage moving the charges is in direction of the power lines, as a voltage loss. This loss is proportional to the current flow.

Answer 3

Because a transformer steps the voltage up or down, and to have the same power on both sides of the ideal transformer, the current will be stepped down or up respectively to keep power constant.

From that, it follows that a transformer with some load impedance taking some power at some voltage and current at secondary, will be taking some other amount of current at some other amount of voltage at the primary, so it will have different impedance even if power is constant.

Since voltages and currents get converted by the ratio of turns, the impedance gets converted by the ratio of turns squared.